6
$\begingroup$

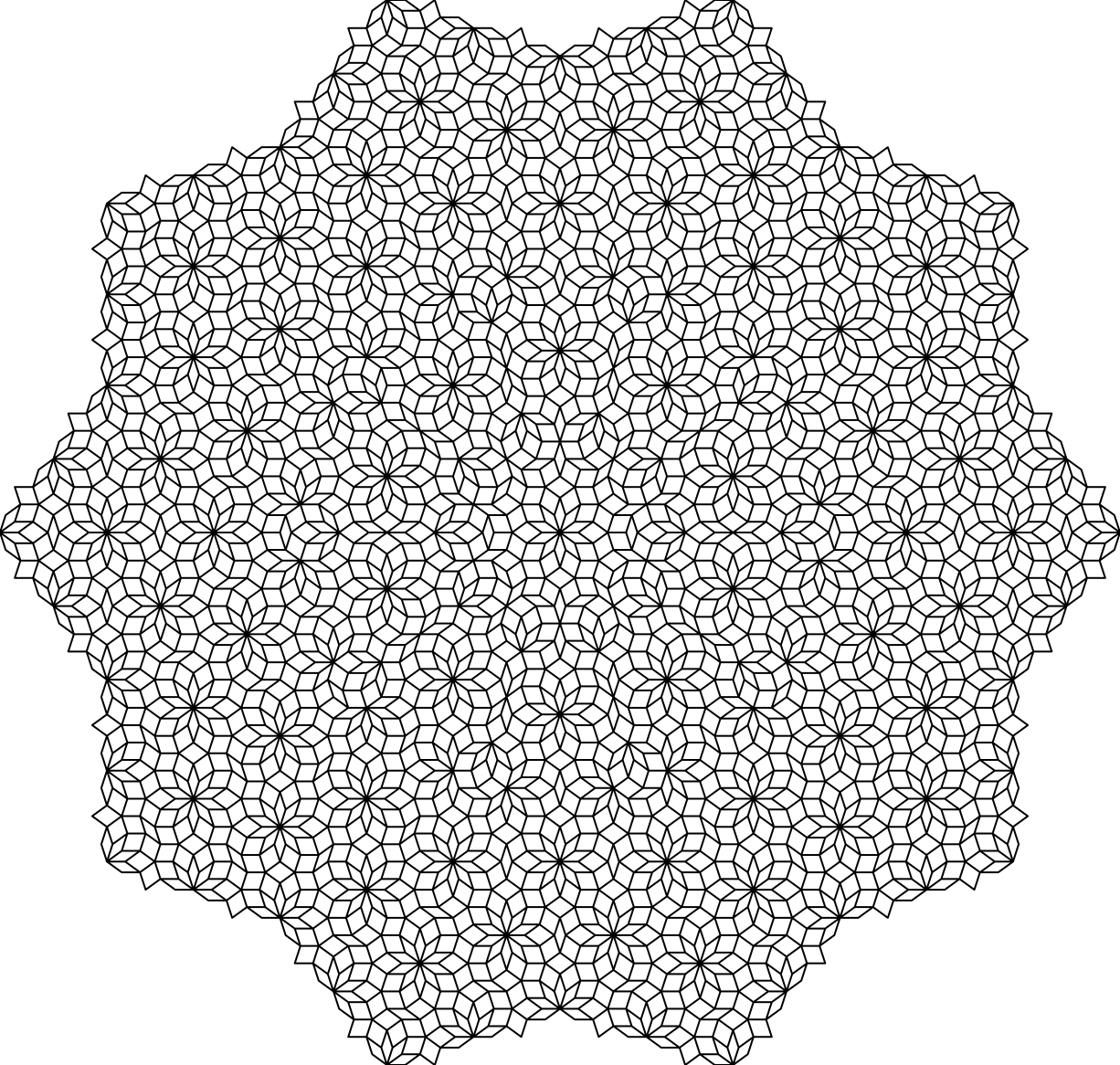
Consider tilings of the plane made out of rhombi of side 1 and either angles $\pi/10$ and $2\pi/5$ or angles $\pi/5$ and $3\pi/10$. If we give a certain orientation to the edges and respect that orientation in our tiling, then, as is well known, we must have a Penrose tiling.

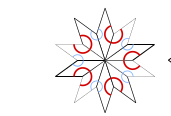
It is not hard to show that a Penrose tiling cannot contain this sort of pattern:

enter image description here

(picture taken from https://arxiv.org/pdf/2211.08239.pdf)

But what happens if we remove the orientation? Can we have that pattern (without the red arcs that show orientation, obviously) and extend it to a tiling of the entire plane by our two kinds of rhombi, preferrably keeping 10th-fold symmetry?

Obviously, such a tiling would have to be non-periodic, since a lattice cannot have 10th-fold symmetry.

(The fact a Spanish tiling company sells such a tiling (https://todobarro.com/suelo/coleccion-penrose/) makes me suspect one might exist, though something tells me they may not have actually extended it to all of $\mathbb{R}^2$.

enter image description here

EDIT: in order to avoid simple solutions, let us forbid tilings where only one of the two kinds of tiles is used outside a finite neighborhood.

Improve this question
$\endgroup$
2
  • $\begingroup$ That also raises the question: if you remove the orientations from an actual Penrose tiling (with 5-fold symmetry), can you complete the intersection of the tiling with an arbitrarily large ball in a "boring" way (periodic, or one-tile, etc.)? $\endgroup$
    – H A Helfgott
    Commented Dec 20, 2022 at 13:19
  • $\begingroup$ Perhaps you will also find the following question interesting: mathoverflow.net/questions/447233/… $\endgroup$
    – Max Lonysa Muller
    Commented Nov 29 at 21:43

5 Answers 5

Reset to default
8
$\begingroup$

If you impose no other restrictions, something like this works just fine:

enter image description here

For a slightly more complicated variant incorporating both tile shapes:

enter image description here

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ Right, I thought I had forbidden the first option with my wording ("... by our two kinds of rhombi"). The second option also involves only one of the two tiles outside a bounded neighborhood. What happens if one also forbids that? $\endgroup$
    – H A Helfgott
    Commented Dec 19, 2022 at 11:04
  • 1
    $\begingroup$ @HAHelfgott: In the second tiling, you can see "inward-pointing arrows" where two lines of thin rhombs at opposite orientations meet along edges. For every point in each such arrow, one can insert a fat rhomb in between them, leaving the rest of the arrow pointing outwards (and creating a new inward-pointing arrow). By repeatedly doing this to whichever inward-facing arrows remain, we can get infinitely many fat rhombs. (I can include a diagram later if this description isn't clear.) $\endgroup$
    – RavenclawPrefect
    Commented Dec 19, 2022 at 19:03
  • $\begingroup$ Ah yes. (a) Does this procedure give all possible tilings with 10-fold symmetry and a star in the middle? (b) Note that the tilings produced by this procedure have the property that, for every radius R, one can take the part of our tiling inside a ball of radius R around the origin, and complete it as a tiling of the plane in a "boring" way ( = using only one kind of tile). Are there tilings with 10th fold symmetry that do not have this property. (This is analogous to the strong version of the definition of "aperiodic".) $\endgroup$
    – H A Helfgott
    Commented Dec 19, 2022 at 19:35
  • 1
    $\begingroup$ @HAHelfgott: It's not clear to me that genuine Penrose tilings even have this property? E.g. here's an incomplete diagram demonstrating that one common chunk of a Penrose tiling can be extended only using thin rhombs. $\endgroup$
    – RavenclawPrefect
    Commented Dec 20, 2022 at 18:57
  • $\begingroup$ That's a really small neighborhood! But yes, let me look... $\endgroup$
    – H A Helfgott
    Commented Dec 21, 2022 at 9:45
6
$\begingroup$

We can start with a floret that looks like this:

floret with 10-fold symmetry

then follow the following substitution rules (repeatedly) :

substitution rules

this yields a tiling, part of which is shown below.

patch

Some things to note about this tiling. It is non-periodic, has 10-fold rotational symmetry, and has infinitely many centers of rotation (of order 10), each of which is the center of a patch of indefinite area.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Hi Andrew, I urge you to include at minimum a few words explaining what you did. Link-only answers on MathOverflow are fragile, and also it's better for posterity to keep the answers actually on the site. Thanks! $\endgroup$
    – David Roberts ♦
    Commented Nov 29 at 23:31
  • 1
    $\begingroup$ Thanks @DavidRoberts that is useful feed-back, I edited the post accordingly! $\endgroup$
    – Andrew Bayly
    Commented Nov 30 at 0:03
  • $\begingroup$ thank you, and welcome to MO! $\endgroup$
    – David Roberts ♦
    Commented Nov 30 at 1:23
3
$\begingroup$

There are tiles with this star in the center. It also has inflation as you see in the image:

(image)

These are not Penrose tilings since they do not follow the same rules in its creation.

Improve this answer
$\endgroup$
2
$\begingroup$

I think pages 364/365 in Kari, Lutfalla; Substitution Discrete Plane Tilings with 2n-Fold Rotational Symmetry; Discrete & Computational Geometry (2023) 69:349–398 https://doi.org/10.1007/s00454-022-00390-z give an example and some theory.

Improve this answer
$\endgroup$
1
$\begingroup$

The division of a pentagon into triangular pieces described here can be used to generate a tenfold quasilattice. At each node generated by any iteration additional edges are rendered in subsequent iterations until a complete set of ten edges at 36° angles is made. Then each mode approaches tenfold symmetry in the limit of infinite divisions.

enter image description here

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .