3
$\begingroup$

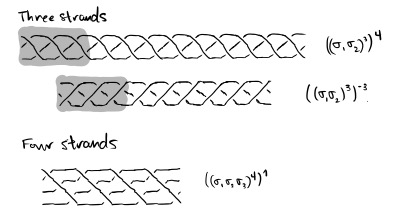
In order to simplify Kirby calculus proofs, one can use a box notation which indicates a number of full twists up to sign. In the scenario of two strands with twist boxes, it is straightforward to draw the corresponding picture.

Let's assume that we have three strands with -3 and 4 full twists. I am very confused when I have tried to draw these diagrams.

How can we draw them explicitly? What is the algorithm? Any reference for explicit drawings would be useful.

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ If you let $A$ denote the braid where the left-most strand goes over all the others, and terminates at the right endpoint, with all the other strands being linearly embedded and "shifting left" by one slot, then the "full twist" braid is $A^n$ where $n$ is the number of strands. $A$ is also expressible in terms of the generators of the braid group, $A = \sigma_{n-1} \sigma_{n-2} \cdots \sigma_2 \sigma_1$. Birman's "Braid Groups" text has some good pictures of these -- they are central in the braid group. $\endgroup$
    – Ryan Budney
    Commented Dec 19, 2022 at 23:28
  • 1
    $\begingroup$ I endorse Ryan's answer, but keep in mind that if you are keeping track of framings using ribbon notation (rather than integers labeling an unframed diagram), then you need to add a kink with appropriate sign to each strand in the $A^n$ braid. $\endgroup$
    – Kevin Walker
    Commented Dec 20, 2022 at 0:30
  • 1
    $\begingroup$ You may look at Figure 5 in the classical paper of Fenn and Rourke on Kirby calculus: core.ac.uk/reader/82333300 $\endgroup$
    – Oğuz Şavk
    Commented Dec 22, 2022 at 20:48

1 Answer 1

Reset to default
3
+100
$\begingroup$

A trio of braids: the first is four full twists on three strands, the second is minus three full twists on three strands, and the third is one full twist on four strands

Here are the relevant pictures, plus one more (on four strands) for good measure. I learned this material from the well-illustrated book Knots, links, braids and 3-manifolds by Prasolov and Sossinsky. Chapters III and VI discuss the braid group and surgery diagrams, respectively.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Does the gray part correspond to one full twist? $\endgroup$
    – Terry Black
    Commented Dec 20, 2022 at 20:48
  • 1
    $\begingroup$ Yes, and the 4-strand version is one full twist, as well (without any grey). $\endgroup$
    – Ryan Budney
    Commented Dec 20, 2022 at 20:57

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .