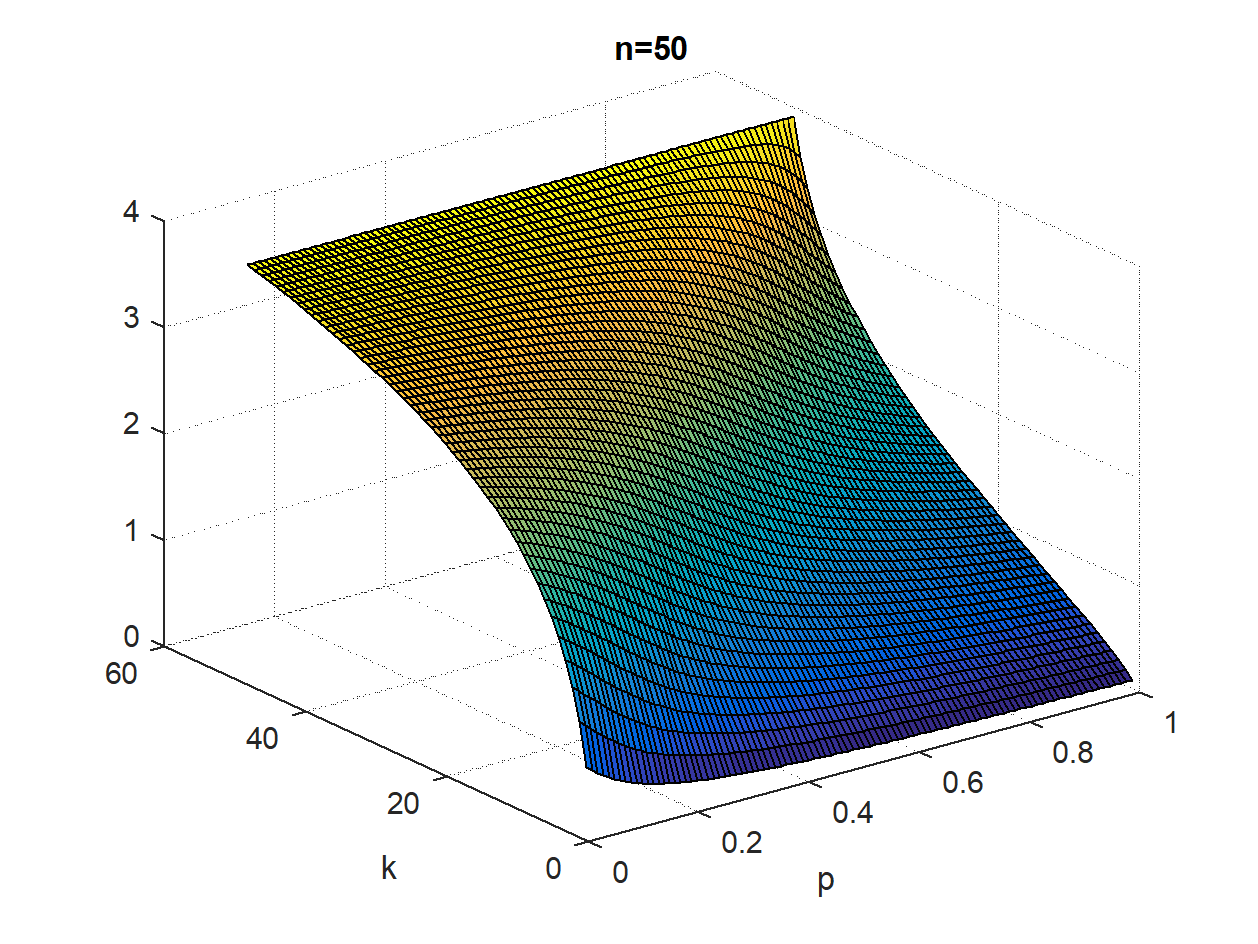
Let $X$ follow a binomial distribution with $n$ trials and success probability $p$, and let $0\leq k\leq n$. Are there any natural approximations or bounds for the ratio $$\frac{\boldsymbol{E}\log\binom{X}{(X-k)^+}}{\boldsymbol{E}(X-k)^{+}}$$ where $(t)^{+}=\max\lbrace0,t\rbrace $? I attached a surface plot for $n=50$.
It is obvious that when $p\to 0$, the quantity looks like $\log k$, and when $p\to 1$, it looks like the quantity is more or less linear in $k$, and we're interpolating between those two.