11
$\begingroup$

Let $\phi(x,y,z)$ be an alternating trilinear form on a space $V$ over a field $K$.

Let $u \in \mathbb{P}(V)$ be a projective point over $V$, then we say that the rank of $u$ is equal to the rank of the alternating bilinear form $\phi(u,.,.)$.

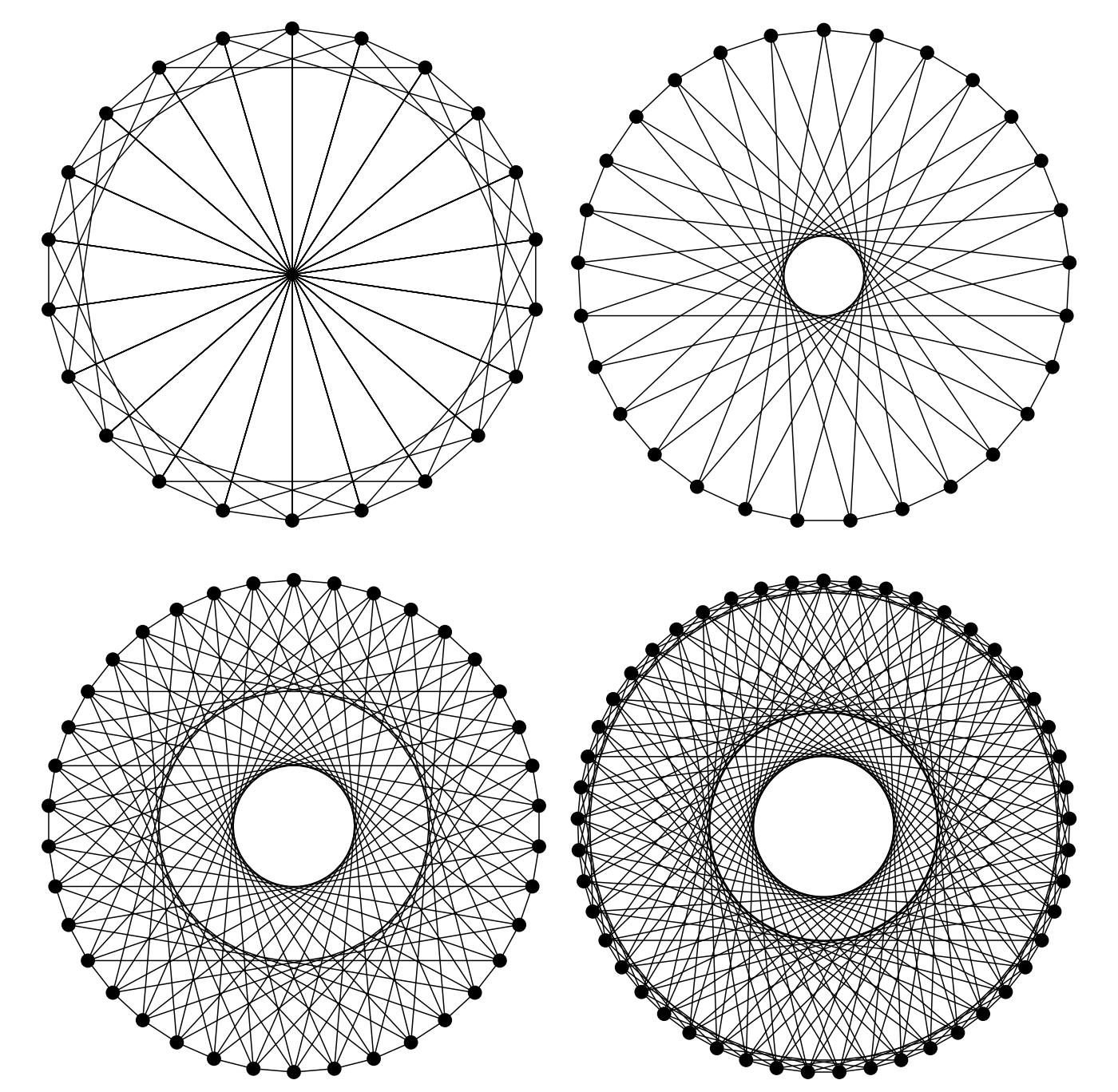
To the form $\phi$ we can associate a graph $G_\phi$, whose the vertices are the projective points in $\mathbb{P}(V)$, and two vertices $u,v$ share an edge if the linear form $\phi(u,v,.)$ is identically zero.

For Section 7 of my work, I computed the restriction of these graphs to points of rank 4 for uniformly random $9$-dimensional alternating trilinear forms over finite fields of prime order $p$, and I stumbled on the following observation, which I cannot explain:

It seems that with high probability (the probability seems to go to 1 as $p$ grows), the graphs have Dihedral symmetry $D_{N}$, where $N$ is the number of points of rank 4. See, e.g., pictures below over GF(5).

Where does this come form? A guess would be that the automorphisms of the graph come from automorphisms of the trilinear form, but this is not the case. Usually, random forms $\phi$ have only a few (e.g. 2,3,4, or 6) automorphisms, but the graphs have much more automorphisms.

In fact, the automorphism group of the graph is not (always) a subgroup of $GL(n,K)$, because the rotation of the second picture below has order 29, but 29 does not divide $|GL(9,5)| = 2^{25}×3^5×5^{36}×7×11×13^2×19×31^3×71×313×829×19531$.

It is not so difficult to prove that the average (for random $\phi$) number of nodes in the graph is $q^2 + O(q)$, and the average number of edges is $q^3/2 + O(q^2)$ (Theorem 1 in my work). But the structure of the graph is still very mysterious to me. The pictures say that there is a free and transitive group action of the cyclic group of order $N$ on the $N$ points of rank $4$, but I have no idea what this group is and how it acts.

enter image description here

Improve this question
$\endgroup$
4
  • 4
    $\begingroup$ In case the link you supplied rots sometime in the next decade, your paper at the link is titled Graph-theorethic Algorithms for the Alternating Trilinear Form Equivalence problem $\endgroup$
    – David Roberts ♦
    Commented Nov 8, 2022 at 13:43
  • 1
    $\begingroup$ What is so special about rank 4 and dimension 9? Do you have similarly unexpected symmetries for other ranks/dimensions? $\endgroup$
    – Marco Golla
    Commented Nov 9, 2022 at 16:56
  • 1
    $\begingroup$ @MarcoGolla For $n \leq 8$ there is a huge number of automorphisms, just because $|GL(n,q)|\approx q^{n^2} > |ATF(n,q)| = q^{\binom{n}{3}}$, so these behave differently. For $n \geq 10$ I don't see the symmetries (although I didn't do a lot of experiments, because the graphs become very large and hard to compute). For $n=9$ I focus on rank 4, because that is whp the smallest rank that appears. $\endgroup$
    – Ward Beullens
    Commented Nov 9, 2022 at 19:17
  • 1
    $\begingroup$ So it appears that rank 4 and dimension 9 is special somehow. $\endgroup$
    – Ward Beullens
    Commented Nov 9, 2022 at 19:25

1 Answer 1

Reset to default
8
$\begingroup$

The structure of the graph comes from a group structure on the set of rank-4 points!

Apparently you can associate an Abelian surface to alternating trilinear forms in dimension 9, e.g., see The geometry of the Coble cubic and orbital degeneracy loci by Vladimiro Benedetti, Laurent Manivel, Fabio Tanturri.

The points of the abelian surface are the rank-4 vectors of the alternating trilinear form, and after choosing an identity element, theorem 6.1 of Benedetti et al. allows you to compute the group law.

The rotations of the graphs I plotted are translations $X \mapsto X+Y$, and the reflections are just inversion $X \mapsto -X$. You get one inversion per choice of identity element.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .