Proof of a theorem in degenerate Monge Ampère equation by Vincent Guedj and Ahmed Zeriahi

Question

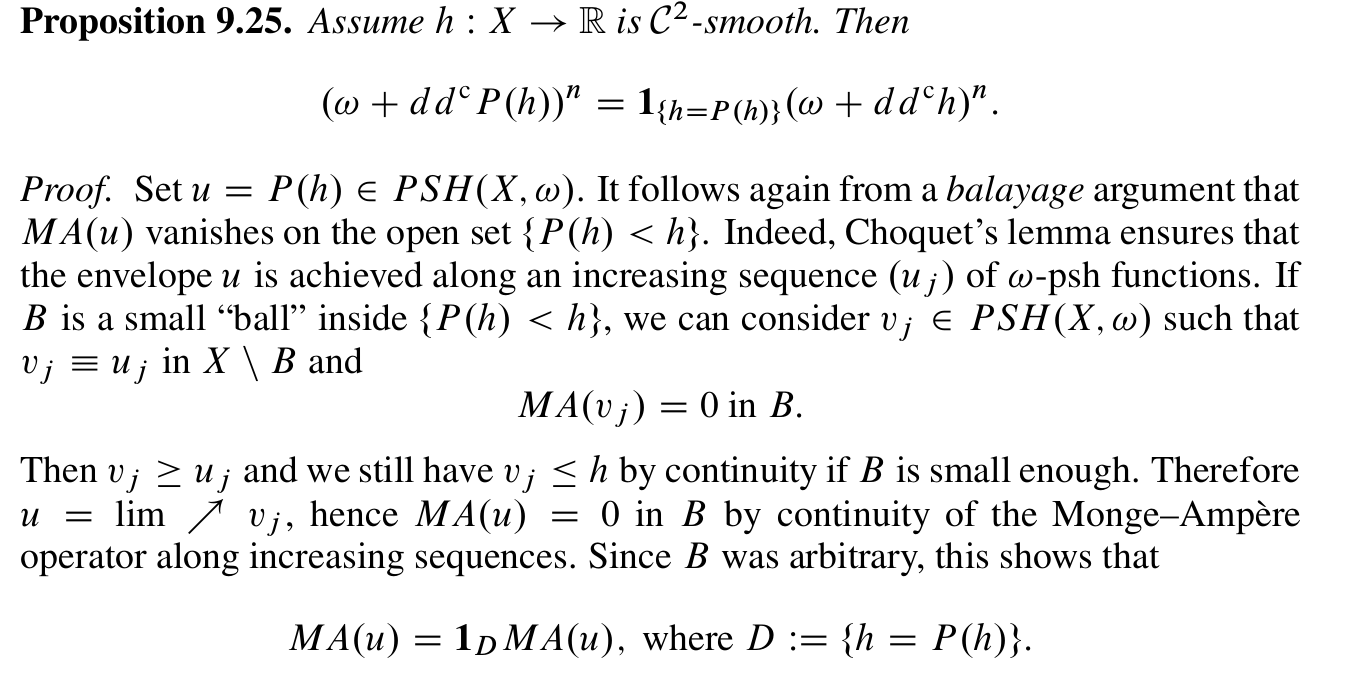
$\DeclareMathOperator\PSH{PSH}$This question is about Proposition 9.25 page 252 from the book "Degenerate Complex Monge-Ampère Equations" by Vincent Guedj and Ahmed Zeriahi (see picture below).

Let $X$ be a compact complex Kähler manifold and $\omega$ a closed $(1, 1)$ form which is semi positive and smooth. One can define the set $\PSH(X, \omega)$ as the set of $\phi \in L^{1}$ such that the current $\omega + dd^{c} \phi$ is positive. If $\phi$ is locally bounded one defines, for every positive close current $T$ of bidegree $(n-1, n-1)$, the positive current $(\omega + dd^{c} \phi) \wedge T := \omega \wedge T + dd^{c}(\phi T)$. Hence, the positive distribution $MA(\phi) := (\omega + dd^{c} \phi)^{n}$ which is a positive measure is well defined.

Fact : if $B$ is a small open in $X$, for all $\phi \in \PSH(X, \omega)$, their exists a unique $\psi \in \PSH(X, \omega)$ such that $\psi \ge \phi$ in $X$ and such that $MA(\psi) = 0$ in $B$ and $\phi = \psi $ in $X - B$.

---

Now, if $h$ is $C^{2}$ smooth on $x$ with real values, then one can defined the envelope $P(H)(x) := \sup ( \phi(x) | \phi \in \PSH(X, \omega), ~ \phi \leq h )$.

I send you as an image the proof I don't understand : when they say Then $v_{j} \ge u_{j}$ and we still have $v_{j} \leq h$ by continuity if B is small enough.

How can they choose $B$ small enough uniformly in $j$?

By unicity of the previous fact, it's easy done for a fixed $j$. But I don't see how to do it uniformly.

Answer 1

On $B$, there is $\delta>0$ such that $P(h)\le h-\delta$. Next, pick a point $x\in B$ and choose a potential $\rho$ for $\omega$ on $B$ such that $\rho(x)=0$. By shrinking $B$, you can assume that $\sup_B |\rho| \le \frac \delta 4$ and $\mathrm{osc}_B h \le \frac \delta 4$.

Since $\rho+v_j$ is psh on $B$, one has $$\sup_B(v_j+\rho)= \sup_{\partial B} (v_j+\rho)=\sup_{\partial B}(u_j+\rho) \le \sup_B P(h)+\frac \delta 4.$$

Given $y\in B$, one has $$v_j(y) \le \sup_B(v_j+\rho)+\frac \delta 4 \le \sup_B P(h) + \frac \delta 2 \le \sup_B h -\frac \delta 2 \le h(y).$$