I'm having some trouble following an argument in Moritz Groth's paper on Derivators, pointed derivators and stable derivators. More precisely, I'm currently stuck on the rotation axiom of the triangulated structure for stable derivators and I'm hopeful that someone familiar with the paper can help out.
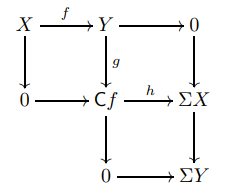
Let $\mathbb{D}$ be a stable derivator and let $X \xrightarrow{f} Y \xrightarrow{g} Z \xrightarrow{h} \Sigma X$ be a distinguished triangle in $\mathbb{D}(e)$. We wish to prove that the rotation $Y \xrightarrow{g} Z \xrightarrow{h} \Sigma X \xrightarrow{-\Sigma f} \Sigma Y$ is distinguished.
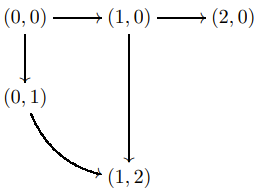
To do so, Moritz considers $J \subseteq [2] \times [2]$ depicted as
Moreover, $i:[1] \to J$ classifies $(0,0) \to (1,0)$ and $j:J \hookrightarrow ([2] \times [2]) - \{(0,2) \} = K$. One checks that the underlying diagram of $j_! i_*(f)$ looks as follows:
At this point, I cannot see why the underlying map $\Sigma X \to \Sigma Y$ has to be $\Sigma f$ and how one formally rotates this rectangle on the right.
Groth is writing something about a unique natural transformation $(d^0 \times d^1) \Rightarrow (d^1 \times d^2)$ of functors $\ulcorner \to K$ and that $(d^0 \times d^1) : \ulcorner \to K$ differs from the usual inclusion by an automorphism. But I can't see how these help.
(Sorry, this is towards the end of the paper, so I'm really not sure how to make my question more transparent for more readers without it being infinitely long. But I hope that someone who has read Groth's paper sees this question.)
This is a repost from MSE: MSE/4555945.