Coincidence of sequential weak star closure and weak star closure

Question

Given a real Banach space $X$ and its continuous dual $X^*$. Recall from [Definition 3.106 & Corollary 3.110, Jean-Paul Penot, Calculus without derivatives] that

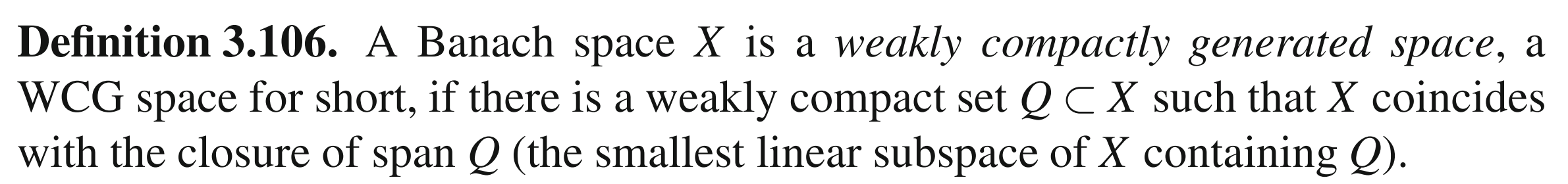
Here are my questions:

1. Are there some well-known, broader classes $\mathcal{V}$ of spaces compared to those WCG in which the following property still holds:

${\bf (A)}$: For any bounded subset $B$ of $X^*$, with $X\in\mathcal{V}$, the weak$^*$ sequential closure and weak$^*$ closure of $B$ coincide.

2. Can we derive some sufficient conditions on $B$, in addition to its boundedness, such that ${\bf (A)}$ still holds in arbitrary Banach spaces?

Thank you!

Answer 1

For question (1), weakly Lindelöf determined spaces (WLD spaces, for short) does it. A WLD space is characterised by the dual ball, modulo the weak-star topology, being a Corson compactum (i.e., for some appropriate set $\Gamma$, it is homeomorphic to a compact subspace of the space $\Sigma(\Gamma)$ of elements from $\mathbb{R}^\Gamma$, modulo pointwise/product topology, with only countable non-zero indices). In this case, the dual ball is weak-star angelic, i.e. the weak-star closure of any subset of the dual ball coincides with the weak-star sequential closure. Every WCG space is a WLD space. (It is perhaps worth remarking that the dual ball being weak-star sequential—i.e. any weak-star sequentially closed subset being weak-star closed—does not necessarily imply that it is weak-star angelic; for this, and other references there regarding WLD-ness, see https://arxiv.org/pdf/1612.05948.pdf, also see https://cmj.math.cas.cz/full/59/3/cmj59_3_5.pdf)

For the above reason, for question (2), a sufficient condition is that the weak-star closure of $B$ be a Corson compactum.