Sum of reciprocals of A086005

Question

Does the sum of reciprocals of terms of A086005 converge?

Answer 1

The sum does converge. We will follow the suggestion of Professor Elkies stated in the comments. Note that the number $k$ is ''sandwiched'' between two semiprimes iff $k=2p$ and $2p\pm 1$ are semiprimes. Let us estimate the number of such primes $p\leq X$, where $X\to +\infty$. Here we will use only two conditions: primality of $p$ and semiprimality of $2p+1$. Using semiprimality of $2p-1$ one can arrive at sharper estimates. Since $2p+1$ is semiprime, it has a form $2p+1=qr$, where $q$ and $r$ are primes. We will assume that $q\leq r$. In particular, $q\leq \sqrt{2X+1}$. For a given prime $q$, let us estimate the number of prime $p\leq X$ with $2p+1=qr$, where $r$ is also prime.

Clearly, $r$ is odd. Let $r=2s+1$, then $s\leq X/q$ and $p=qs+(q-1)/2$. If $s\geq X^{1/6}$, then both $2s+1$ and $qs+(q-1)/2$ must be coprime to all primes $\ell\leq X^{1/6}$. For $\ell\neq 2,q$ this means that $s$ should avoid two residue classes $\mod \ell$. For $\ell=2$ or $q$ we get a single prohibited residue class instead. By standard sieve methods (see, for instance, Fundamental lemma of sieve theory), we see that the number $A(q)$ of such $s\leq X/q$ is $$ A(q)\ll X^{1/6}+\frac{X}{q}\prod_{\ell\neq 2,q, \ell\leq X^{1/6}}\left(1-\frac{2}{\ell}\right)\left(1-\frac{1}{q}\right)\left(1-\frac12\right)\ll \frac{X}{q\ln^2 X}+X^{1/6} $$ Summing over $q\leq \sqrt{2X+1}$, we see that the total number of $s$ (and hence of $p\leq X$) is bounded by $$ \sum_{q\leq \sqrt{2X+1}}\left(\frac{X}{q\ln^2 X}+X^{1/6}\right)\ll \frac{X\ln\ln X}{\ln^2 X}. $$ This is certainly enough, since this gives for all $N$ $$ \sum_{\substack{k\in \text{A086006}\\ 2^N<k\leq 2^{N+1}}}1/k\ll \frac{\ln N}{N^2} $$ and we get a convergent series. Adding the semiprimality of $2p-1$, one can get $\frac{X(\ln\ln X)^2}{\ln^3 X}$ instead. This order of growth is probably accurate.

Answer 2

This sequence, say $(a_1,a_2,\dots)$, is the increasing enumeration of the set (say $S$) of natural numbers $k$ such that each of the numbers $k-1,k,k+1$ is semiprime. So, the problem seems to be of the same flavor as the twin prime conjecture.

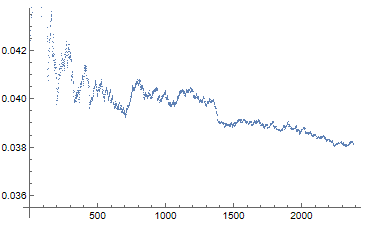
Here is the graph $\{(n,\frac{n\,\ln n\,\ln\ln n}{a_n})\colon n=2,3,\dots,\ a_n\le10^6\}$:

This graph suggests that $a_n\asymp n\,\ln n\,\ln\ln n$ and hence $\sum_n\frac1{a_n}=\infty$. At least, the graph suggests that $a_n\ge c n\,\ln n\,\ln\ln n$ for some real $c>0$ and all natural $n$, and then the set $S$, of all semiprimes $k$ "sandwiched" between semiprimes $k-1$ and $k+1$, would be infinite.

So, it seems unclear if this problem is easier than the twin prime conjecture.