2
$\begingroup$

I was recently reading a book about the implicit function theorem (IFT): The implicit function theorem: history, theory, and applications, and before that I learned that Peano's existence theorem can be used to prove the IFT (the existence of implicit functions); in this book, an idea of using the IFT to prove Peano's existence theorem (PET) is given: idea of using the implicit function theorem to prove Peano's existence theorem

Theorem 3.4.10 mentioned in it is as follows (with its proof): Theorem 3.4.10 with proof

where $d_{2}G(x,y)$ is defined as d_2

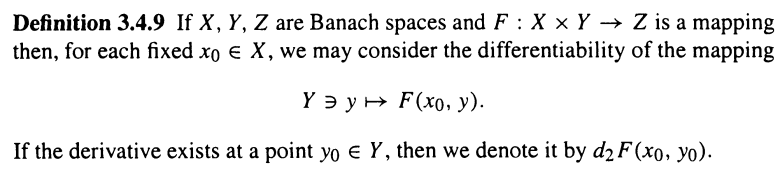
Apart from this book, I haven't found other papers or books describing IFT to prove PET (maybe this view is of little value?), so I have to consult you here.

My questions are as follows: Is this proof of PET using IFT correct? If correct does this mean that IFT is equivalent to PET?

Improve this question
$\endgroup$
7
  • 3
    $\begingroup$ This is perhaps a bit pedantic and certainly unhelpful as far as the actual question goes, but I don't like this use of the word "equivalent". What exactly does it mean to claim that two true statements are (or are not) equivalent to each other? (For example, is the fundamental theorem of calculus "equivalent" to Fermat's last theorem? In any formal sense, the answer would seem to have to be yes since one holds if and only if the other does.) $\endgroup$
    – Christian Remling
    Commented Jul 21, 2022 at 14:19
  • $\begingroup$ Perhaps a naive question, but I have to ask: what's the other view on the existence theorem for ODEs? The only time I saw a proof of this was when I took a course on ODEs as an undergrad, and my vague recollection is that it was proven using the implicit function theorem. $\endgroup$
    – Anurag Sahay
    Commented Jul 21, 2022 at 15:44
  • 1
    $\begingroup$ By the way, @ChristianRemling: perhaps the discussion in the following answer might help alleviate your irritation? mathoverflow.net/a/284231/37327 Certainly this is not what people "mean" when they say two theorems are equivalent to one another, but I think of this as being morally what they are saying [the same way as a theorem in a non-set-theory paper "is" morally its formalization in, say, ZFC]. $\endgroup$
    – Anurag Sahay
    Commented Jul 21, 2022 at 15:52
  • $\begingroup$ Rudin was rather fond of listing equivalent statements in his books. I can think of four equivalent (and very useful) ways to characterize any Appell polynomial sequence. Using the umbral notation with $(a.)^k = a_k$, given $A_0=1,$ 1} $D_x A_n(x) = nA_{n-1}(x)$, 2) e.g.f. is $e^{a.t}e^{xt}$, 3) $A_n(x) = (a.+x)^n$, and 4) the raising op, defined by $RA_n(x) = A_{n+1}(x)$, is $R = D_{t = D_x} \ln[e^{a.t}e^{xt}]$. Take any one as a definition, the others can be treated as theorems. 1) doesn't allow for direct construction, 2) to 4) do given the moments $a_k$. Each has its use. $\endgroup$
    – Tom Copeland
    Commented Jul 21, 2022 at 16:21
  • $\begingroup$ When I think of an inverse pair of formal e.g.f.s $f(x)$ and $g(x)$, I keep in mind equivalent perspectives: 1) an autonomous o.d.e., 2) integral curves constructed from a vector field of tangents, 3} reflection through $y=x$, 4) iterated Graves-Lie infinitesimal generators, 5] refined Euler characteristic polynomials of associahedra, 6) marked phylogenetic trees, 6} punctured Riemann surfaces, 7} moduli spaces of certain marked curves, 8) weighted noncrossing partitions, 9) $f(x) =x h(f(x))$, 10) . . . , and, of course, the IFT, but never Fermat's LT. $\endgroup$
    – Tom Copeland
    Commented Jul 21, 2022 at 16:55

0

Reset to default

You must log in to answer this question.