Smooth approximation for non differentiable function

Question

Let $f(t) = \min(\frac{1}{\lvert t\rvert}, 1)$. I would like to find a smooth approximating function $g$ such that $f(t) \leq g(t)$ for all real $t$. Is there a nice function $g$ out there? Any suggestion appreciated!

Answer 1

$\newcommand\de\delta$If the function $f$ were convex, then a convolution of $f$ with (say) an even positive mollifier would do. However, $f$ is not convex.

Yet, it would be quite easy to construct just some smooth approximating function $g$ such that $f\le g$. The challenge here, as I see it, is to make sure that the approximating function $g$ is nice (as requested by the OP) and preferably explicitly given.

Such an explicit construction is as follows:

For $\de\in(0,1/2)$ and all real $t$, let $$ g_\de(t):=\frac12\,\Big[\frac1{\sqrt{t^2+\de}}+\de+1-h_\de\Big(\frac1{\sqrt{t^2+\de}}+\de-1\Big)\Big], $$ where $$h_\de(y):=\frac{y^2}{\sqrt{y^2+\de}}.$$

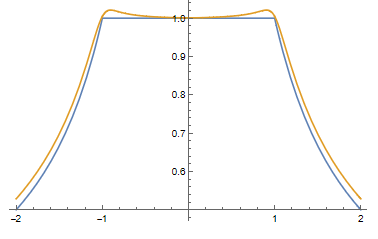
Then $g_\de$ is a smooth function, $g_\de\ge f$, and $g_\de\to f$ uniformly on $\mathbb R$ as $\de\downarrow0$ -- as desired.

(Details on this are provided at the end of this answer.)

---

Here are the graphs $\{(t,f(t))\colon|t|<2\}$ (blue) and $\{(t,g_{1/50}(t))\colon|t|<2\}$ (golden):

---

Details: First here, we understand that the definition $f(t):=\min(\frac1{|t|},1)$, which can work only for $t\ne0$, is complemented by $f(0):=1$, by continuity.

Next, take indeed any $\de\in(0,1/2)$. Then, for real $t$ with $t^2\ge1-\de$, \begin{equation*} 0\le\frac1{|t|}-\frac1{\sqrt{t^2+\de}}=\frac\de{|t|\sqrt{t^2+\de}\,(|t|+\sqrt{t^2+\de})} \\ \le\frac\de{\sqrt{1-\de}(\sqrt{1-\de}+1)}<\de. \tag{1}\label{1} \end{equation*} So, for real $t$, \begin{equation*} f(t)\le f_\de(t):=\min(1,x_\de(t)),\quad\text{where}\quad x_\de(t):=\frac1{\sqrt{t^2+\de}}+\de. \tag{2}\label{2} \end{equation*} Moreover, $f_\de(t)-f(t)\le x_\de(t)-\frac1{|t|}<\de$ for all real $t$. So, \begin{equation} 0\le f_\de-f<\de. \tag{2a}\label{2a} \end{equation}

Next, for all real $y$, \begin{equation*} h_\de(y)\le|y| \tag{3}\label{3} \end{equation*} and \begin{equation*} |y|-h_\de(y)=\frac{|y|\de}{\sqrt{y^2+\de}\,(|y|+\sqrt{y^2+\de})} \le\frac{|y|\de}{y^2+\de}<\sqrt\de, \end{equation*} so that \begin{equation*} |y|-h_\de(y)\to0 \tag{4}\label{4} \end{equation*} uniformly in real $y$ (as $\de\downarrow0$).

Further, for all real $x$, \begin{equation*} \min(1,x)=\frac{x+1-|x-1|}2\le\frac{x+1-h_\de(x-1)}2, \tag{5}\label{5} \end{equation*} by \eqref{3}. By \eqref{2} and \eqref{5}, for real $t$, \begin{equation*} f(t)\le f_\de(t)\le\frac{x_\de(t)+1-h_\de(x_\de(t)-1)}2=g_\de(t) \tag{6}\label{6} \end{equation*} and, in view of \eqref{4}, \begin{equation*} g_\de(t)-f_\de(t)\to0 \tag{7}\label{7} \end{equation*} uniformly in real $t$.

So, by \eqref{2a} and \eqref{7}, \begin{equation*} g_\de(t)\to f(t) \tag{8}\label{8} \end{equation*} uniformly in real $t$ and, by \eqref{6}, $g_\de\ge f$.

Finally, that $g_\de$ is smooth is obvious. $\quad\Box$

Answer 2

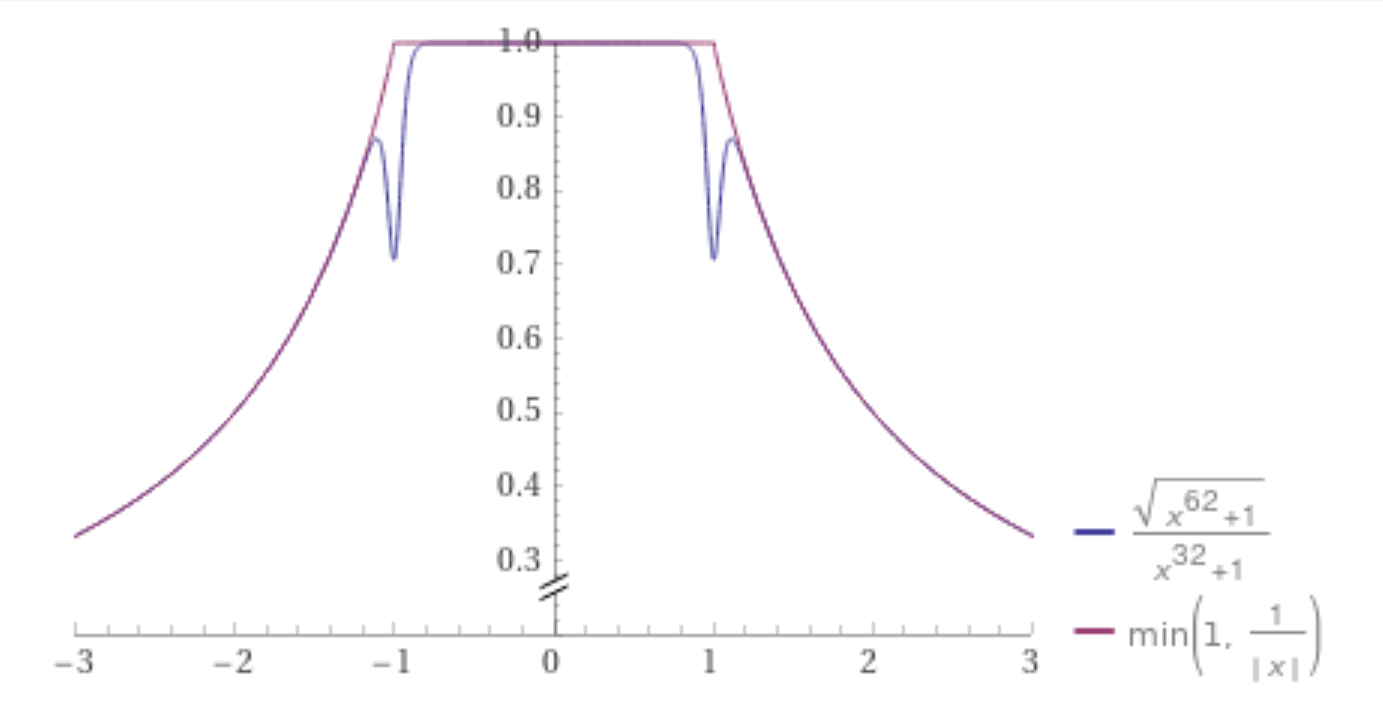
$\frac{\sqrt{1+(x^{2k+1})^2}}{1+x^{2k}}$ has an arbitrarily small range with large error:

The reason for the large deviation lies in the points of extremal curvature of $x^n$;
better solutions would require functions with monotone curvature.

Taking the monotone curvature argument into account leads to: $\sqrt{\frac{1+(x^{2k+1})^2}{1+(x^{2k+2})^2}}$