0
$\begingroup$

I want to plot the two surfaces which are defined in $ \mathbb{ R }^3 \ni ( x, y, z ) $ via the equations $ 0 = y^2 - x*(x^2 + 1) $ and $ 0 = z^2 - y*(y^2 + 1) $, respectively. Moreover, I want also to highlight the intersection curve of these surfaces.

Any tool is ok for me, but I know that sage has some functions for plotting implicit functions in 3d: The commands

var('x, y, z') 
s1 = implicit_plot3d(0 == y^2 - x*(x^2 + 1), (x,-3,3), (y,-3,3), (z,-3,3))
s2 = implicit_plot3d(0 == z^2 - y*(y^2 + 1), (x,-3,3), (y,-3,3), (z,-3,3))
plot(s1 + s2)

plot both surfaces. But I would like to highlight the intersection curve or at least change the transparency-level of the surfaces (changing the alpha-values seems not to do anything)

A bonus would also be if there were a way to directly plot the intersection of these surfaces.

Does anybody know a tool to do this?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

Something like this?

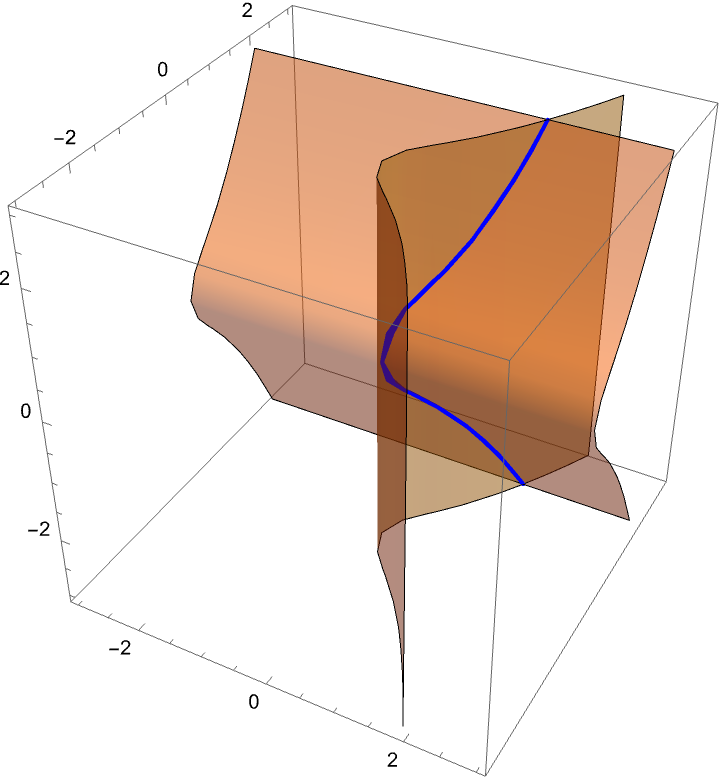Blue curve is the intersection of the two surfaces $ 0 = y^2 - x(x^2 + 1) $ and $ 0 = z^2 - y(y^2 + 1) $.

The 3D plots were generated with Mathematica, as documented here.

Improve this answer
$\endgroup$
4
  • $\begingroup$ This is exaclty what I want. Unfortunately, the free version of mathematica on wolframalpha.com seems not to do that. Or am I mistaking? $\endgroup$
    – diddy
    Commented Jul 17, 2022 at 14:50
  • $\begingroup$ I'm afraid this is beyond the capabilities of wolfram alpha; I would be happy to supply you with any image you like though, you can email me your parameters. $\endgroup$
    – Carlo Beenakker
    Commented Jul 17, 2022 at 14:51
  • $\begingroup$ Thank you for your offer. I appreciate it. But this won't be the last plot I will need. Anyway, I think we have mathematica on the computers in the university. So there will always be this solution. But a solution which runs on my home pc would be better. This is the only reason why I don't click on 'accept as an answer'. $\endgroup$
    – diddy
    Commented Jul 17, 2022 at 14:58
  • $\begingroup$ I found wolframcloud.com. There I could do the plot using mathematica for free. Thank you very much. $\endgroup$
    – diddy
    Commented Jul 17, 2022 at 18:47

Not the answer you're looking for? Browse other questions tagged .