This question is motivated by College Mathematics Journal problem 1196, proposed by Ferenc Beleznay and Daniel Hwang. My solution to this problem (pre-publication version here) uses Chebyshev polynomials to show that for every $m \in \mathbb{N}$ there is an even polynomial $P_{2m}(x) \in \mathbb{Q}[x]$ of degree $2m$ such that the graph
$$\{(x, P_{2m}(x)) : -1 \le x \le 1\}$$
is tangent to the unit circle $x^2+y^2=1$ at $2m-1$ points, and has two further points of intersection at $\pm 1$. Numerical calculations suggest that something stronger is true.
Is is true that for every $m \in \mathbb{N}$ with $m \ge 2$ there is an even polynomial $Q_{2m}(x) \in \mathbb{Q}[x]$ of degree $2m$ such that the graph $\{(x, Q_{2m}(x)) : -1 \le x \le 1\}$ is tangent to the unit circle at $2m-3$ points, and has two further triple points of intersection?
Examples. The quartic $Q_2(x) = 1 - \frac{(3x)^2}{2} + \frac{(3x)^4}{24}$ is tangent to the unit circle at $(x,y) = (0,1)$ and has triple points at $(x,y) = (\pm \frac{2\sqrt{2}}{3},-\frac{1}{3})$. The graphs below show the intersections for
\begin{align*} P_8(x) &= 1 - 25 x^2 + 104 x^4 - 144 x^6 + 64 x^8 \\ Q_8(x) &= 1 - \frac{(7 x)^2}{2} + \frac{(7 x)^4}{24} - \frac{(7 x)^6}{864} + \frac{(7 x)^8}{96768} \end{align*}
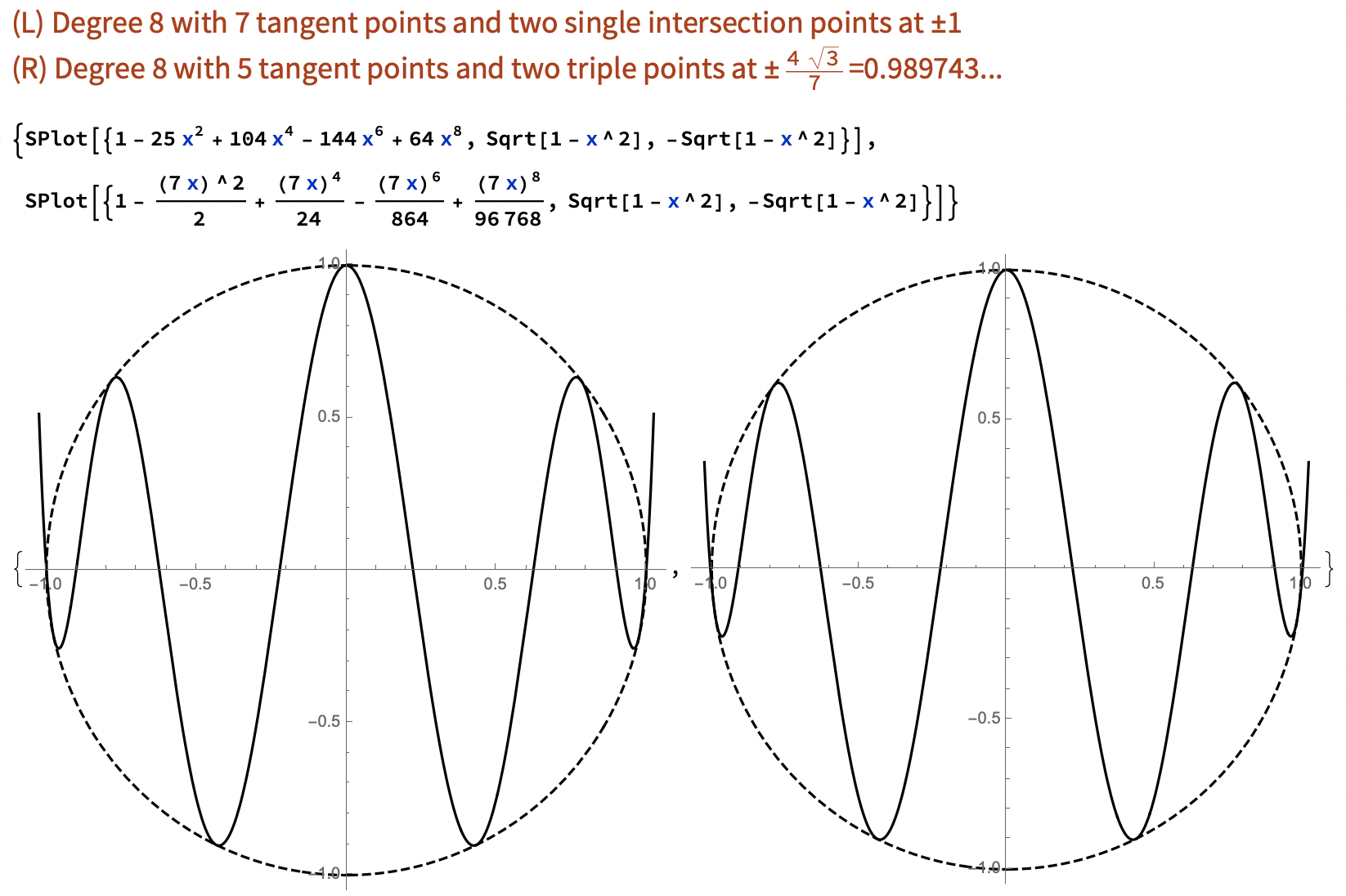
Further remarks.
- The polynomials $P_{2m}(x)$ in fact have coefficients in $\mathbb{Z}$. They meet the bound in Bezout's Theorem for the intersection multiplicity between the plane algebraic curves $y = P_{2m}(x)$ of degree $2m$ and $x^2 + y^2 = 1$ of degree $2$, since $(2m-1)\times 2 + 2 \times 1 = 4m$. If $Q_{2m}(x)$ exists then it also meets the bound, now with $(2m-3) \times 2 + 2 \times 3 = 4m$.
- I've asked for a rational polynomial $Q_{2m}(x)$ because my numerical experiments suggest this is possible, and the coefficients of the polynomials I've found seem to have some interesting aritmetic properties, see the solutions below. (Does anyone recognise the denominators?) But I have not proved the existence of $Q_{2m}(x)$ working over the real numbers.
\begin{align*} 2:\quad & 1 - \frac{(3x)^2}{2} + \frac{(3x)^4}{24} \\ 3:\quad & 1 - \frac{(5x)^2}{2} + \frac{(5x)^4}{24} - \frac{(5x)^6}{1080} \\ 4:\quad & 1 - \frac{(7x)^2}{2} + \frac{(7x)^4}{24} - \frac{(7x)^6}{864} + \frac{(7x)^8}{96768} \\ 5:\quad & 1 - \frac{(9x)^2}{2} + \frac{(9x)^4}{24} - \frac{(9x)^6}{800} + \frac{(9x)^8}{64000} - \frac{(9x)^{10}}{14400000} \\ 6:\quad & 1 - \frac{(11x)^2}{2} + \frac{(11x)^4}{24} - \frac{7(11x)^6}{5400} + \frac{(11x)^8}{54000} - \frac{(11x)^{10}}{8100000} + \frac{(11x)^{12}}{3207600000} \end{align*} and for $m=7$ and $m=8$ there are $$\begin{align*} 7: \quad & \scriptstyle 1 - \frac{(13x)^2}{2} + \frac{(13x)^4}{24} - \frac{(13x)^6}{756} + \frac{(13x)^8}{49392} - \frac{(13x)^{10}}{6223392} + \frac{(13x)^{12}}{1568294784} - \frac{(13x)^{14}}{999003777408}\\ 8: \quad & \scriptstyle 1 - \frac{(15x)^2}{2} + \frac{(15x)^4}{24} - \frac{3(15x)^6}{2240} + \frac{15(15x)^8}{702464} - \frac{11(15x)^{10}}{59006976} + \frac{(15x)^{12}}{1101463552} - \frac{(15x)^{14}}{431773712384} + \frac{(15x)^{16}}{414502763888640}.\end{align*}$$ The prime factorizations of the denominators of the coefficients of $x^{2m}$ for $2 \le m \le 8$ are
$$2^3\cdot 3, \quad 2^3\cdot 3^3\cdot 5,\quad 2^9\cdot 3^3\cdot 7,\quad 2^9\cdot 3^2\cdot 5^5, \quad 2^7\cdot 3^6\cdot 5^5\cdot 11,\quad 2^7\cdot 3^6\cdot 7^7\cdot 13, \quad 2^{25}\cdot 3\cdot 5\cdot 7^7$$
- At Fedor Petrov's suggestion I computed the coordinates of the triple points. For $m \le 8$ the triple point with positive $x$-coordinate is
$$\bigl(\frac{\sqrt{(2m+1)^2-1}}{2m+1}, \frac{(-1)^{m-1}}{2m+1}\bigr).$$
(Sign of $y$ coordinate corrected from $(-1)^m$.) There appears to be no simple closed form for the double points in my solutions.
- The original problem also required odd polynomials $P_{2m-1}$ of degree $2m-1$ with analogous tangency properties. I conjecture that the analogous question for $Q_{2m-1}$ also has a positive answer.
