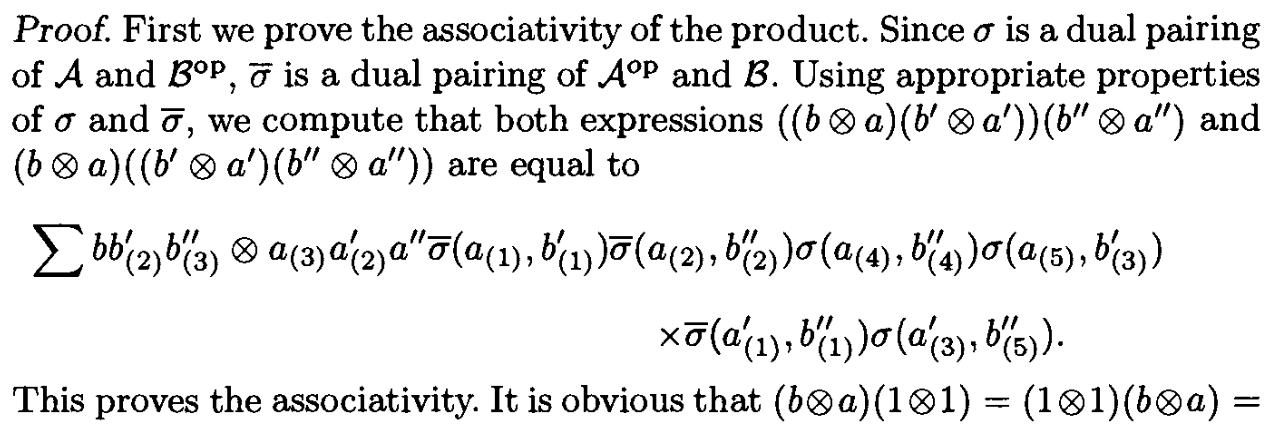0
$\begingroup$

Here is the statement about the associativity of the quantum double of bialgebras in Klimyk-Schmudgen "Quantum Groups ..." (Sec 8.2.1)

enter image description here

Can anyone help me derive the formula of on bottom of their proof? I get a different expression and don't have enough practice with experience with such computations to connect my formula to theirs. $$(b \otimes a)(b' \otimes a')(b'' \otimes a'')= (\sum bb_2'\otimes a_2a'\cdot \text{sigmas})(b'' \times a'')$$ $$=\sum bb_2'b_2''\otimes (a_2a')_2a''\otimes \text{sigmas},$$ where "sigmas" means a scalar (a product of $\sigma$'s and $\bar\sigma$'s).

enter image description here

Improve this question
$\endgroup$
4
  • $\begingroup$ Just a hint: you might find it helpful to write that product as $$(b \otimes a)(b^\prime \otimes a^\prime) := \sum b \cdot \overline{\sigma}(a_{(1)},b^\prime_{(1)})b^\prime_{(2)} \otimes a_{(2)}\sigma(a_{(3)},b^\prime_{(3)}) \cdot a^\prime,$$ which makes clear that the ‘sigmas’ encode the price of moving $b^\prime$ past $a$ (while adhering scrupulously to the Sweedler conventions). $\endgroup$
    – Branimir Ćaćić
    Commented May 4, 2022 at 13:18
  • $\begingroup$ Moreover, you really can't sweep the ‘sigmas’ under the rug without breaking the Sweedler index bookkeeping. $\endgroup$
    – Branimir Ćaćić
    Commented May 4, 2022 at 13:25
  • $\begingroup$ @BranimirĆaćić: Yes, I agree that sigmas are integral part of the formula and that your order of terms is more intuitive. But yet, my problem stands: When you expand $((b\otimes a)(b'\otimes a'))(b''\otimes a'')$ you get a substantially different formula than that in the proof. So my question is how to use the many properties of bialgebras and pairings between them to bring it to the formula in the proof. $\endgroup$
    – Adam
    Commented May 4, 2022 at 14:44
  • $\begingroup$ Hence the comment about not breaking Sweedler bookkeeping: you need to expand $(a_{(2)} a^\prime)_{(2)}$ out and exploit coassociativity. $\endgroup$
    – Branimir Ćaćić
    Commented May 4, 2022 at 15:14

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .