The following sentence in the above question seems to need to be made more clear.
Let $\mathcal{D}$ be a 1-planar drawing of a 1-planar graph $G$ that
has the minimum number of crossings, i.e, the number of crossings in
$\mathcal{D}$ is exactly the crossing number of $G$.
Does the crossing number here refer to the concept of the link below?
Definition. The crossing number ${\rm cr} (G)$ of a graph $G$ is the minimum number of crossing pairs of edges, over all drawings of $G$ in the plane.
That is, when we consider the crosssing number of a 1-planar graph $G$, all drawings of $G$ need to be considered, including its non- 1-plane drawings. Thus the crossing number of an optimal 1-planar drawing (a 1 -planar drawing with minimum crossings) of $G$ may not be equal to crossing number of $G$.
Notice that there are many 1-planar graphs whose any optimal 1-planar drawing have least a non-crossing edge, such as $K_5$, $K_6$, and any optimal 1-planar graph. Note that they have an unique 1- planar drawing up to weak equivalence. Their crossing number are $1$, $3$ and $n-2$, respectively.
See the following two papers for details.
- Suzuki Y. Re-embeddings of maximum 1-planar graphs[J]. SIAM Journal on Discrete Mathematics, 2010, 24(4): 1527-1540.
- Ouyang, Z., Huang, Y. & Dong, F. The Maximal 1-Planarity and Crossing Numbers of Graphs. Graphs and Combinatorics 37, 1333–1344 (2021).
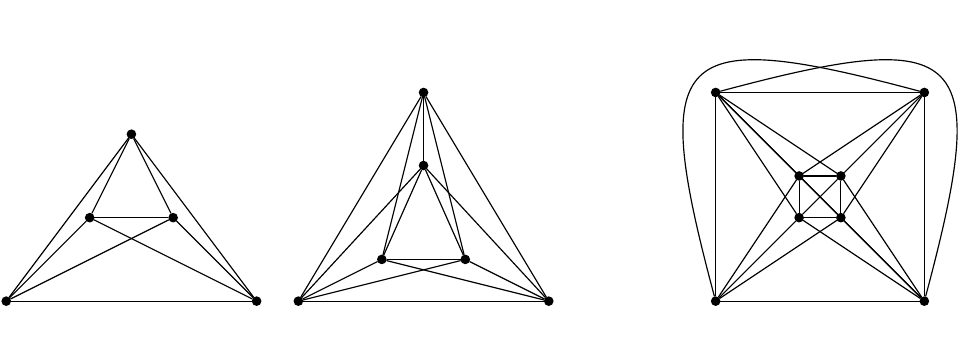 The unique 1-planar drawing of $K_5$ on the left, of $K_6$ on the middle, and of an optimal 1-planar graph with $8$ vertices.
The unique 1-planar drawing of $K_5$ on the left, of $K_6$ on the middle, and of an optimal 1-planar graph with $8$ vertices.
More generally, any maximal 1-planar graph will not be considered.
Fact.([a]) If $ab$ and $cd$ are crossing edges in $G$, then $a$, $b$, $c$, $d$ span a $K_4$ in $G$.
-[a] Barát J, Tóth G. Improvements on the density of maximal 1‐planar graphs[J]. Journal of Graph Theory, 2018, 88(1): 101-109.
The crossing point of $ab$ and $cd$ is called $x$. Thus, $ac$ is an edge in $G$ which is a non-crossing edge otherwise, we can redraw $ac$ so that it's infinitely close the line $axc$ without crossing.