6
$\begingroup$

Take a point $A$ inside a non-convex polygon $P$. Is it always possible to place a finite set of mirrors given by straight segments (not necessarily along the boundary of $P$, any position inside $P$ is allowed) such that every point of $P$ is visible from $A$? Light-rays propagate along straight lines, are reflected in the usual way on both sides of mirrors and are absorbed by the boundary of $P$.

Is there a position of mirrors which is "universal" in the sense that any pair of points inside $P$ are within view of each other?

These questions have of course obvious higher-dimensional generalizations.

Improve this question
$\endgroup$
1
  • $\begingroup$ The boundary of the polygon can of course always be supposed to be covered by mirrors (and it reflects then light-rays in the same ways as a polygonal billiard) without loss of generality. $\endgroup$
    – Roland Bacher
    Commented Oct 13, 2010 at 9:26

1 Answer 1

Reset to default
10
$\begingroup$

Permit me to repeat this example from a related MO question, based on a paper by George Tokarsky, "Polygonal Rooms Not Illuminable from Every Point" [Amer. Math. Monthly, 102:867-879 (1995)]. Here is a figure from the MathWorld article on the topic:
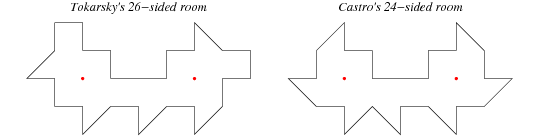
Tokarsky
These are rooms all of whose walls are mirrors but which have a pair of points $x$ and $y$ such that if a light is placed at $x$, point $y$ is dark. It is an unresolved conjecture that, at least in rational polygons (angles rational multiples of $\pi$), the number of dark points for any light position is finite. If that conjecture were true, then it seems plausible that it would be possible to place additional internal mirrors to cover those points and still illuminate the remainder of the room.

Update (13 May 2015). The "unresolved conjecture" I mention above has been settled. I found the reference in Alex Wright's 2015 paper, "From rational billiards to dynamics on moduli spaces." (arXiv abstract):

Lelièvre, Monteil and Weiss have shown that if $P$ is a rational polygon, for every $x$ there are at most finitely many $y$ not illuminated by $x$.

The reference is: Samuel Lelievre, Thierry Monteil, Barak Weiss. "Everything is illuminated." 2014. (arXiv abstract.)

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ Am I right in thinking that one can at least show that for rational polygons the set of points you can illuminate is dense? I'm not sure that even that is known if you drop the rationality hypothesis (which helps because then you can convert the problem into one about interval-exchange maps). $\endgroup$
    – gowers
    Commented Oct 13, 2010 at 13:22
  • 1
    $\begingroup$ @gowers: I feel you must be right. I had made that conjecture back when Tokarsky constructed his example, but I am not skilled enough in billiard dynamics to prove it. $\endgroup$
    – Joseph O'Rourke
    Commented Oct 13, 2010 at 13:42
  • 1
    $\begingroup$ I too thought hard about the problem once, and I'm pretty sure I was able to prove that a light ray that goes out at an irrational angle in a rational polygon has a dense orbit. But I think this was almost certainly known. (If not, then perhaps I'll try to reconstruct the proof.) I then tried to prove the same thing without the rationality hypothesis and got nowhere. $\endgroup$
    – gowers
    Commented Oct 13, 2010 at 15:29
  • 1
    $\begingroup$ I am not completely convinced that one can solve the problem with mirrors for a finite number of dark points. Indeed, mirrors in the interior of the polygon can create new invisible points and regions. Putting a mirror in the interior of a polygon $P$ corresponds to branching in the developed surface with two ramifications of order two at the endpoints of the mirrors. $\endgroup$
    – Roland Bacher
    Commented Oct 14, 2010 at 7:32
  • 1
    $\begingroup$ @Roland: Yes, it is certainly not clear, and I did not mean to imply that it is evident. It would require a strategy for inserting a mirror while maintaining previous coverage, and I do not see how to guarantee that no new dark points would be thereby introduced. $\endgroup$
    – Joseph O'Rourke
    Commented Oct 14, 2010 at 12:00

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .