1
$\begingroup$

I have some questions regarding the strategy of the proof of the existence of Hilbert and Quot schemes (I will focus on the latter since it's more general), as in the book Fundamental Algebraic Geometry–Grothendieck's FGA Explained. (I have just a sketch of the related part and not go into the details yet.)

(1) I find the basic setting is restricted to the category ${\rm Sch}^{\rm locnoe}_S$ of locally noetherian schemes over a fixed locally noetherian schemes $S$. I think it first proves representability of the functor $\mathfrak{Q}uot_{E/X/S}$ in this category ${\rm Sch}^{\rm locnoe}_S$, then through Yoneda lemma, we can find a locally noetherian scheme ${\rm Quot}_{E/X/S}$ representing the functor $\mathfrak{Q}uot_{E/X/S}$, then we in particular regard this scheme ${\rm Quot}_{E/X/S}$ as in ${\rm Sch}_S$, the category of all $S$-schemes. Am I correct? (In my opinion, people only want it to be an $S$-scheme a priori; being locally noetherian is an extra property.)

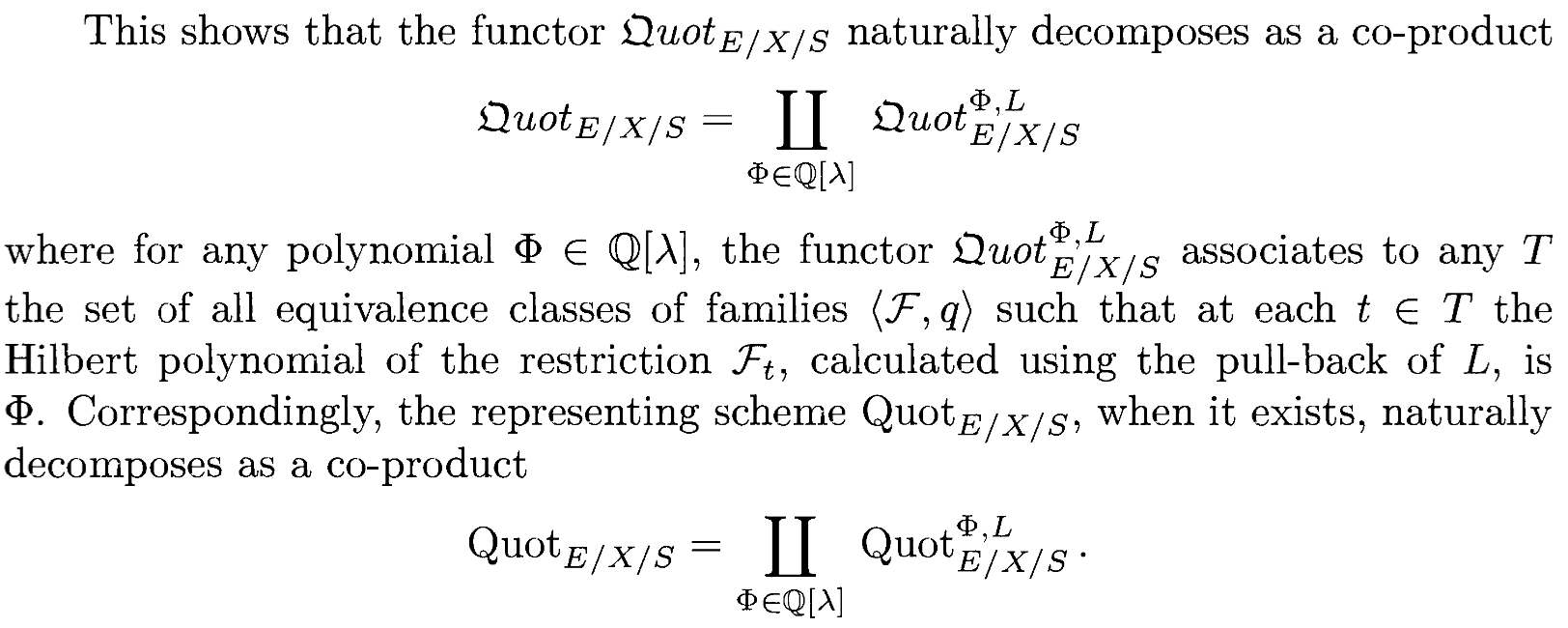
(2) This is more crucial to me. The following picture is from p.110 of the above-mentioned book.

enter image description here

I think, in general, Yoneda embedding doesn't commute with coproducts, i.e., we don't have $h_{\coprod Y_i}\cong\coprod h_{Y_i}$. How can one get a coproduct decomposition of the representative from a coproduct decomposition of the corresponding functor (in our situation, $\mathfrak{Q}uot_{E/X/S}$)?

Thanks in advance for those who answer my doubts!

Improve this question
$\endgroup$
11
  • 2
    $\begingroup$ The first coproduct decomposition does not actually take place in the category of presheaves. It takes place in the subcategory of product-preserving presheaves. The Yoneda embedding into that subcategory preserves coproducts. $\endgroup$
    – Marc Hoyois
    Commented Feb 15, 2022 at 18:48
  • $\begingroup$ You can use limit theorems as in EGA III to reduce representability to the Noetherian case, cf. the comments to the following MO question: mathoverflow.net/questions/259423/… $\endgroup$
    – Jason Starr
    Commented Feb 15, 2022 at 19:28
  • $\begingroup$ @ Marc Hoyois Thanks Marc! By "product-preserving presheaves", do you mean those presheaves taking (arbitrary) coproducts to products? (Namely, you are using that products in the opposite category are coproducts in the original one?) $\endgroup$
    – Lao-tzu
    Commented Feb 15, 2022 at 20:09
  • $\begingroup$ @Marc Hoyois Moreover, I think in the category of product-preserving presheaves, the value of the coproduct of (say two) product-preserving presheaves on an object is not simply the coproduct (i.e. disjoint union) of the values of these presheaves. $\endgroup$
    – Lao-tzu
    Commented Feb 15, 2022 at 20:26
  • $\begingroup$ Indeed, and correspondingly the value of the functor Quot on a non-connected scheme is not simply the coproduct of the values of the functors Quot^Phi, one has to make the latter product-preserving. $\endgroup$
    – Marc Hoyois
    Commented Feb 17, 2022 at 8:01

0

Reset to default

You must log in to answer this question.