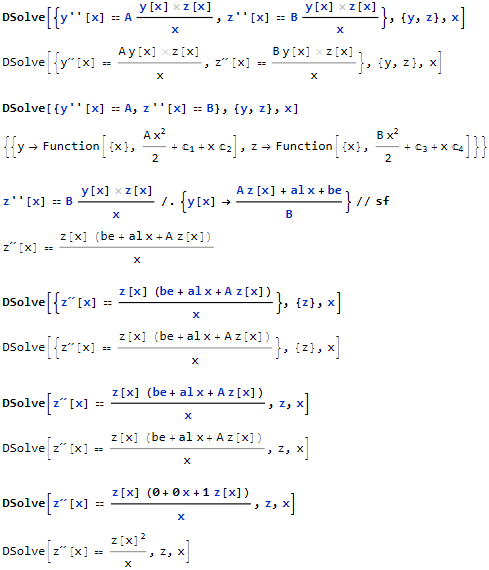I came across this coupled system of ODE in my research (related to foulant in reaction diffusion system): $$\frac{d^2y}{dx^2} - A\frac{yz}{x} = 0$$ $$\frac{d^2z}{dx^2} - B\frac{yz}{x} = 0$$ where $A$ and $B$ are constants. Can it be solved analytically?
$\begingroup$
$\endgroup$
1
-
1$\begingroup$ By multiplying $B$ to the first equation and $A$ to the second, the equation can be decoupled as $By-Az=\alpha x+\beta$, where $\alpha$ and $\beta$ only depend on the initial conditions. $\endgroup$– LeechLatticeCommented Feb 13, 2022 at 13:23
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
7
Mathematica cannot solve this system of ODEs, even after the decoupling suggested by user LeechLattice, even with $\alpha=\beta=0$ and $A=1$. This means that the existence of an explicit solution is highly unlikely.
Here is the image of the corresponding Mathematica notebook:
-
$\begingroup$ If the boundary condition shows that alpha and beta are 0, would that be solvable? $\endgroup$– JohnsonCommented Feb 13, 2022 at 15:44
-
$\begingroup$ @David : Even then, no, and even with $A=1$. See the added last two lines of the image. $\endgroup$ Commented Feb 13, 2022 at 16:15
-
$\begingroup$ Starting from $xz'' - Az=0,$ change variables to $z=u/x$ and then $x=e^t$ to reduce the system to $\ddot{u} -2\dot{u} +2u-Au^2,$ with no explicit $t$ dependence. It's now a classical mechanics problem with a damping term $-2\dot{u}$ which obstructs integrating directly. Maybe Mathematica can solve this equation? $v=u-1/A$ also switches the term in $u$ for a constant, although I don't know how much difference that makes. $\endgroup$– JojoCommented Feb 14, 2022 at 9:57
-
$\begingroup$ @Joe : No, Mathematica cannot do anything with these two transformed ODEs either, even with $A=1$. $\endgroup$ Commented Feb 16, 2022 at 4:09
-
$\begingroup$ @David : Do you have any further response to this answer? $\endgroup$ Commented Feb 16, 2022 at 4:10