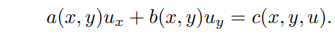3
$\begingroup$

I am interested in understanding complex first-order quasilinear partial differential equations. In the real setting there is a huge literature dealing with such equations but in the complex setting, I can not find a reference dealing with these types of equations. The form is a follows

enter image description here

I hope that someone knows some reference in the subject to let me know.

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ You want holomorphic PDEs or smooth? $\endgroup$
    – Ben McKay
    Commented Feb 9, 2022 at 15:27
  • 3
    $\begingroup$ One interesting source: almost complex structures on 4-manifolds have quasilinear Cauchy--Riemann equations for pseudoholomorphic curves. There are some explicitly integrable examples which are not complex. But these equations are smooth, not holomorphic. $\endgroup$
    – Ben McKay
    Commented Feb 9, 2022 at 15:38
  • $\begingroup$ I want just the smooth PDEs. $\endgroup$
    – Said Kamam
    Commented Feb 10, 2022 at 8:52

1 Answer 1

Reset to default
3
$\begingroup$

Vekua, I. N. Generalized analytic functions. Pergamon Press, London-Paris-Frankfurt; Addison-Wesley Publishing Co., Inc., Reading, Mass. 1962 xxix+668: starting at page 334, Vekua discusses the existence and uniqueness problem for complex valued quasilinear equations of the form you indicate, assuming they are uniformly elliptic. I would imagine that the reason you think of your system in term of complex numbers is because it is elliptic, so roughly similar to the Cauchy-Riemann equations.

I think there is also some quasi-linear theory in W. L. Wendland, Elliptic Systems in the Plane, Pitman, London, 1979, but I can't find a copy.

My PhD thesis dealt with the theory of (possibly fully nonlinear) first order elliptic systems for two real functions of two real variables, using symplectic geometry. The first thing you have to do is to find coordinates (or a $G$-structure) in which the equations look locally like Cauchy-Riemann equations, to get local estimates. The global theory is entirely in the hands of Gromov, using symplectic methods adapted from his famous paper on pseudoholomorphic curves. I didn't use much from Vekua or Wendland.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .