1
$\begingroup$

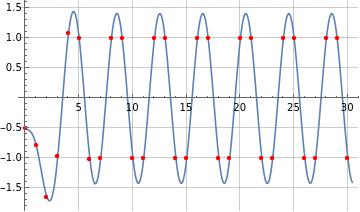
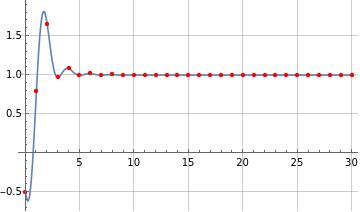
Define the sequence $$a_s=(-1)^{\binom{s-1}2}\left(\frac{\pi}2\right)^s\frac1{2\cdot s!}\begin{cases} s\,E_{s-1}, \qquad \text{if $s$ is odd} \\ 2^{2s}B_s, \qquad \,\,\text{if $s$ is even};\end{cases}$$ where $E_s$ and $B_s$ are Euler and Bernoulli numbers, respectively.

Of course, we know that $$\sum_{n=1}^{\infty}\frac1{n^{2s}}=a_{2s} \qquad \text{and} \qquad \sum_{n=1}^{\infty}\frac{(-1)^{n-1}}{(2n-1)^{2s-1}}=a_{2s-1}.$$ The focus here is that each $a_s$ is rational multiple of a power of $\pi$ unlike the classical Riemann zeta function at odd values of $s$. A few examples are in order: for $s\geq1$, $$\frac{\pi}4, \frac{\pi^2}6, \frac{\pi^3}{32}, \frac{\pi^4}{90}, \frac{5\pi^5}{1536}, \frac{\pi^6}{945}, \frac{61\pi^7}{184320}, \frac{\pi^8}{9450}, \,\,\dots$$ Now, I like to ask:

QUESTION. Is there a function $F(s)$, continuous in real $s$, whose values are $F(s)=a_s$ at integral $s$? One may try upgrading this to a meromorphic function.

Improve this question
$\endgroup$
3
  • $\begingroup$ Nice idea of course to accommodate those powers of $\pi$. But as the ratios of consecutive values form a zigzag pattern, I'd guess that $F''(x)$ would already have zeros between each pair of consecutive naturals... so I don't think there will be a very satisfying function to interpolate. $\endgroup$
    – Wolfgang
    Commented Jan 17, 2022 at 21:31
  • 3
    $\begingroup$ For any sequence $(b_n)_{n \in \mathbb{Z}}$ one can find an entire function $f$ such that $f(n) = b_n$. See math.stackexchange.com/a/1529860/304290 . To get an interesting answer you probably need more conditions on $F$. $\endgroup$
    – Bma
    Commented Jan 18, 2022 at 1:42
  • $\begingroup$ Wouldn't it be more "natural" to define $a_{2s}=\sum_{n=1}^{\infty}\frac{1}{(2n-1)^{2s-1}}$, that is rescaling it by $1-1/2^{2s}$? Or even better, what about $a_s=\sum_{n=1}^{\infty}\frac{e^{\pi i s(n-1)}}{(2n-1)^s}$? $\endgroup$
    – Yaakov Baruch
    Commented Jan 18, 2022 at 8:57

1 Answer 1

Reset to default
3
$\begingroup$

The function $$f(s):=\sin(\tfrac{\pi}{2}(s+1)) \zeta(s)+\sin(\tfrac{\pi s}{2}) L(\tfrac{s+1}{2})\tag{1}$$ where $L(s)$ is the second Dirichlet series $$L(s)=\sum\limits_{n=1}^{\infty } \frac{(-1)^{n-1}}{(2 n-1)^{2 s-1}}\tag{2}$$ which is valid for $\Re(s)>1$ but which can be analytically extended using the Hurwitz zeta function as $$L(s)=\frac{\zeta \left(2 s-1,\frac{1}{4}\right)-\zeta \left(2 s-1,\frac{3}{4}\right)}{4^{2 s-1}}\tag{3}$$ gives the two sequences except for this sign $$\underset{s\to 1}{\text{lim}}f(s)=-\frac{\pi }{4}, f(2)=\frac{\pi^2}{6}, f(3)=-\frac{\pi^3}{32}, f(4)=\frac{\pi^4}{90},\dots\tag{4}$$ and you can square the trigonometric functions to get the sign.

The plot of the function in Mathematica for 2<s<30 is a nice sine like wave, but this is all trivial.

Figure (1) below illustrates formula (1) for $f(s)$ in blue using formula (3) for $L(s)$ where the red discrete evaluation points illustrate the evaluation of formula (1) at integer values of $s$.

Illustration of Formula (1) for f(s)

Figure (1): Illustration of Formula (1) for $f(s)$

Squaring the $sin$ terms in formula (1) for $f(s)$ above leads to $g(s)$ defined below which evaluates correctly in sign as well as magnitude.

$$g(s)=\sin^2\left(\frac{\pi}{2}(s+1)\right)\ \zeta(s)+\sin^2\left(\frac{\pi s}{2}\right)\ L\left(\frac{s+1}{2}\right)\tag{5}$$

Figure (2) below illustrates formula (5) for $g(s)$ in blue using formula (3) for $L(s)$ where the red discrete evaluation points illustrate the evaluation of $a_s$ defined in the question above at integer values of $s$.

Illustration of Formula (5) for g(s)

Figure (2): Illustration of Formula (5) for $g(s)$ (blue curve) and $a_s$ (red evaluation points)

Improve this answer
$\endgroup$
6
  • $\begingroup$ I'm having problems verifying your result. By "where $L(s)$ is the second Dirichlet series", do you mean $L_{2,1}(s)=\left(1-2^{-s}\right) \zeta (s)$? $\endgroup$
    – Steven Clark
    Commented Jan 18, 2022 at 20:33
  • $\begingroup$ @Clark my $L(s)$ is defined as $$L(s)=\sum_{n=1}^\infty \frac{(-1)^{n-1}}{(2n-1)^{2s-1}}.$$ $\endgroup$
    – juan
    Commented Jan 18, 2022 at 20:34
  • $\begingroup$ @juan: do you mind posting the Mathematica plot? $\endgroup$
    – T. Amdeberhan
    Commented Jan 18, 2022 at 21:06
  • $\begingroup$ @Amdeberham I am not sure I know how to do that. L[s_] := Sum[(-1)^(n - 1)/(2 n - 1)^(2 s - 1), {n, 1, Infinity}]; f[s_] := Sin[(Pi (s + 1))/2] Zeta[s] + Sin[(Pi (s))/2] L[(s + 1)/2]; Table[f[s], {s, 2, 8}] $\endgroup$
    – juan
    Commented Jan 18, 2022 at 22:13
  • $\begingroup$ @Amdeberham it was interesting the Plot of the complex function $t \mapsto e^{-\pi t/2} \zeta(1/2+it). The zeros of this function are not on the critical line. (in general). $\endgroup$
    – juan
    Commented Jan 18, 2022 at 22:16

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .