2
$\begingroup$

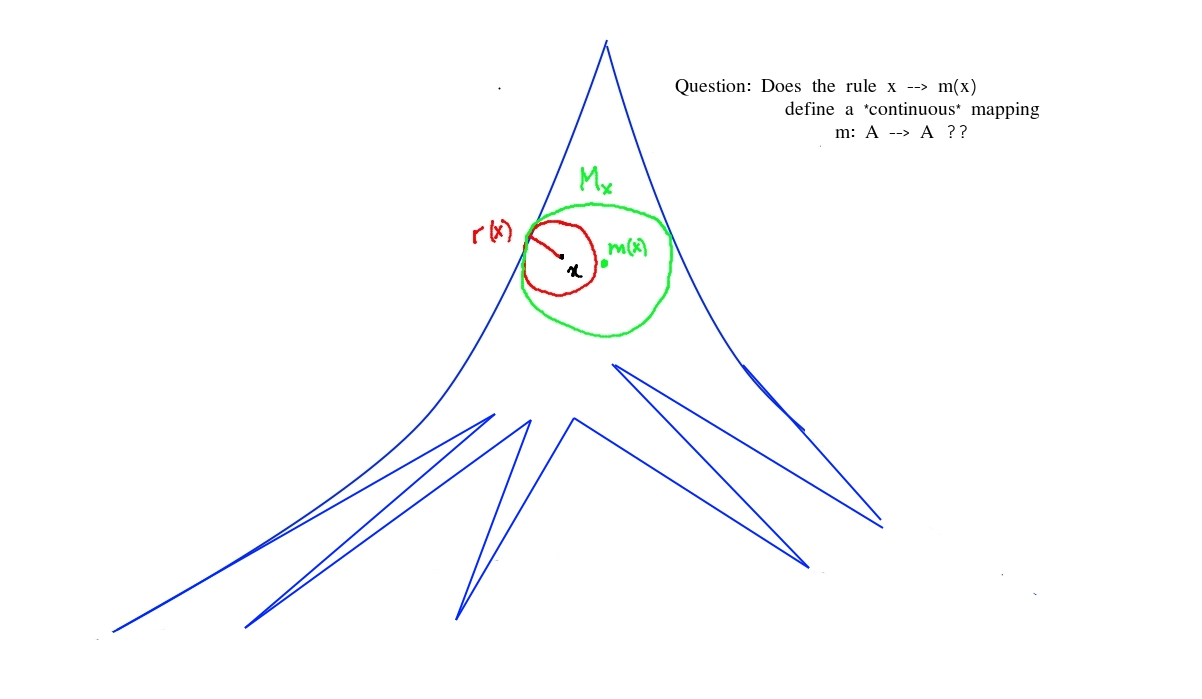
Let $A$ be an open bounded subset of euclidean $n$-space $\mathbb{R}^n$. For $x\in A$, let $r=r(x)$ be the maximal radius such that the ball centred at $x$ with radius $r=r(x)$ is contained in $A$, i.e. $r(x)$ is the maximal $r>0$ satisfying $B_r(x) \subset A$. N.B. the radius $r(x)$ coincides with distance-to-the-boundary $dist(x,\partial A)$.

For $x\in A$, let $M_x$ be the maximal ball containing $B_{r(x)}(x)$ and contained in $A$. Thus $M_x$ is the maximal ball satisfying $B_{r(x)}(x) \subset M_x \subset A$.

My question: If we assume $A$ is open and bounded, then does $x\mapsto M_x$ vary continuously with $x$?

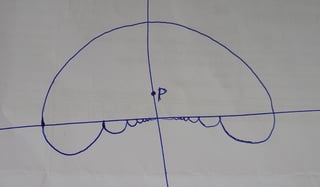
enter image description here

N.B. SRM has provided nice class of counterexamples in his answer, showing the answer is no whenever the medial axis is noncompact and has nontrivial accumulation points (i.e. Cauchy sequences in the euclidean distance).

N.B. For given $x$, I argue that there exists a unique maximal ball $M_x$ satisfying the above conditions.

Remark. For my purposes a positive answer would imply that the centre $m$ of $M_x$ varies continuously with $x$. Thus we would obtain a continuous map $x\mapsto m(x)$ from $x$ to the centre $m(x)$ of the max ball $M_x$.

Remark. I remember reading an article/book of Vitali Kapovich which had some similar constructions, but I cannot recall the specifics. My goal is to establish continuity of $x\mapsto m(x)$ with mild hypotheses on $A$, i.e. without requiring a $C^1$ boundary.

Remark. There is a variational characterization of the max-centre defined as the argmax of a convex function on a convex domain. Therefore the argmax is unique element on the boundary of the domain, and varies continuously with respect to the datum.

Improve this question
$\endgroup$
1
  • $\begingroup$ Yes I've added a figure to illustrate the meaning of $r(x), M_x, m(x)$. $\endgroup$
    – JHM
    Commented Jan 10, 2022 at 13:00

1 Answer 1

Reset to default
3
$\begingroup$

The result is not necessarily true. Consider for $n\geq1$ the open ball $R_n$ with diameter from $(\frac{1}{n},0)$ to $(\frac{1}{n+1},0)$. Let $L_n$ be the reflection of $R_n$ with respect to the $y$ axis.

enter image description here

Now let $A$ be the union of the $L_n$, the $R_n$ and the upper half of the open unit disc. Then the function $x\mapsto m(x)$ is not continuous at $p=(0,0.1)$. This is because $m(p)=(0,0.5)$, but for the sequence of points $p_n=(\frac{1}{2}(\frac{1}{n}+\frac{1}{n+1}),0.1)$, we have $m(p_n)=p_n$ for $n\geq2$.

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ I don´t know what condition could work. There are convex counterexamples (consider the disk $D(0,1)$ and the points $e^\frac{i}{n}$ in its boundary, and the tangent lines at those points. Then the convex region delimited by those lines and the disk, (the disk but adjoining a sequence of small "triangles"), seems to be a counterexample too). Maybe some strong differentiability condition on the boundary could work. Also, my example isn´t stable, if by stable you mean under small changes (small in Hausdorff distance) or sth like that. Have fun with the medial axis of the bean :D $\endgroup$
    – Saúl RM
    Commented Jan 10, 2022 at 17:33
  • $\begingroup$ Interesting bean example! Thank you. $\endgroup$
    – JHM
    Commented Jan 10, 2022 at 18:48
  • $\begingroup$ The medial axis is like infinite trivalent tree whose branches accumulate to the vertical line segment $(0,t), 0\leq t \leq 1/2$ in the plane. The points $p_n$ belong to the distinct "branches". So you've identified the fact that $m$ is discontinuous whenever the medial axis is not relatively closed in $A$. $\endgroup$
    – JHM
    Commented Jan 10, 2022 at 19:30
  • $\begingroup$ Hm but in the set $\{(x,y)\in\mathbb{R}^2;10>y>x^2;\}$, the medial axis is not closed in $A$ (the point $(0,0.5)$ is in the closure) but $m$ seems to be continuous $\endgroup$
    – Saúl RM
    Commented Jan 10, 2022 at 19:53
  • $\begingroup$ It's something about Cauchy sequences in the background euclidean distance which are not Cauchy sequences in the subset metric. In your bean example, the branches accumulate w.r.t. euclidean distance but are not Cauchy in the subset metric (as measured in the medial axis along the branches). This is some kind of metric condition on the inclusion $M(A)\hookrightarrow A$. $\endgroup$
    – JHM
    Commented Jan 10, 2022 at 20:13

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .