$\newcommand{\ep}{\varepsilon}\newcommand{\Ga}{\Gamma}\newcommand{\de}{\delta}$Here is a proof of your first claim:
Integral representation:
We have the integral representation
\begin{equation*}
\sqrt\pi\,\text{Li}_{1/2}(x)=\int_0^\infty \frac{t^{-1/2}\,dt}{e^t/x-1}.
\end{equation*}
Here and in what follows, $\ep\in(0,\infty)$, $x\in(0,1)$, and $t\in(0,\infty)$.
So,
\begin{equation*}
f(x):=\sqrt\pi\,\big(\sqrt{-\ln x}\big)^{1+\ep}\text{Li}_{1/2}(x)
=\int_0^\infty dt\,t^{-1/2} g_t(x), \label{1}\tag{1}
\end{equation*}
where
\begin{equation*}
g_t(x):=\frac{\big(\sqrt{-\ln x}\big)^{1+\ep}}{e^t/x-1}.
\end{equation*}
So,
\begin{equation*}
f'(x)
=\int_0^\infty dt\,t^{-1/2} g_t'(x)
\end{equation*}
and hence
\begin{equation*}
-2\big(-\ln x\big)^{(1-\ep)/2}f'(x)
=\int_0^\infty dt\,t^{-1/2} h_x(t), \label{2}\tag{2}
\end{equation*}
where
\begin{equation*}
h_x(t):=\frac{(1+\ep) (e^t-x)+2 e^t \ln x}{(e^t-x)^2}.
\end{equation*}
Main difficulty: It is that, for each $\ep\in(0,1]$ and each $x$ in a left neighborhood of $1$, $h_x(t)$ changes its sign (from $-$ to $+$) as $t$ goes from $0$ to $\infty$. Moreover, it does not seem easy to appropriately bound $h_x(t)$ from below.
If such a lower bound on $h_x(t)$ were easily available, we could just note that $h_x(t)\underset{x\uparrow1}\longrightarrow\frac{1+\ep}{e^t-1}>0$ and then use Fatou's lemma to conclude that $f'<0$ in a left neighborhood of $1$, which would imply that
for each $\ep\in(0,\infty)$ there is a nonempty interval $I\subset(0,1)$ such that $\big(\sqrt{-\ln x}\big)^{1+\ep}\text{Li}_{1/2}(x)$ is strictly decreasing in $x\in I$.
To overcome this "sign-changing" difficulty, we seem to have to work hard, as follows.
Replacing the integrand by a simpler, appropriately approximating integrand:
We have $|\ln x-(x-1)|\le(1-x)^2/2$ and hence
\begin{equation*}
|h_x(t)-H_x(t)|\le\de_x(t):=\frac{e^t(1-x)^2}{(e^t-x)^2}\le e^{-t},
\end{equation*}
where
\begin{equation*}
H_x(t):=\frac{(1+\ep) (e^t-x)-2 e^t(1-x)}{(e^t-x)^2}. \label{2.5}\tag{2.5}
\end{equation*}
Also, for each real $t>0$ we have $\de_x(t)\to0$ as $x\to1$.
Therefore and by dominated convergence,
\begin{equation*}
\int_0^\infty dt\,t^{-1/2}|h_x(t)-H_x(t)|\to0
\end{equation*}
as $x\uparrow1$.
So, it suffices to show that
\begin{equation*}
K(x):=\int_0^\infty dt\,t^{-1/2} H_x(t)\underset{x\uparrow1}\longrightarrow\infty \label{3}\tag{3}
\end{equation*}
-- then, in view of \eqref{1} and \eqref{2}, it will follow that
for each $\ep\in(0,\infty)$ there is a nonempty interval $I\subset(0,1)$ such that $\big(\sqrt{-\ln x}\big)^{1+\ep}\text{Li}_{1/2}(x)$ is strictly decreasing in $x\in I$.
Integrating part of the integrand by parts, to get a further improved integrand:
Integrating by parts, we get
\begin{equation*}
\int_0^\infty dt\,t^{-1/2} \frac{(1+\ep)(e^t-x)}{(e^t-x)^2}
=\int_0^\infty dt\,t^{-1/2} \frac{2(1+\ep)te^t}{(e^t-x)^2}
\end{equation*}
and hence, in view of \eqref{3} and \eqref{2.5},
\begin{align*}
\tfrac12 K(x)&=\int_0^\infty dt\,t^{-1/2} \frac{(1+\ep)te^t- e^t(1-x)}{(e^t-x)^2} \\
& =\int_0^\infty dt\,t^{-1/2}e^{-t} \frac{(1+\ep)t-(1-x)}{(1-xe^{-t})^2}. \label{3.5}\tag{3.5}
\end{align*}
Minorizing the further improved (sign-changing) integrand by a yet simpler one, with an elementary integral:
Note that the latter integrand has the same sign as $t-t_x$, where
\begin{equation*}
t_x:=\frac{1-x}{1+\ep},
\end{equation*}
and, by the convexity of the exponential function, $e^{-t}-(1-k_x t)$ also has the same sign as $t-t_x$, where
\begin{equation*}
k_x:=\frac{1-e^{-t_x}}{t_x}.
\end{equation*}
Also, $v\frac1{(1-xv)^2}$ is increasing in $v\in(0,1)$, for each $x\in(0,1)$.
It follows that
\begin{equation*}
\tfrac12 K(x)>L(x)
:=\int_0^{\sqrt{1-x}} dt\,t^{-1/2}(1-k_x t) \frac{(1+\ep)t-(1-x)}{(1-x(1-k_x t))^2} \label{4}\tag{4}
\end{equation*}
(since $\sqrt{1-x}>t_x$, the integrand in \eqref{3.5} is $>0$ for $t>\sqrt{1-x}$.)
Completing the proof:
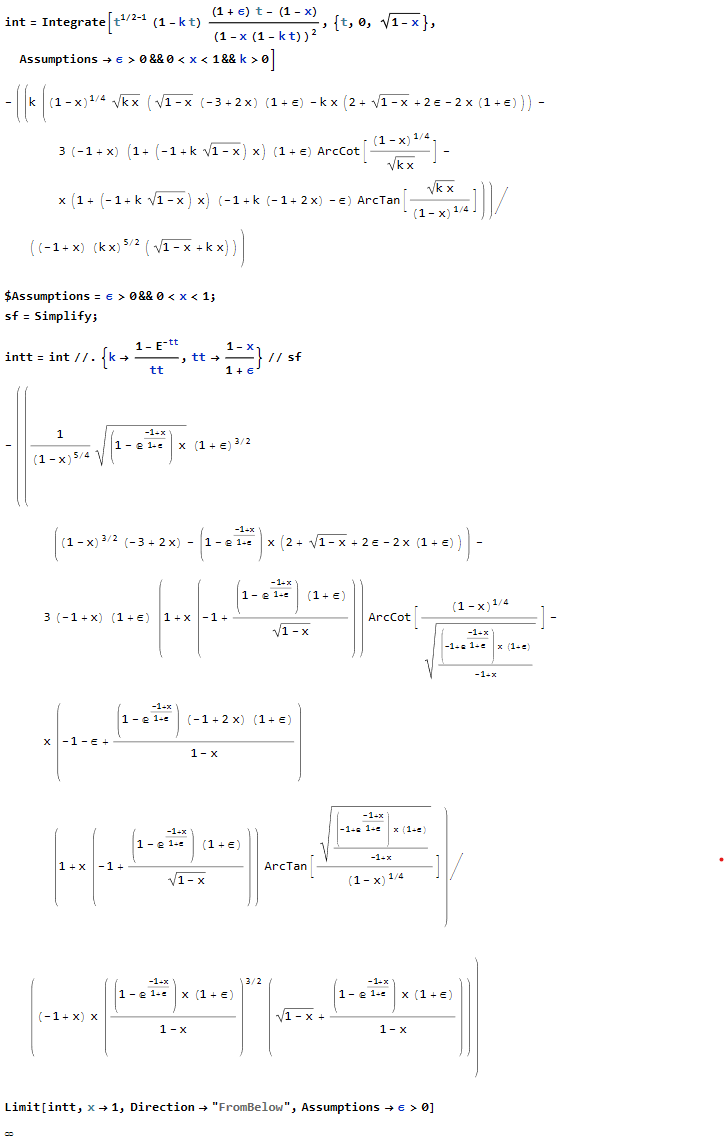
The integral $L(x)$ is elementary, even if rather cumbersome. It is also elementary, even if tedious, to show that $L(x)\to\infty$ as $x\uparrow1$.
Thus, \eqref{3} follows from \eqref{4}, and we are done.
Details of the calculation of $L(x)$ and its left limit $L(1-)$ are presented in the image of a Mathematica notebook below (click on the image to magnify it):