14
$\begingroup$

Let $\rho$ range over the non-trivial zeroes of the Riemann zeta function. Let $$M(T) = \max_{|\Im \rho|\leq T} \left|\mathrm{Res}_{s=\rho} \frac{1}{\zeta(s)}\right| = \max_{|\Im \rho|\leq T} \frac{1}{|\zeta'(\rho)|}.$$ Are there any conjectures, or even just vague, reasonable guesses, as to what the growth of $M(T)$ should be?

Here's a picture of $1/|\zeta'(\rho)|$ against $(\log \Im \rho)^2$ for the first 50000 zeros with $\Im \rho>0$. The green line is of slope $1/3$, i.e., it corresponds to a bound of $(\log T)^2/3$.

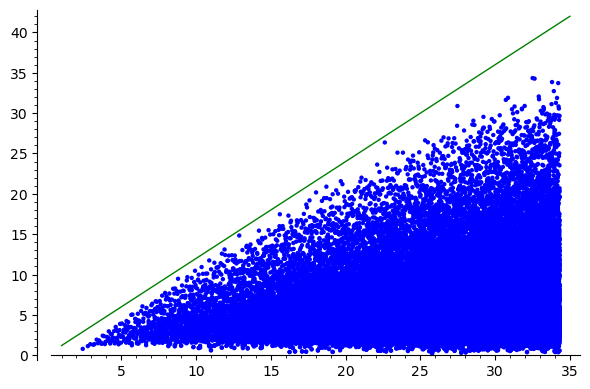
Addendum (following @Lucia's comment): here is a plot of $1/|\zeta'(\rho)|$ against $(\Im \rho)^{1/3}$ for the first $50000$ zeros. The green line is of slope $6/5$, that is, it corresponds to a bound $\frac{6}{5} T^{1/3}$.

enter image description here

Residues courtesy of D. Platt.

Improve this question
$\endgroup$
19
  • 1
    $\begingroup$ Residue of $f$ equals $1/f'$ only when the root of $f$ is simple, is this known for Zeta? $\endgroup$
    – Fedor Petrov
    Commented Dec 17, 2021 at 13:38
  • 2
    $\begingroup$ It's not known but believed for $\zeta$. $\endgroup$
    – H A Helfgott
    Commented Dec 17, 2021 at 13:38
  • 10
    $\begingroup$ The usual guess is that pair correlation predicts that up to height $T$ there may be a pair of zeros that are within $T^{-1/3 +o(1)}$ of each other. This then suggests that$1/\zeta'(\rho)$ maybe grows like $T^{1/3 +o(1)}$. This is an unpublished conjecture of Gonek. For related work, see for example arxiv.org/pdf/2003.09368.pdf and references therein. $\endgroup$
    – Lucia
    Commented Dec 17, 2021 at 16:25
  • 4
    $\begingroup$ related: mathoverflow.net/questions/394323/… $\endgroup$
    – Terry Tao
    Commented Dec 17, 2021 at 17:49
  • 1
    $\begingroup$ @HAHelfgott then your second equality is only conjectural $\endgroup$
    – Fedor Petrov
    Commented Dec 17, 2021 at 18:49

0

Reset to default

You must log in to answer this question.