$\newcommand{\id}{\mathrm{id}}$My reference for this post is Musto, Reutter and Verdon's A compositional approach to quantum functions, arXiv:1711.07945. Questions are in bold below. Allow me to begin with a bit of background. Taking inspiration from Gelfand duality for commutative $C^*$-algebras, we take the notion of a quantum set to be a Frobenius algebra, which is defined to be a finite dimensional Hilbert space $H$, which is a unital algebra, with multiplication $\mu : H\otimes H\to H$ and unit $\eta : \mathbb{C}\to H$, that has a co-multiplication $\delta : H\to H\otimes H$ and co-unit $\epsilon : H\to \mathbb{C}$, and satisfies the Frobenius condition: $$(\mu\otimes \id)(\id\otimes\delta) = \delta\circ\mu = (\id\otimes \mu)(\delta\otimes \id).$$ A SSFA is a special symmetric Frobenius algebra (which is just a special class of Frobenius algebras (see the above arXiv reference)). Given a linear function $f : A\to B$ between SSFA's $A$ and $B$, the dagger $f^\dagger : B^*\to A^*$ is just the Hilbert space adjoint of $f$. Then $\delta = m^\dagger$ and $\epsilon = \eta^\dagger$.
There is a diagrammatic approach to the formulation a Frobenius algebra, which involves replacing the algebraic axioms of multiplication, unitality, co-multiplication, etc, with nice string diagrams.
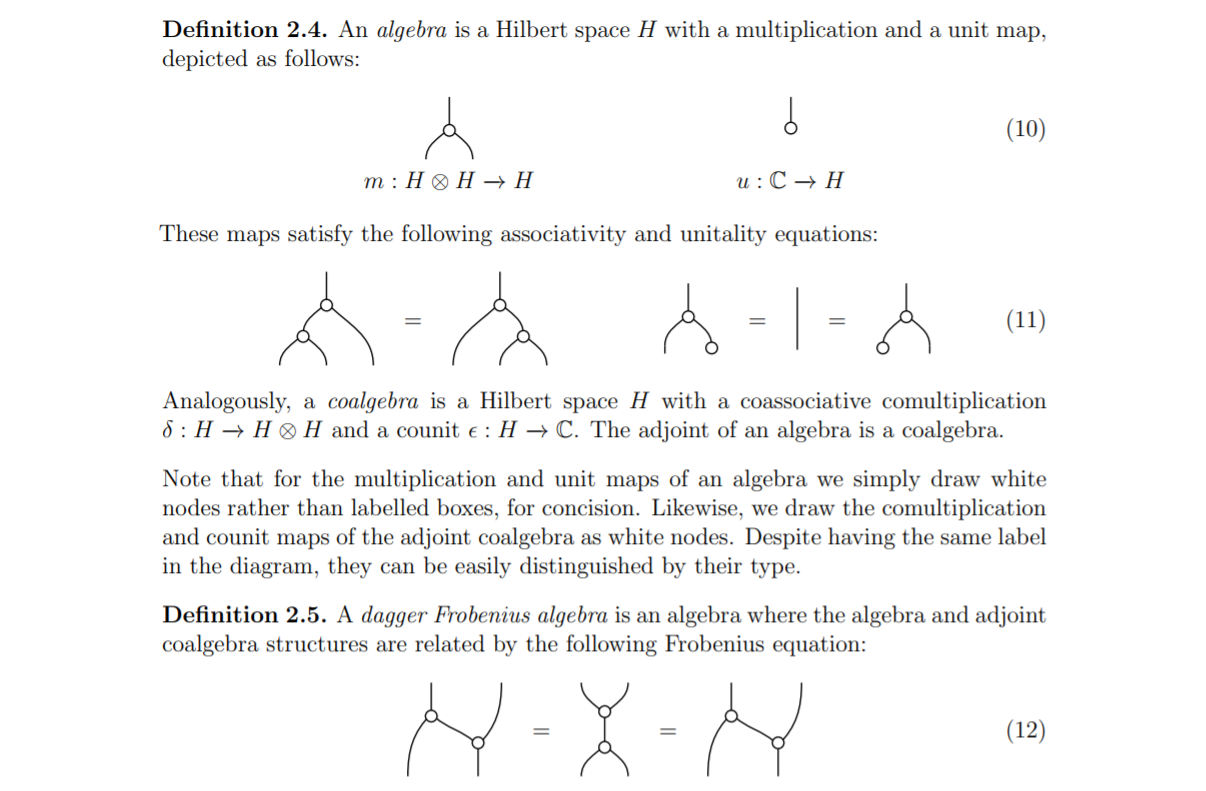 What I am concerned about lies in the image below.
What I am concerned about lies in the image below.
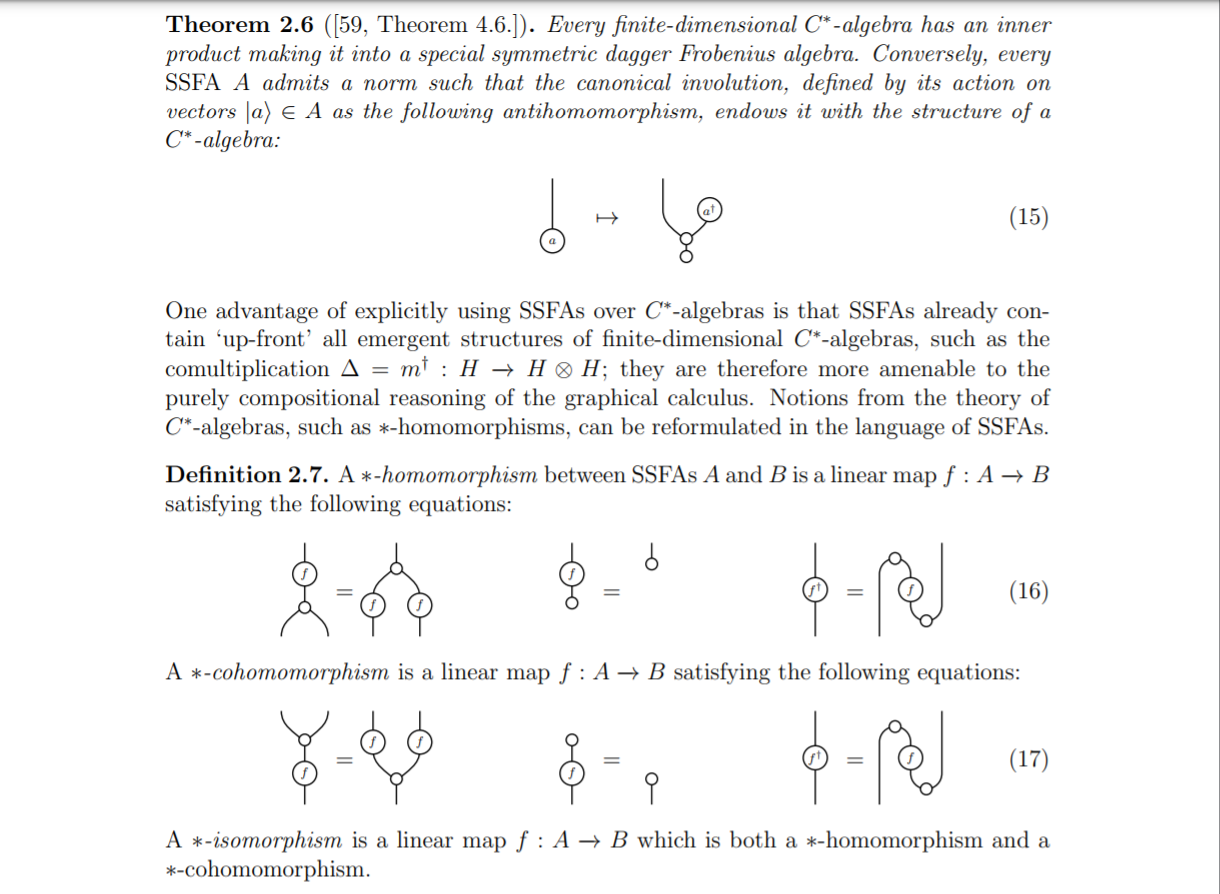 Question 1: How do we interpret the formulation of the $C^*$-involution in Theorem 2.6 algebraically (diagram (15))?
Question 1: How do we interpret the formulation of the $C^*$-involution in Theorem 2.6 algebraically (diagram (15))?
Question 2: How do we interpret the diagram for the "dagger formula" of a $*$-homomorphism / $*$-co-homomorphism in Definition 2.7 algebraically (third diagram on the right in (16) / (17))?
Question 2 is what I'm really after, but I figure the main gap is my understanding of question 1. The multiplicative / co-multiplicative and unital / co-unital diagrammatic properties in Definition 2.7 are clear to me. The diagram in question for Definition 2.7 should correspond to the involution property of a $*$-morphism: $f(x^*) = f(x)^*$. I am not seeing it when I try to convert the diagram into algebra. Here is may failed attempt.
The upside popsicle diagram in Theorem 2.6 looks to me like it should be the "point map" $a : \mathbb{C}\to A$, $\lambda\mapsto \lambda a$. Then $a^\dagger : A^*\to \mathbb{C}$ is the adjoint of the map $a$, and replacing the strings with their definitions in terms of the co-multiplication and unit, we get that the involution is $$a\mapsto (\id\otimes a^\dagger)\delta(1) = 1\otimes a^\dagger(1) = a^\dagger(1)\in \mathbb{C}.$$ This is not an antiautomorphism of $A$ ,so, obviously, I am quite confused about these diagrams.
I am knowledgeable on Woronowicz's formulation of a compact quantum group and its operator algebraic aspects. I have some (albeit limited) knowledge on Frobenius algebras defined in terms of Frobenius forms and in terms of algebras and co-algebras. More generally, I am knowledgeable on the basics of operator algebras. My main my goal with this paper is to understand the notion of a quantum function and a quantum element of a quantum set. In particular, I find Proposition 4.12 intriguing. It seems like it's possibly a non-commutative analogue of the fact classical permutations are just bijections on a classical finite set. For this, I am required to translate the concepts into a language I understand.
Any help is much appreciated!
