Let $Z_i:=\nu_i$, $m:=e_1$, and $n:=e_2$. We want to find a bound on the tails of the pdf $f_X$ of the random variable (r.v.)
\begin{equation*}
X:=UV,
\end{equation*}
where
\begin{equation*}
U:=|Z_1|^m,\quad V:=|Z_2|^n.
\end{equation*}
Indeed, if $m$ and $n$ are both even, then $X=Y:=Z_1^m Z_2^n$, and otherwise $X=|Y|$ and the r.v. $Y$ is symmetric, so that $f_Y(y)=f_X(y)1(y>0)/2+f_X(-y)1(y\le0)/2$ for all real $y$, where $f_Y$ is the pdf of $Y$.
The pdf $f_X$ is the multiplicative convolution of the pdf $f_U$ and $f_V$ of the positive r.v.'s $U$ and $V$: for $x>0$,
\begin{equation*}
f_X(x)=\int_0^\infty\frac{dy}y\, f_U(x/y)f_V(y).
\end{equation*}
Also,
\begin{equation*}
f_U(u)=\frac2m u^{1/m-1}f(u^{1/m}),\quad f_V(v)=\frac2n v^{1/n-1}f(v^{1/n}) \tag{0}
\end{equation*}
for positive $u,v$, where $f$ is the pdf of $N(0,1)$.
So, for $x>0$,
\begin{equation*}
f_X(x)=\frac2{\pi mn}\int_0^\infty dy\,x^{1/m-1}y^{1/n-1/m-1}\exp\{-[(x/y)^{2/m}+y^{2/n}]/2\}.
\end{equation*}
Making here the substitution $y=x^{an/2}z$, where
\begin{equation*}
a:=\frac2{m+n}, \tag{1}
\end{equation*}
we have
\begin{equation*}
f_X(x)=\frac2{\pi mn}\,x^b\int_0^\infty dz\,z^{1/n-1/m-1}\exp\{-x^a g(z)/2\},
\end{equation*}
where $b:=1/m-1+(1/n-1/m)an/2=a-1$ and
\begin{equation*}
g(z):=z^{-2/m}+z^{2/n}.
\end{equation*}
So, by standard asymptotic analysis,
\begin{equation*}
f_X(x)\sim A\,x^{a/2-1}\exp\{-Gx^a/2\} \tag{2}
\end{equation*}
as $x\to\infty$, where
\begin{equation*}
G:=\min_{z>0}g(z)=g(z_*)=m^{-\frac{m}{m+n}} n^{-\frac{n}{m+n}} (m+n),
\end{equation*}
\begin{equation*}
z_*:=\left(\frac{n}{m}\right)^{\frac{m n}{2 (m+n)}},
\end{equation*}
and
\begin{equation*}
A:=\frac2{\pi mn}\,\sqrt{2\pi}\, \frac{z_*^{1/n - 1/m - 1}}{\sqrt{g''(z_*)/2}}
=\frac2{\sqrt{\pi(m+n)}} \, m^{-\frac{m}{2 (m+n)}} n^{-\frac{n}{2 (m+n)}}.
\end{equation*}
Thus, the exact exponent $a$ of $x$ in the term $\exp\{-Gx^a/2\}$ in (2) is given by (1). (This exponent is better than the corresponding exponent you had in the upper bound on the tail probability.)
Note that (2) will hold for all real $m,n>0$. That the exact exponent $a$ depends only on the total $m+n$ of the exponents of $Z_1$ and $Z_2$ in $Z_1^m Z_2^n$ seems quite reasonable. Moreover, the roles of $m$ and $n$ on the right-hand side of (2) are interchangeable, as they should be. Furthermore, in the limit cases when $m\downarrow0$ or $n\downarrow0$, (2) is in agreement with (0).
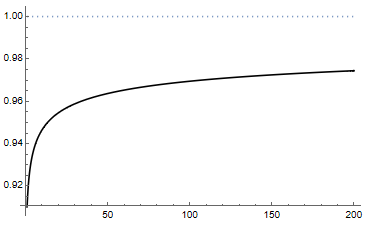
Here is the graph of the ratio of $f_X(x)$ to the right-hand side of (2) for $m=3$, $n=4$, and $x\in[1,200]$: