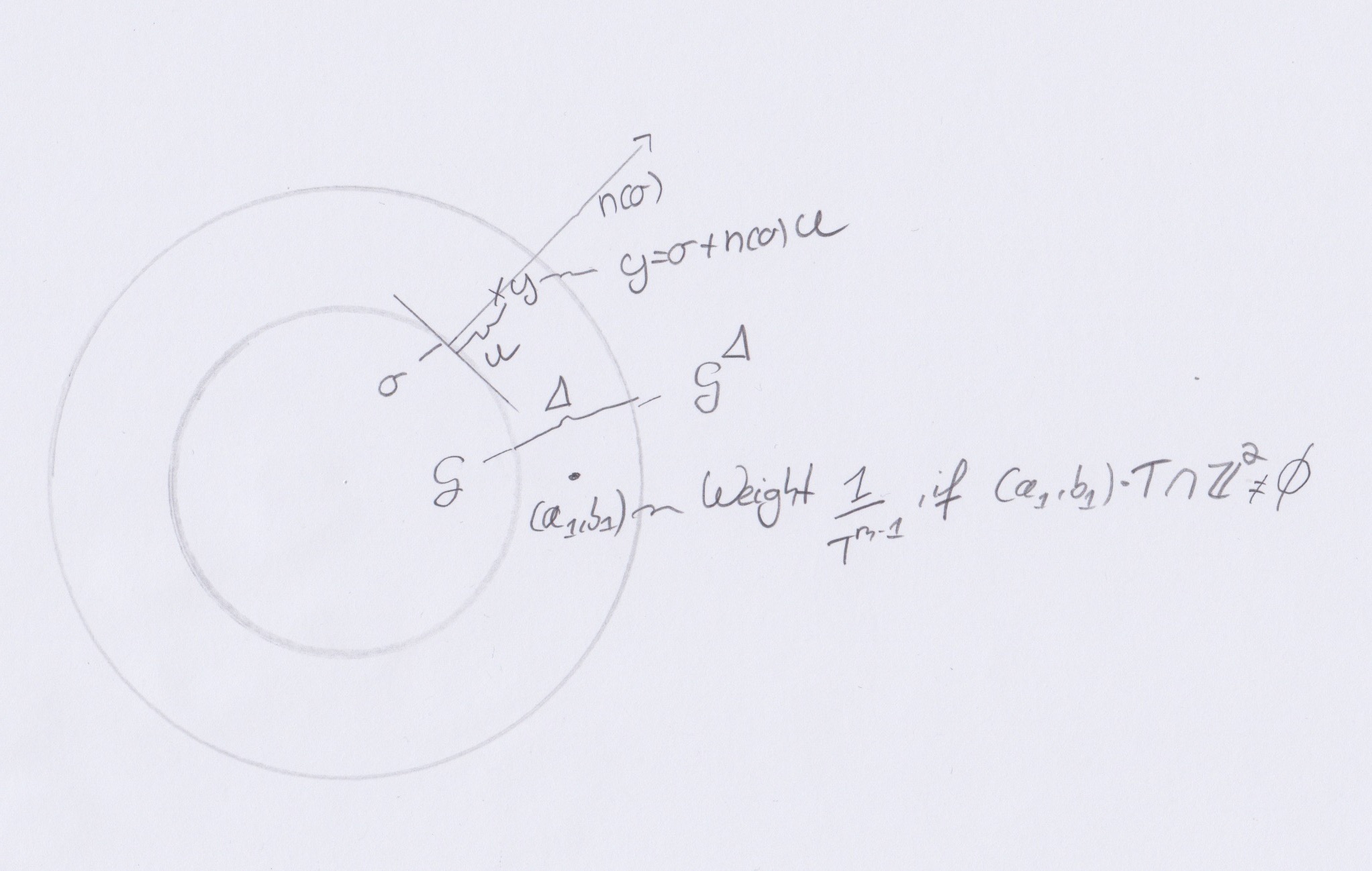
Let $G$ be the open $n$-ball in $\mathbb{R}^n$ and $G^\Delta$ the set of points in $\mathbb{R}^n$ with distance less than $\Delta>0$ from $G$.
Let $G_T=\{Tx: x\in G \}$ and $G_T^\Delta = \{ Tx: x \in G^\Delta\}$.
Let $\partial G$ be the boundary of $G$, $n(\sigma)$ the outer unit normal vector at the point $\sigma \in G $ and $u>0$.
Every point $y \in G^\Delta \setminus G$ can be uniquely identified by the pair $(u,\sigma)\in ([0,\infty),\partial G)$ through the relationship $y=\sigma + n(\sigma)u$.
Let $T>0$. $\mu_T$ is the measure in $G^\Delta \setminus G$ consisting of atoms of weight $T^{-(n-1)}$ at the points $$ \{ \omega \in G^\Delta \setminus G: T\omega \in \mathbb{Z}^n \}. $$
$\bar\mu_T$ is the measure in $G_T^\Delta \setminus G_T$ consisting of atoms of weight $T^{-(n-1)}$ at the points $$ \{ \omega \in G_T^\Delta \setminus G_T: \omega \in \mathbb{Z}^n \}. $$
For more clarity I added a small sketch for the $2$-dimensional case.
I would like to understand the proof of the statement:
The measure $\mu_T$ converges weakly on the set $[0,\Delta)\times \partial G$ as $T\rightarrow \infty$ to the measure $\mu$ equal to the direct product of the Lebesgue measures on $[0,\Delta)$ and $\partial G$.
Proof: The proof uses the following lemma
Lemma 1: Let $$\phi(t_1,...,t_r)\in C^2((a_1,b_1)\times...\times(a_r,b_r)),\\ \bigg|\frac{\partial^2 \phi}{\partial t_1^2} \bigg|\geq C >0,\quad \xi=\prod_{j=1}^m(b_j-a_j),\quad T \in (0,+\infty), $$ let $P_T$ be the measure on $[0,1]$ constituted by atoms of weight $T^{-r}$ at the points $\{T\psi(l_1/T,...,l_r/T) \}$, where $\{ \}$ stands for the operation of taking the fractional part and $l_j$ runs through independent integral values in the intervals $(Ta_j,Tb_j)$. Then $\xi^{-1}P_T$ converges weakly to the Lebesgue measure on [0,1] as $T \rightarrow \infty$.
Proof: We consider the conditional measure $P'_T$ for fixed $l_2,l_3,...,l_r$. The author of the paper claims that $$ \int_0^1e^{2\pi i m t} dP'_T(t)<C_1(\phi)\frac{\sqrt{|m|}}{\sqrt{T}} $$ for integral $m \neq 0$ holds.
Question 1: Why is this inequality correct?
Hence $$ \int_0^1 e^{2\pi i m t}dP_T(t) \rightarrow 0 $$ as $T\rightarrow \infty$. This proves Lemma 1.
Question 2: Why does the lemma follow from this (Levy continuity theorem, Weyl criterion, something else...)
Main part of the proof:
Each sufficiently small open subset of $\partial G$ can be represented as the graph $\Gamma$ of a 2-smooth function $\phi(\tau)$ for $\tau \in V$, where V is an open subset of the hyperplane perpendicular to one of the basis vectors e of the space $\mathbb{R}^n$ in which $\mathbb{Z}^n$ is imbedded canonically.
Let $t(\sigma)$ be the cosine of the angle between e and $n(\sigma)$. We suppose that $t(\sigma)>0$ for $\sigma \in \Gamma$.
Let $\Pi$ be an open (n-1)-dimensional parallelepiped with edges parallel to the basis vector of $\mathbb{R}^n$, $\Pi\subset V$; $\Gamma(\Pi)$ is the graph of the function $\phi(\tau)$ for $\tau \in \Pi$, $\xi$ is the Lebesgue measure of $\Pi$ for dimension $n-1$, $\eta = \max_{\sigma \in \Gamma(\Pi)}t(\sigma)$, and $a,b\in \mathbb{R}, 0<a<b<\Delta\eta^{-1}$.
We introduce the sets $F\subset [0,\Delta)\times\partial G$ and $H_T \subset \mathbb{R}^n$ in the following way:
$$ F=\{(u,\sigma): \sigma \in \Gamma(\Pi), at(\sigma)<u<bt(\sigma) \} $$ $$ H_T=\{ (p+qe): p\in T \Pi, a <T\phi(pT^{-1})-q<b \}. $$ Question 3: Why these two particular sets?
For sufficiently large T, $H_T \subset G_T^\Delta \setminus G_T$. We define a map $W: G_T^\Delta \setminus G_T \rightarrow [0,\infty)\times \partial G$ by the formula $$ W(y)=\bigg(u,\frac{\omega}{T} \bigg). $$ We put $F_T=W(H_T)$. It follows from Lemma 1 that $$ \lim_{T\rightarrow \infty} \mu_T(F_T)=\lim_{T\rightarrow \infty}\bar\mu_T(H_T)=\xi(b-a). \quad (*) $$ Question 4: I don't see the connection to the fractional part in Lemma 1.
In addition, $\mu(F)=\xi(b-a)$.
Question 5: Why?
We introduce $a_i,b_i$ and $\Pi_i$, i=1,2, subject to the same conditions as $a,b$ and $\Pi$. Let $a_1 <a<a_2$, $b_1<b<b_2$ and $\Pi_1 \subset \Pi \subset \Pi_2$, and let the boundaries of $\Pi_1$, $\Pi$ and $\Pi_2$ be mutually disjoint. We define $H_{T,i}$, $F_{T,i}$ and $\xi_i$ as above. For large enough T we have $F_{T,1}\subset F \subset F_{T,2}$. We apply (*) for $i=1,2$ and let $a_i$ tend to $a$ and $b_i$ to $b$ and $\Pi_i$ to $\Pi$, and we obtain $$ \lim_{T\rightarrow \infty} \mu_T(F)=\xi(b-a). $$ If $t(\sigma)<0$, we replace the basis vector e by -e and carry out the same reasoning. Because of the arbitrariness of the choice of a, b and $\Pi$ the statement is proved.
Question 6: Why? What theorem is used here?