7
$\begingroup$

I'm curious about what is known about the distribution of the function $\frac{1}{\pi}\operatorname{Arg} \zeta(1/2+2\pi i t) \in (-1,1]$, on a linear or logarithmic scale, where $\operatorname{Arg}$ is the principal value of the argument. In particular, does it have mean value $0$? In other words, is it true that $$\lim_{x \to \infty} \frac{1}{x}\int_0^x \frac{1}{\pi}\operatorname{Arg} \zeta(1/2+2\pi i t) \, dt = 0?$$ If not, then does the function have a mean value of some sort, or is it equidistributed in some sense? Maybe some of its higher order moments exist?

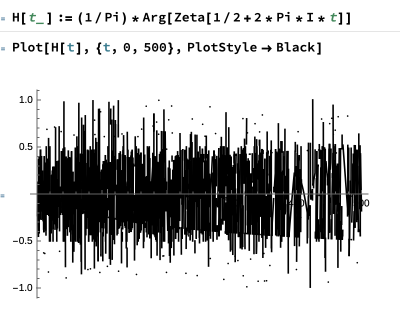
Here is a Mathematica plot of the function on $[0,500]$.

enter image description here

EDIT: The problem is motivated by my answer to my own question at Riemann-Von Mangoldt formula

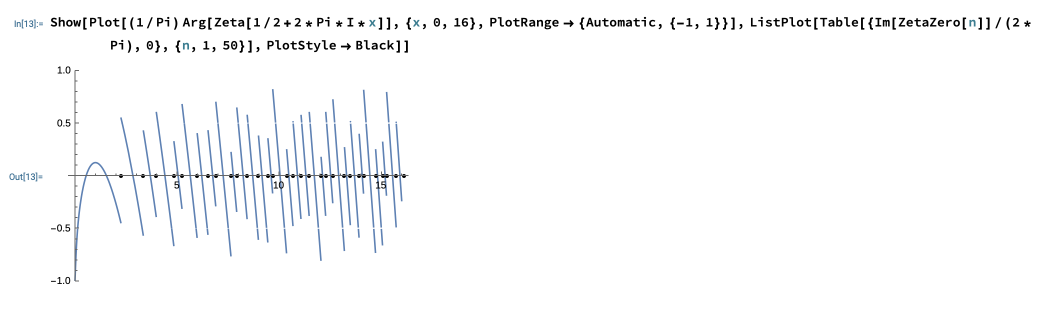
The reason you want the principal value of arg is that you want the function to have discontinuities at the imaginary parts of the nontrivial zeros of $\zeta(s)$ normalized by $\frac{1}{2\pi}$. Here is a graph of the function $\frac{1}{\pi}\operatorname{Arg} \zeta(1/2+2\pi i t)$ on $[0,16]$ along with a plot of the said normalized imaginary parts of the zeros. (I don't know how to make the image larger.)

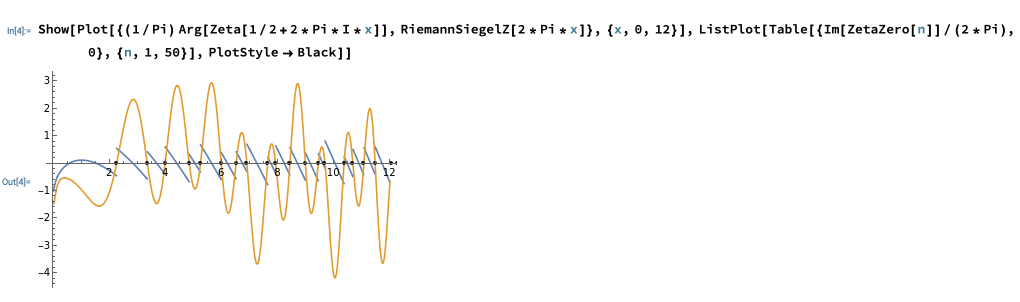
enter image description here

SECOND EDIT: @Lucia mentions a possible connection with the Riemann-Siegel or Hardy $Z$ function, but I don't quite follow how it's related. Here's another graph with the $Z$ function in the same image but on the interval $[0,12]$. How does one relate the mean value of the arg of zeta function with the $Z$ function? enter image description here

Improve this question
$\endgroup$
16
  • 1
    $\begingroup$ @StevenStadnicki It's probably motivated by the fact that this is the normalization that appears in the zero counting function for the Riemann zeta function. It (i.e., the normalized argument) is usually called $S(T)$ in the literature. See, for example, aimath.org/WWN/rh/articles/html/71a Although the way that $S(T)$ is usually defined is via continuous variation starting at $s = 2$, going vertically upward until it reaches $2 + it$ and then going horizontally leftward until it reaches $1/2+it$, which I think is different from the "principle" branch of the argument. $\endgroup$
    – Anurag Sahay
    Commented Nov 3, 2021 at 22:07
  • 1
    $\begingroup$ I don't believe this is known. The functional equation tells you the argument modulo $\pi$ instead of $2\pi$, so I think your question is really asking about the intervals in which the Hardy $Z$-function is positive or negative. This is not well understood -- see this recent paper of Gonek and Ivic: arxiv.org/abs/1604.00517. $\endgroup$
    – Lucia
    Commented Nov 4, 2021 at 4:47
  • 1
    $\begingroup$ The sign of the Z-function together with the functional equation allow you to determine the argument in your sense. I think your problem is exactly the one considered by Gonek and Ivic (but I didn't work this out carefully). $\endgroup$
    – Lucia
    Commented Nov 4, 2021 at 13:43
  • 1
    $\begingroup$ Maybe it follows from Selberg's theorem $\frac{1}{\sqrt{\frac{1}{2} \log \log T}} \log |\zeta(1/2+it)|\xrightarrow{d} N(0,1)$? In your answer to your previous question where did you get that formula for N(T)? ($N(2 \pi T) =1+ \frac{1}{\pi} \theta(2\pi T) + \frac{1}{\pi}\operatorname{Arg}\zeta\left(\frac{1}{2}+2\pi i T\right)$) $\endgroup$
    – Dabed
    Commented Nov 5, 2021 at 6:53
  • 1
    $\begingroup$ @Dabed. I edited the answer to my previous question to answer yours, and provided a slight correction. (The formula does not hold at the points of discontinuity--you have to do a right-hand limit.) I will have to study up on Selberg's theorem--this is the first I've heard of it. $\endgroup$
    – Jesse Elliott
    Commented Nov 5, 2021 at 23:12

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .