The lower bound of number of vertices covered by maximum matching in $3$-regular graph

Question

Let $G$ be a $3$-regular graph (cubic graph) with order $n$.
From here, the lower bound of # of vertices covered by maximum matching in $G$ is $\frac{3}{4}n$.
And from here, the lower bound is $\frac{7}{8}n$ (actually $\lceil \frac{7}{8}n \rceil$).
I understood the proof, but I am curious about the sharpness of these bounds, especially $2\alpha'(G) \geq \frac{7}{8}n$.
I tried to find better bound or find an algorithm to construct $G$ with $2\alpha'(G)=\frac{7}{8}n$, but I failed both strategy.
Would you help me?

Answer 1

The bound $\frac{7}{8}n$ is tight. The example shown below (image courtesy of David Eppstein) is a well-known cubic (planar) graph that has no perfect matching.

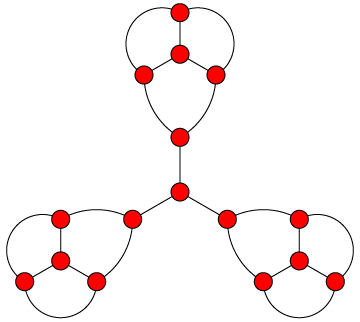
_{(source: uci.edu)}

This graph has $16$ vertices, so the maximum number of vertices covered by a maximum matching is $14$. Note that $\frac{14}{16}=\frac{7}{8}$, and we can get larger examples by taking disjoint unions of this graph.