$\newcommand{\R}{\mathbb{R}}\newcommand{\be}{\beta}\newcommand{\al}{\alpha}\newcommand{\ga}{\gamma}$Let $k_1:=h$, $k_2:=k$,
\begin{equation*}
g_i(t):=2+\be t-k_i(t), \tag{1}
\end{equation*}
$B_t:=-W_t$,
\begin{equation*}
\tau_i:=\inf\{t\ge0\colon B_t\ge g_i(t)\}, \tag{2}
\end{equation*}
$i\in\{1,2\}$.
It is assumed that $g_1$ and $g_2$ are right-continuous (r.c.), $g_1\le g_2$, and
\begin{equation*}
g_1(u)<g_2(u) \tag{3}
\end{equation*}
for some real $u\ge0$. It is also assumed that $\be>0$ and
\begin{equation}
0\le k_1,k_2\le1. \tag{3.5}
\end{equation}
We want to show that then $P(\tau_1=\infty)<P(\tau_2=\infty)$, that is,
\begin{equation*}
P(\tau_1<\infty=\tau_2)\overset{\text{(?)}}>0. \tag{4}
\end{equation*}
Since $g_1$ and $g_2$ are r.c., (3) implies that for some $w\in(u,\infty)$ and some real $a,b$ we have
\begin{equation}
g_1<a<b<g_2\text{ on the interval }[u,w]. \tag{5}
\end{equation}
Take any
\begin{equation}
v\in(u,w). \tag{6}
\end{equation}
Consider the events
\begin{equation}
\begin{aligned}
A&:=\{|B_t|<1\ \forall t\in[0,u]\}, \\
B&:=A\cap\{\tau_1\le v\}, \\
C&:=B\cap\{B_t<h_2(t)\ \forall t\in[\tau_1,w]\}, \\
D&:=C\cap\{B_t<1+\be t\ \forall t\ge w\},
\end{aligned}
\tag{7}
\end{equation}
where
\begin{equation}
h_2(t):=b-c(t-\tau_1),\quad c:=\frac b{w-v}. \tag{8}
\end{equation}
For $\al,\ga,T$ in $[0,\infty]$ and $L\in\R$, consider the probabilities
\begin{equation}
\begin{aligned}
p_{\al,\ga,L,T}&:=P\big(-\al+Lt<B_t<\ga+Lt\ \forall t\in(0,T)\big), \\
q_{\ga,L,T}&:=p_{\infty,\ga,L,T}=P\big(B_t<\ga+Lt\ \forall t\in(0,T)\big), \\
q_{\ga,L}&:=q_{\ga,L,\infty}=P\big(B_t<\ga+Lt\ \forall t\in(0,\infty)\big).
\end{aligned}
\tag{9}
\end{equation}
Then
\begin{equation}
\begin{aligned}
p_{\al,\ga,L,T}>0&\text{ if }\al>0,\ga>0,T<\infty, \\
q_{\ga,L,T}\in(0,1)&\text{ if }\ga>0,T<\infty, \\
q_{\ga,L}>0&\text{ if }\ga>0,L>0.
\end{aligned}
\tag{10}
\end{equation}
So,
\begin{equation}
P(A)=p_{1,1,0,u}>0. \tag{11}
\end{equation}
By (1) and (3.5),
\begin{equation*}
g_i(t)\ge1+\be t\ge1 \tag{12}
\end{equation*}
for $t\ge0$.
So, by (5),
\begin{equation}
b>1>0, \tag{13}
\end{equation}
and on the event $A$ we have $\tau_1>u$ and hence,
\begin{equation}
P(B)=P(A)P(B|A)\ge P(A)(1-q_{1+b,0,v-u})>0, \tag{14}
\end{equation}
by (5), (10), and (11).
Next, by (8), (13), and (6), $c>0$ and hence $h_2(t)\le b<g_2(t)$ on the event $B$ for all $t\in[\tau_1,w]$, by (5). So,
\begin{equation}
\tau_2>w\text{ on the event $C$.} \tag{15}
\end{equation}
Moreover,
\begin{equation}
P(C)=P(B)P(C|B)\ge P(B)q_{b-a,-c,w-u}>0, \tag{16}
\end{equation}
by (5), (10), and (14).
Further, on the event $C$ we have $B_w<h_2(w)\le b-c(w-v)=0$, by (8), (7), and the condition $c>0$. Therefore,
\begin{equation}
P(D)=P(C)P(D|C)\ge P(C)q_{1,\be}>0, \tag{17}
\end{equation}
by (10) and (16).
Also, by (15) and (12), we have $\tau_1<\infty=\tau_2$ on the event $D$.
Thus,
\begin{equation*}
P(\tau_1<\infty=\tau_2)\ge P(D)>0,
\end{equation*}
which proves (4). $\quad\Box$
(The condition for $k$ and $h$ to be nondecreasing was not needed or used in this proof.)
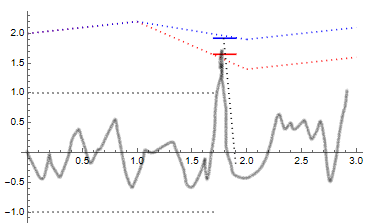
The picture below shows my crude rendering of a path (gray) of $(B_t)$ in the event $D$; plus graphs of $g_1$ (dotted, red) and $g_2$ (dotted, blue) corresponding to $\be=0.2$, $h_1(t)=\min(1,\max(0,t-1))$, and $h_2(t)=h_1(t)/2$; horizontal segments at levels $a=1.65$ (red) and $b=1.92$ (blue) over the interval $[u,w]=[1.7,1.9]$;
plus two horizontal segments (dotted, black) at levels $-1$ and $1$ over the interval $[0,u]=[0,1.7]$; and the graph of $h_2$ (dotted, black) with slope $-c=-19.2$.