Symmetric algebra over a realization of Coxeter System is a dgg algebra

Question

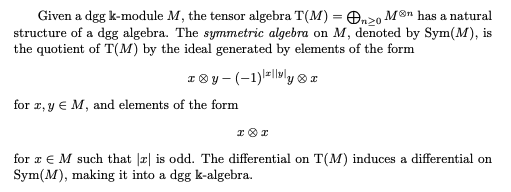
I have been reading a paper of Achar, Makisumi, Riche and Williamson. In the chapter 3, the authors talk us of bigraded modules and dgg modules and I'm stuck here.

Let $(W, S)$ be a Coxeter system, let $\mathbb{k}$ be a commutative ring, and let $\mathfrak{h}=(V,\{\alpha_{s}^{\vee}\}_{s\in S},\{\alpha_{s}\}_{s\in S})$ be a realization of $(W,S)$ over $\mathbb{k}$. Let's consider the dgg algebras $$\begin{align*} R&=Sym(V^*(-2)), & R^{\vee}&=Sym(V\langle 2\rangle). \\ \Lambda&=Sym(V^*(-2)[1]), & \Lambda^{\vee}&=Sym(V^*\langle-2\rangle[1]). \end{align*} $$ I do not understand the following: First, why $V$ and $V^*$ is a dgg $\mathbb{k}$-module? Because the shift are on this free $\mathbb{k}$-modules. Second I cannot see why $\Lambda$ is isomorphic to antisymmetric algebra.

Here is the link for the paper: https://arxiv.org/abs/1703.05843

Answer 1

For all these modules, the differential is trivial, so a dgg module just means a bigraded module (which $V$ and $V^*$ are with all elements in the same degree).

The module $\Lambda$ and $\Lambda^{\vee}$ are exterior algebras because of the definition just above in the paper:

Since the elements of $V$ and $V^{\vee}$ are in odd degree, they anti-commute.