Say that a tile $T$ that alone can tile the plane—a monohedral tile—is rigid if it is not the case that $T$ can be slightly deformed to $T'$ so that:
- $T'$ can also tile the plane
- $T'$ is arbitrarily close to $T$, say, according to Hausdorff distance.
- The tiling by $T'$ is "combinatorially equivalent."
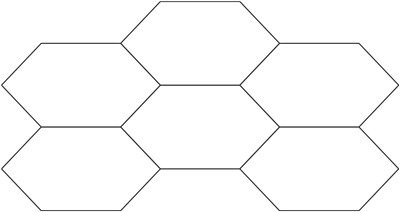
For example, the familiar regular hexagon tiling is not rigid, because it can be "compressed" vertically:
Rigid tilers are "brittle" in that even a slight deformation changes their tiling properties. Since all triangles and all quadrilaterals are monohedral tilers, it is not difficult to claim that no $n\in\{3,4\}$-sided polygons are rigid tilers.
Questions:
- Q1 Has this notion of a "rigid tiler" been explored?
- Q2 Which of the $15$ pentagonal monohedral tilers are rigid?
Clearly the pentagonal cmm (2*22) tiler is not rigid, because the pentagon tile is simply a hexagon split vertically: