6
$\begingroup$

A linklessly embeddable graph is a graph which can be embedded into $\Bbb R^3$ so that no two of its cycles are linked. For example, the Petersen graph is not such a graph.

Now, I can think of another type of "linkedness" that is not already addressed by this notion, namely, whether an embedding contain a Borromean rings configuration. By that I mean three cycles of the graph, no two of which are linked, but all three can still not be "entangled into three separate cycles" (see the image from Wikipedia).

$\qquad\qquad$

Question: if a graph is linklessly embeddable, does it also have a linkless embedding without a Borromean rings configuration?

Or the other way around, are there linklessly embeddable graphs that cannot be linklessly embedded without Borromean rings?

Some thought

Maybe one can show that whenever a graph cannot be embedded without Borromean rings, then the following two (black) paths must be present. This would then already imply the presence of a link:

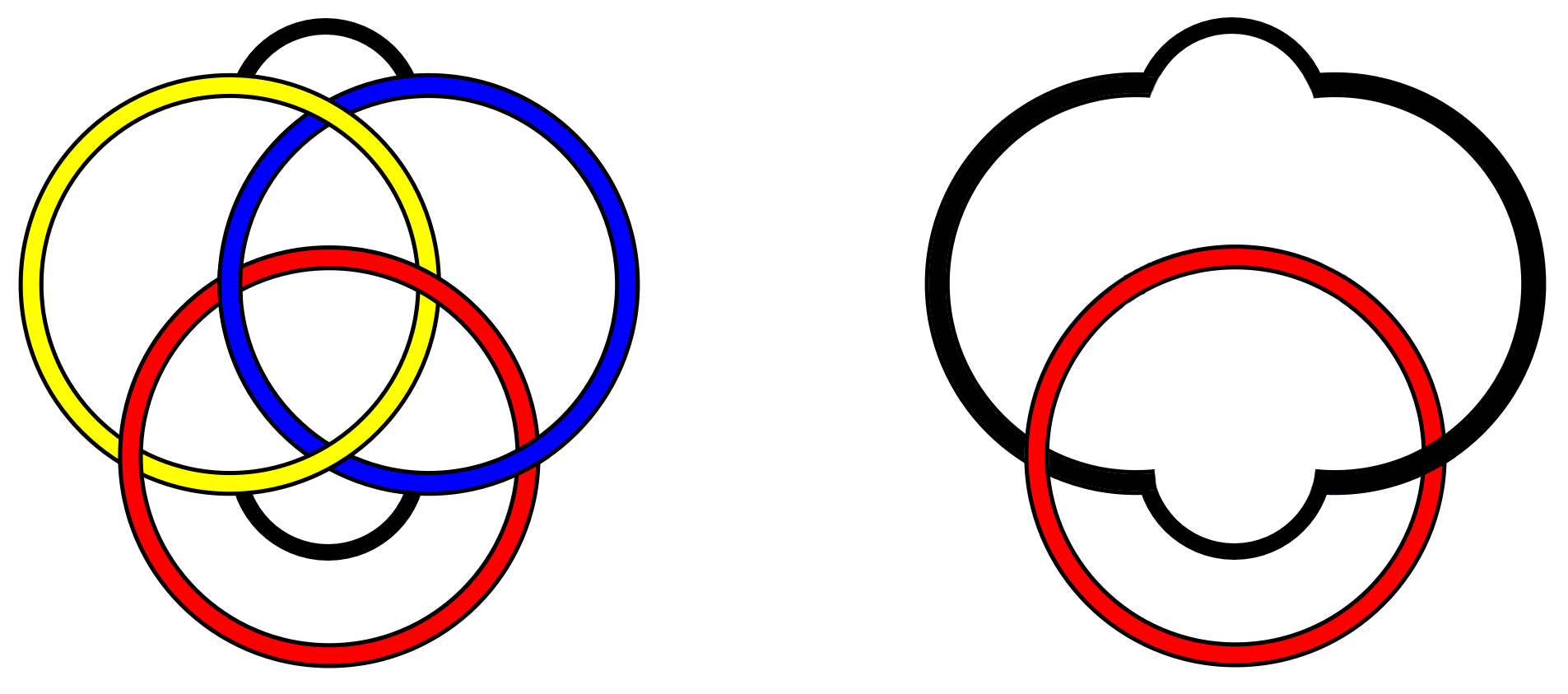
This does not address the possibility that a graph might be linklessly embeddable, and embeddable without Borromean rings, but not both at the same time.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

Isn't it that linklessly and flatly embeddable are the same family, and that a flat embedding can not contain a Borromean ring?

upd - clarification: From the wiki article on linkless embeddings: "A flat embedding is an embedding with the property that every cycle is the boundary of a topological disk whose interior is disjoint from the graph." As far as I remember (and the wikipedia seems to confirm), every linklessly embeddable graph has a flat embedding (every flat embedding is trivially linkless). Finally, that a flat embedding can't contain a Borromean ring seems trivial to me from the above definition of a flat embedding (still can be wrong)

Improve this answer
$\endgroup$
3
  • $\begingroup$ Could you elaborate what you mean by flatly embeddable and what it has to do with linkless embeddable and Borromean rings? $\endgroup$
    – M. Winter
    Commented Aug 23, 2021 at 12:07
  • 1
    $\begingroup$ From the wiki article on linkless embeddings: "A flat embedding is an embedding with the property that every cycle is the boundary of a topological disk whose interior is disjoint from the graph." As far as I remember (and the wikipedia seems to confirm), every linklessly embeddable graph has a flat embedding (every flat embedding is trivially linkless). Finally, that a flat embedding can't contain a Borromean ring seems trivial to me from the above definition of a flat embedding (still can be wrong) $\endgroup$
    – Alexander Igamberdiev
    Commented Aug 23, 2021 at 12:09
  • $\begingroup$ This is a helpful comment. And the claim seems to go back to the paper "Sachs' linkless embedding conjecture" by Robertson, Seymour and Thomas. They call it a "panelled embedding". If you could work your comment into your post to make it a full answer I would be happy to accept! $\endgroup$
    – M. Winter
    Commented Aug 23, 2021 at 12:15

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .