I wonder if there is something like a general "prime component distribution pattern" of "the general natural number" $n$?
Using the following notation for the prime factorization $n = p_1^{\alpha_1}p_2^{\alpha_2}\dots p_{k-1}^{\alpha_{k-1}} p_k^{\alpha_k}$ with $k={\pi(n)}$ thus going through all primes from $2$ up to $p_{\pi(n)}$ and setting $\alpha_j = 0$ for all $p_j\nmid n$, I define a distribution density function
$\delta_n(x)=\begin{cases} \alpha_i \frac{\pi{(n)}}{\Omega{(n)}} & (i-1)/\pi(n) < x\le i/\pi(n), \forall i\in\{1,...,k\} \\ 0 & else \end{cases}$
Two examples $\delta_4$ and $\delta_{21}$ are shown below
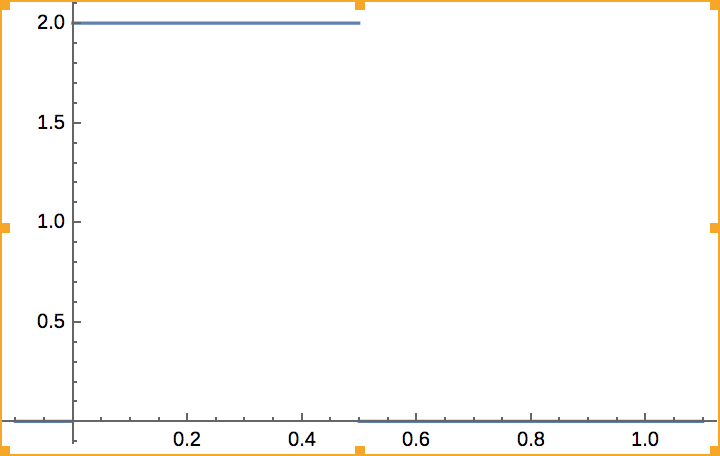 $\delta_4$(x)" />
$\delta_4$(x)" />
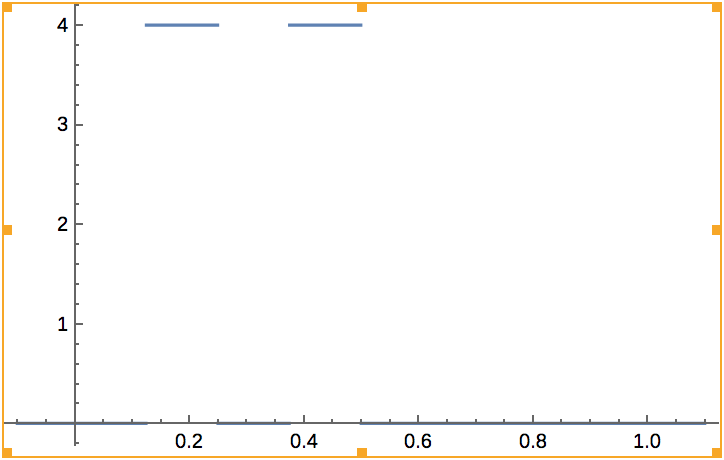 $\delta_{21}(x)$" />
$\delta_{21}(x)$" />
The functions are normalized according to
$\int_{0}^{1}{\delta_n (x) \mathrm{d}x} = 1$
Now the question is if this limit
$$ \Delta_{\infty}(x) = \lim_{n\to\infty} \frac{1}{n}\sum_{k=2}^{n+1} \delta_k(x) \tag{1}$$
exists and in case how that might look like. I have calculated the average for $n = 250$ (my online Mathematica subscription doesn't allow for higher numbers) and it looks relatively interesting:
The increasing domain to the right is obviously due to the primes, the next peak to the left appears to be due to even numbers and so on.
I would be grateful for any comments, ideas on the primary question (first sentence in the post) and if this could be an appropriate way to adress it. Most of all if convergence of $(1)$ is to be expected or not.

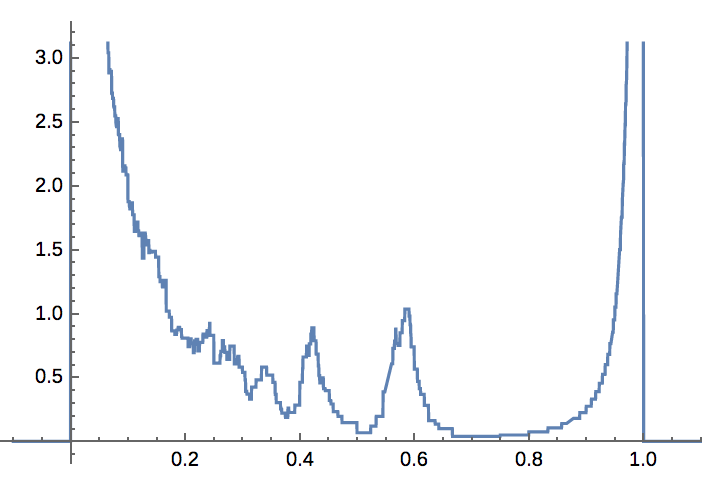 $\Delta_{250}(x)$" />
$\Delta_{250}(x)$" />
$\begin{cases} 1 & x=0 \\ 0 & x\neq 0 \end{cases}$lets you do cases. $\endgroup$