6
$\begingroup$

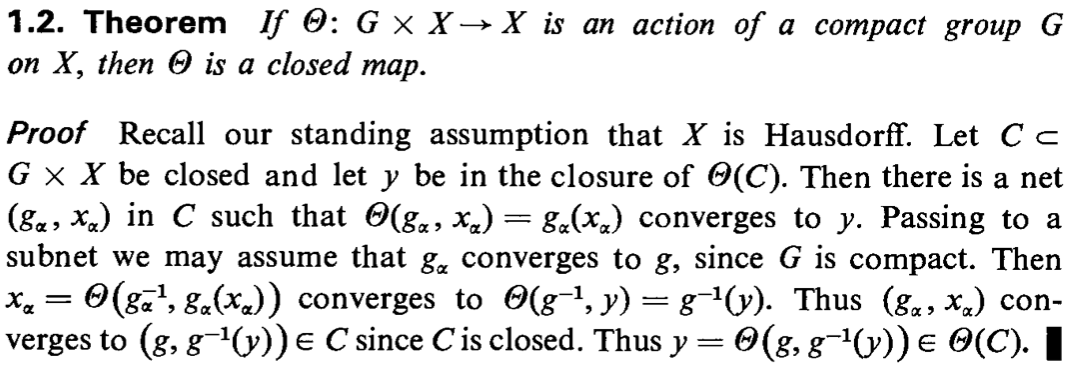
I am reading Bredon's Introduction to compact transformation groups, and came across the following result and proof on page 34:

enter image description here

Even though he writes "Recall our standing assumption that $X$ is Hausdorff," I cannot see where any properties of Hausdorffness of $X$ are used in this proof. I suspect it would be used to ensure that convergent nets in $X$ converge uniquely, but I do not see why this fact would be needed for this proof.

Is it true that the continuous action of a compact topological group on a topological space $X$ is always closed, regardless of whether $X$ is Hausdorff? If not, then where in the above proof is Hausdorffness used?

Improve this question
$\endgroup$
4
  • $\begingroup$ There's also the question of whether one assumes a topological group to be $T_0$, and hence Hausdorff, by default. $\endgroup$
    – David Roberts ♦
    Commented Jul 13, 2021 at 7:24
  • $\begingroup$ Compact/ Locally compact groups are usually assumed Hausdorff by default, even when topological groups are not. $\endgroup$
    – YCor
    Commented Jul 13, 2021 at 8:03
  • $\begingroup$ Hausdorffness of $X$ doesn't seem to be used at any point in the proof. $\endgroup$
    – YCor
    Commented Jul 13, 2021 at 8:05
  • $\begingroup$ The only critical point seems to be the existence of a subnet, but this is well known, see f.i. Kelley, General Topology. $\endgroup$
    – Dieter Kadelka
    Commented Jul 13, 2021 at 9:16

2 Answers 2

Reset to default
15
$\begingroup$

Yes, it is true, and the following argument will surely convince you so. Recall that a topological space $K$ is compact iff it is universally closed: for every topological space $X$, the coordinate projection map $X\times K \to X$ is closed. Now, use the obvious fact that $G\times X\to G\times X$, $(g,x)\mapsto (g,gx)$, is a homoemorphism and realize the action map $G\times X \to X$ as a composition of this homeo with the coordinate projection map.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks for the great answer. It appears we do not even need $G$ to be Hausdorff (a question raised by David Roberts in the comments to my question). $\endgroup$
    – Ben
    Commented Jul 13, 2021 at 12:30
  • 1
    $\begingroup$ @Ben, right, but this is not a big deal, really. If $G$ is not Hausdorff then, denoting $G_1=\overline{\{1\}}$, we have that $G_1$ is a closed normal subgroup of $G$ on which the topology is trivial, thus each $G_1$ orbit in $X$ is inseparable. Then the action map $G\times X \to X$ fibers naturally over the action map of $G/G_1$ on $X/G_1$, thus everything reduces to the separable case. That is, the theory of dynamical systems reduces easily to the Hausdorff world. $\endgroup$
    – Uri Bader
    Commented Jul 13, 2021 at 16:34
4
$\begingroup$

Here is an elementary proof:

Let $C\subseteq G\times X$ be closed. Note that $x_0\notin \Theta(C)$ if and only if $\{(g,g^{-1}x_0)\mid g\in G\}\cap C = \emptyset$ which is if and only if $G\times \{(e,x_0)\}\subseteq \Phi^{-1}((G\times X)\setminus C)$ where $\Phi: (g,(h,x))\mapsto (hg^{-1}, gx)$. Now, let $x_0\notin \Theta(C)$. By our observation, $G\times \{(e,x_0)\}\subseteq \Phi^{-1}((G\times X)\setminus C)$ and since $C$ is closed, $\Phi^{-1}((G\times X)\setminus C)$ is a neighborhood of $G\times \{(e,x_0)\}$. Hence, (by the tube lemma), we can find neighborhoods $U$ of $e$ and $V$ of $x_0$ such that $G\times (U\times V) \subseteq \Phi^{-1}((G\times X)\setminus C)$. It is easy to see that $\Theta(U\times V)$ is a neighborhood of $x_0$ which does not intersect $\Theta(C)$.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .