7
$\begingroup$

The Turaev-Viro-Barrett-Westbury invariant of a closed oriented topological $3$-manifold $M$ for a spherical fusion category $\mathcal{C}$ is a number denoted $|M|_{\mathcal{C}}$ computed from (but independent of the choice of) a triangulation of $M$ and $\mathcal{C}$-data labelings.

See the book Turaev-Virelizier (2017) on Section 13.1, and the papers Turaev-Viro (1991) and Barrett-Westbury (1996).

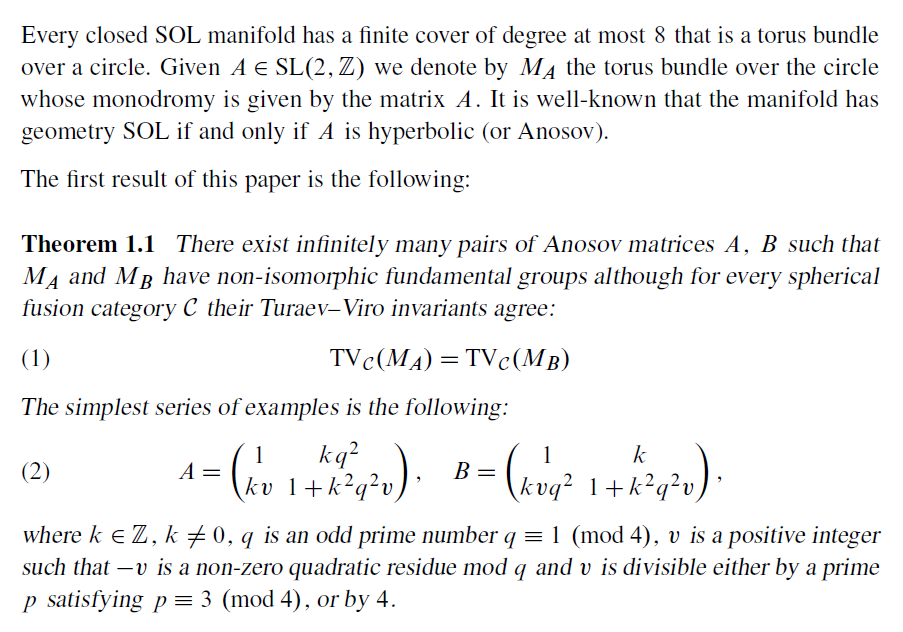
Question: Are there non-homeomorphic closed oriented $3$-manifolds $M$ and $N$ such that $|M|_{\mathcal{C}} = |N|_{\mathcal{C}}$, for all spherical fusion category $\mathcal{C}$?

This answer by Meng Cheng provides a formula for $|M|_{\mathcal{C}}$ with $M=L(p,q)$ a Lens space and $\mathcal{C}=Vec_{G,\omega}$ a pointed fusion category, and shows that it distinguishes $L(7,1)$ from $L(7,2)$, for a good choice of $G$ and $\omega$.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
9
$\begingroup$

I asked Alexis Virelizier (coauthor of the book mentioned above) by e-mail. Here is his answer (reproduced with his authorisation):

The answer is yes. See Theorem 1.1 (page 2291) in the following paper by Funar: https://www-fourier.ujf-grenoble.fr/~funar/2013geomtopol.pdf

enter image description here

Improve this answer
$\endgroup$
4
  • $\begingroup$ That's interesting. Is it the case that two 3-manifolds have all their TV invariants isomorphic if and only if their fundamental groups have the same finite quotients? $\endgroup$
    – HJRW
    Commented Jul 9, 2021 at 15:31
  • 1
    $\begingroup$ @HJRW The lens spaces $L(7,1)$ and $L(7,2)$ have the same fundamental group $C_7$, but different TV invariants (see this answer). $\endgroup$
    – Sebastien Palcoux
    Commented Jul 9, 2021 at 20:34
  • 1
    $\begingroup$ I notice that Funar actually discusses this question in Remark 1.4 of his paper. (See also the sentence immediately preceding the remark: "We don’t know if the $SU(2)$ Turaev--Viro invariants alone determine already the pro-finite completion of the fundamental group.") It seems worth mentioning that Yi Liu has recently made dramatic progress on the question of profinite rigidity for 3-manifold groups.... (cont'd) $\endgroup$
    – HJRW
    Commented Jul 10, 2021 at 9:22
  • $\begingroup$ See especially: arxiv.org/abs/1906.03602, arxiv.org/abs/2011.09412, arxiv.org/abs/2105.01022 . $\endgroup$
    – HJRW
    Commented Jul 10, 2021 at 9:25

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .