Long exact sequence of cohomology from 2-groups

Question

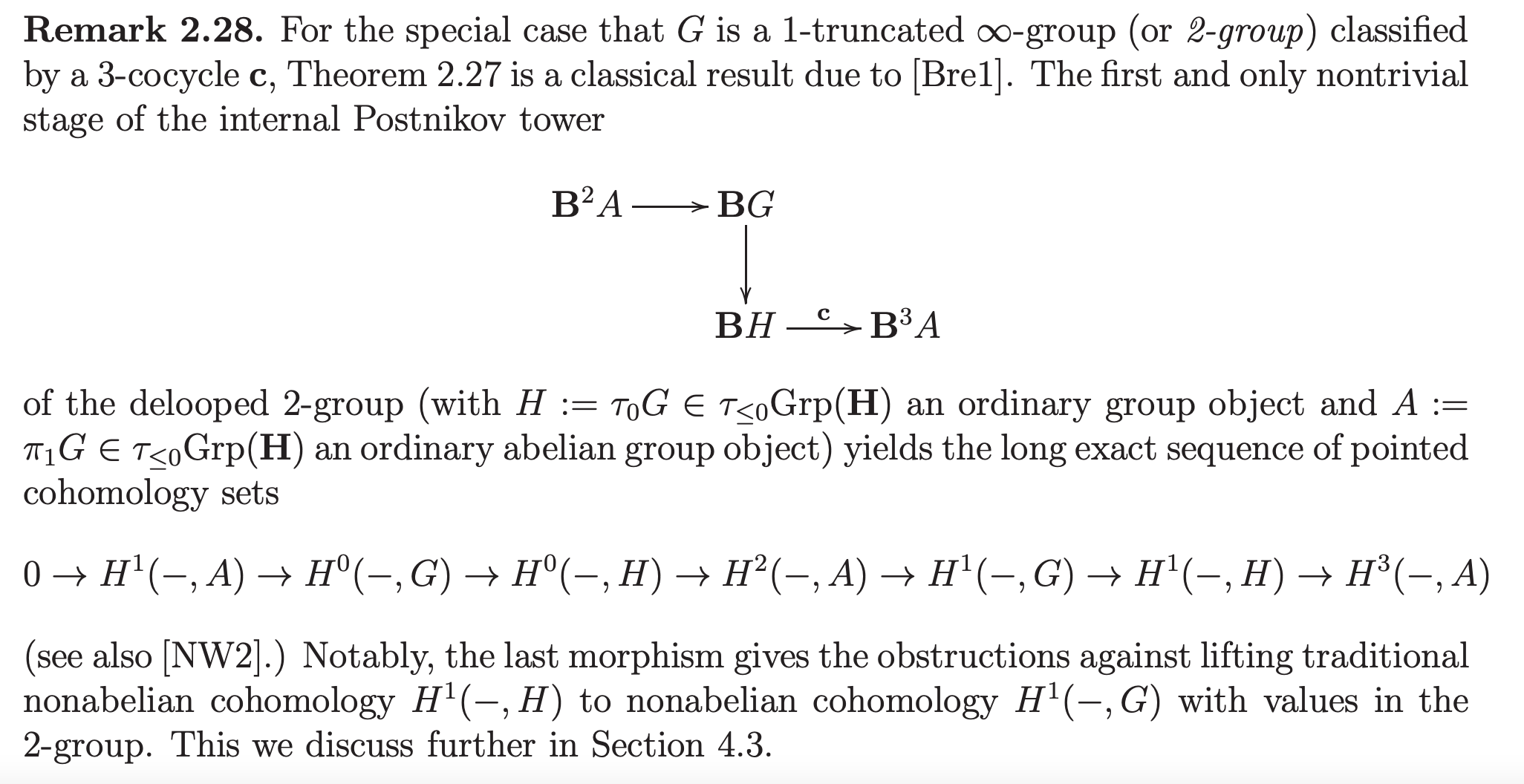
I am trying to understand the following from Principal Infinity Bundles - General by Nikolaus, Schreiber and Stevenson.

So following the reference there to Nikolaus-Waldorf tells us that given any (smoothly separable) Lie $2$-group (let's work in the model of crossed modules for convenience) $[d: H \to G]$, the following is a fiber sequence of Lie $2$-groups $$[\ker d \to 1] \to [H \to G] \to [1 \to \text{coker }d]$$ What this means precisely is explained in more detail in Lemma 2.2 of this paper. In the notation of the problem, this corresponds to $$BA \to G \to H$$ being a fiber sequence of $2$-groups, where $H$ is a group discretely embedded as a $2$-group and $A$ is an abelian group that is being delooped to a $2$-group. Now since $B$ is a right adjoint, it preserves this fiber sequence, and that gives us that $$B^2 A \to BG \to BH$$ is a fiber sequence.

So here are my questions:

• What is this map $c$? In Definition 4.30 of the same paper they give a definition of what a central extension of infinity groups should be involving this $c$, but I don't know how to construct it in this example.
• In what ways can I present this map? Namely how would I present $B^3 A$? Can I use simplicial groups?
• Why is this the internal and only nontrivial stage of the postnikov tower?

Answer 1

$c$ is your crossed module, or 2-group, in a sense. Anything more concrete will depend on a choice of a cocycle description of the pointed set $[BH,B^3A]$.

For example, $[BH,B^3A]$ classifies central 2-group extensions $BA \to G \to H$ up to weak equivalence. Hence, one could regard such extensions as the cocycles, and then $c=G$.

A probably more concrete cocycle model is given by the smooth group cohomology $H^3_{sm}(H,A)$. By this I mean a smooth version of "Segal-Mitchison cohomology", like considered by Brylinksi. Recent references are:

• Schommer-Pries, Christopher J., Central extensions of smooth 2-groups and a finite-dimensional string 2-group, Geom. Topol. 15, No. 2, 609-676 (2011). ZBL1216.22005.

• Wagemann, Friedrich; Wockel, Christoph, A cocycle model for topological and Lie group cohomology, Trans. Am. Math. Soc. 367, No. 3, 1871-1909 (2015). ZBL1308.22006.

• Blanco, Jaider; Uribe, Bernardo; Waldorf, Konrad, Pontrjagin duality on multiplicative Gerbes, preprint.

In the first reference it is described how to obtain the cocycle from the 2-group extension. As usual, this will depend on choices of open covers, sections, etc., so that one cannot expect a canonical formula for $c$.

In several specific cases, $H^3_{sm}(H,A)$ can be identified with more familiar sets. For example, if $H$ is compact and $A=U(1)$, we have $H^3_{sm}(H,A)\cong H^4(BH,\mathbb{Z})$. If $G$ is the string 2-group, then $c \in H^4(BSpin,\mathbb{Z})=\mathbb{Z}$ is a generator. If $G$ is the T-duality 2-group, then $c\in H^4(B\mathbb{T}^{2n},\mathbb{Z})$ is $c=\sum_{i=1}^n c_i \cup c_{i+n}$, where $c_i \in H^2(BS^1,\mathbb{Z})$ is the first Chern class of the $i$th factor. Some further examples have been computed in the above-mentioned preprint in Sections 5.4 - 5.6.