[This question followed up from a question on Math StackExchange.]
Writing Robin's inequality for the Riemann hypothesis (RH) as $$\frac{\sigma(n)}{n \ln\ln n} < e^\gamma \;,$$ we can take logarithms and introduce a function $$s(n) = \ln \frac{\sigma(n)}{n \ln\ln n}$$ such that the bound becomes $s(n) < \gamma$. This is convenient, because if we know the prime factorisation $n = \prod_{q} q^{k_q}$ of a candidate number, we can rather easily compute $$s(n) = \sum_{q} \ln \frac{q^{k_q+1} - 1}{q - 1} - \sum_{q} k_q \ln q - \ln\ln \sum_{q} k_q \ln q \;,$$ without too much numerical trouble.
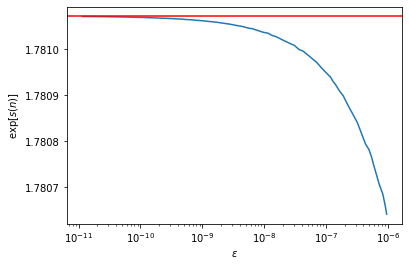
If the RH is false, the inequality will fail to hold for a colossally abundant number. Theorem 10 of Alaoglu & Erdős (1944) gives a simple enumeration of the prime exponents of the colossally abundant number associated with a particular $\epsilon > 0$, $$k_q(\epsilon) = \left\lfloor \frac{\ln \frac{q^{1+\epsilon}-1}{q^{\epsilon}-1}}{\ln q}\right\rfloor - 1 \;.$$ Since all we need to compute the function $s(n)$ are a large list of primes $q$ and their exponents $k_q$, we can pick any $\epsilon > 0$, for example at random, compute the complete list of nonzero $k_q(\epsilon)$, and obtain $s(n)$ rather straightforwardly. The result of a random draw as function of $\epsilon$ is shown here:
Interestingly, we seem able to make the following observation:
For $\epsilon < 0.01$, the function $s(n)$ is monotonically increasing as $\epsilon$ approaches zero.
Is this true?
I think it is pretty straightforward that $s(n)$ is piecewise constant as a function of $\epsilon$. If $s(n)$ changes because the exponent $k_q$ increases (is it always just one of the $k_q$?), the change is $$\delta s = \ln \frac{q^{k_q+2} - 1}{q^{k_q+2} - q} - \ln\ln\ln nq + \ln\ln\ln n \;.$$ It's easy to find the largest $q$ given $\epsilon$, but are there bounds for $k_q$ and $n$ to show $\delta s > 0$?
If the observation was true, it would mean that the RH can be disproved with the limit $\epsilon \to 0$ of $s(n)$ alone (which is of course still a very hard problem). This seems a very analytical problem however, and makes me wonder how and where the structure of the primes matters in this.
Edit:
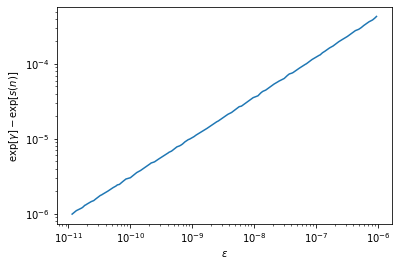
Let me ask a different question: We do have that $$ \limsup_{n \to \infty}\frac{\sigma(n)}{n \ln \ln n} = e^\gamma \;.$$ Does it follow that $\limsup_{\epsilon \to 0} s(n) = \gamma$? Is the conjecture therefore equivalent to the Riemann hypothesis?