An inequality for an integral transform of a function

Question

Let $$J_{f;y}(u):=3 u^3 \int_u^1\frac{dt}{t^4} \,e^{-i y t}f(t)- e^{-i u y}f(u),$$ where $y\in(0,\infty)$, $u\in(0,1)$, and $$f(t):=t+\pi (1-t) t \cot (\pi t).$$

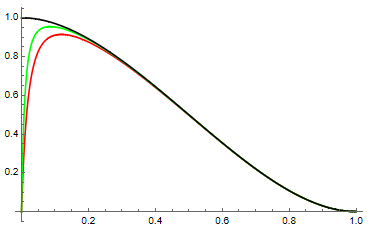
Here are the graphs of $f$ (black), $|J_{f;100}|$ (red), and $|J_{f;200}|$ (green):

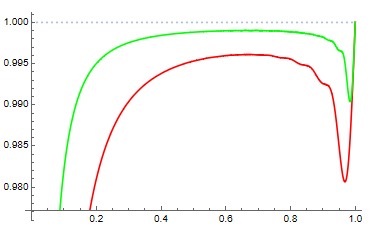
and of $1$ (dotted), $|J_{f;100}|/f$ (red), and $|J_{f;200}|/f$ (green):

(The values of $J_{f;y}(u)$ for all the graphs here were obtained by non-controlled numerical integration using Mathematica's command NIntegrate[].)

It appears that $|J_{f;y}|$ is monotonically increasing in $y>0$ to $f$ (that $|J_{f;y}(u)|\to f(u)$ for each $u\in(0,1)$ as $y\to\infty$ follows by the Riemann–Lebesgue lemma).

Similar pictures obtain if $f(t)$ is replaced by $g(t):= \dfrac{\sin (2 \pi t)}{2 \pi }+\dfrac{1}{3} (1-t) (\cos (2 \pi t)+2)$.

Conjecture: $|J_{f;y}|\le f$ on $(0,1)$ for all real $y>0$.

Question: Is this conjecture true?

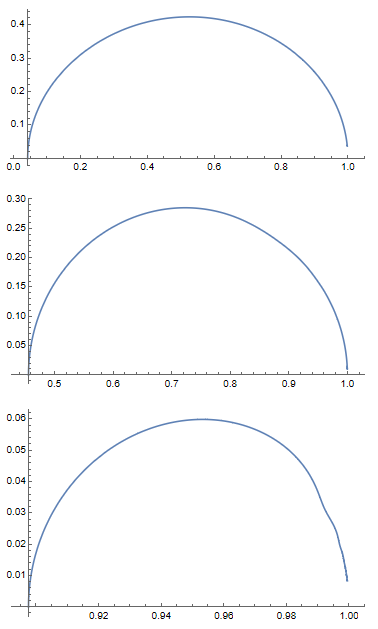
Here are also the plots $P(u_1,Y_1)$ (top), $P(u_2,Y_2)$ (middle), and $P(u_3,Y_3)$ (bottom), where $$P(u,Y):=\Big\{\frac{J_{f;y}(u)}{-e^{-i u y}f(u)}\colon0<y<Y\Big\},$$ $(u_1,Y_1):=(\frac1{10},800)$, $(u_2,Y_2):=(\frac5{10},600)$, and $(u_3,Y_3):=(\frac9{10},400)$:

Comment: The functions $f$ and $g$ are decreasing on $(0,1)$, but the conjecture will not hold in general if $f$ is replaced by an arbitrary decreasing nonnegative function $h$ such that $h(1)=0$. It would be interesting to know conditions on functions $h$ such that $|J_{h;y}|\le h$ on $(0,1)$ for all real $y>0$ -- but that would be just a cherry on the cake. It is enough for me to know if the conjecture is true as stated, for the particular function $f$.

Answer 1

It looks like the inequality is true (at least for the function with the cotangent). The proof (I hope it is correct but, please, check the details: I'm not in my top shape today) is as follows.

Claim 1: For every $y>0$, we have $\left|\int_0^y t^3e^{it}\,dt\right|\le y^3$.

Proof: One trivial bound for the LHS is $\int_0^y t^3=\frac {y^4}4\le y^3$ when $y\le 4$. On the other hand, repeated integration by parts gives $$ \int_0^y t^3e^{it}\,dt=i^{-1}y^3e^{iy}-3i^{-2}y^2e^{iy}+6i^{-3}ye^{iy}-6i^{-4}(e^{iy}-1)\, $$ so $$ LHS\le \sqrt{(y^3-6y)^2+(3y^2-6)^2}+6\,. $$ Thus, it will suffice to show that for $y\ge 4$ $$ (y^3-6y)^2+(3y^2-6)^2\le (y^3-6)^2\, $$ i.e., $-3y^4\le -12 y^3$, which, luckily, holds exactly in the range we need it.

Now we can consider a function $g(t)=g_v(t)=(t-v)^2$ for $t\le v$ and $0$ for $t\ge v$. The claim is that for all $y>0$ and $u<v$, $$ \left|\int_{u}^{+\infty} g(t)(e^{-iy(t-u)}-1)\,dt\right|\le\int_{u}^{+\infty} g(t)\,dt\,. $$ Indeed, making the change of variable $t=v-s/y$, we reduce it to $$ \left|\int_0^{y(v-u)}s^2(e^{is}e^{-iy(v-u)}-1)ds\right|\le y^3(v-u)^3/3\,, $$ which, after one integration by parts, becomes the inequality from Claim 1 with $y$ replaced by $y(v-u)$.

The immediate consequence is that if $F$ is any function on $(u,+\infty)$ that is an integral combination of $g_v$ with non-negative coefficients, then $$ \left|\int_{u}^{+\infty} F(t)(e^{-iy(t-u)}-1)\,dt\right|\le\int_{u}^{+\infty} F(t)\,dt\,. $$

In particular, any function $F$ that is supported on $(0,1]$ with $F(1)=F'(1)=0$, $F''(1-)>0$ and $F'''\le 0$ on $(0,1)$ will do.

We shall show that $F(t)=3u^3\frac{f(t)}{t^4}\chi_{(0,1]}(t)$ is like that. Then we shall have the integral part of $J_{f,y}(u)$ multiplied by $e^{iuy}$ in the disk centered at $A=3u^3\int_u^1 \frac{f(t)}{t^4}\in(0,f(u))$ of radius $A$, so it is also in the disk centered at $f(u)$ of radius $f(u)$ finishing the story (I used that $f$ is non-negative and decreasing on $(0,1]$ here; I assume you know how to prove these properties yourself).

The only non-trivial to check part is that $F'''\le 0$ on $[0,1]$. Writing $t=1-z$, we get to check that $G'''\ge 0$ on $(0,1)$ where $$ G(z)=\frac{1-\pi z\cot\pi z}{(1-z)^3}\,. $$ However, all Taylor coefficients of the numerator are non-negative (say, because of the series representation as $2\sum_{n=1}^\infty\frac{z^2}{n^2-z^2}$) and we are done.

The sine-cosine version of $f$ is a bit uglier to investigate and, to be honest, I haven't checked the third derivative property for it. If you discover that it fails but still need the result for that version, let me know and I'll think more.

Answer 2

Here is a possible approach to proving the conjecture. (Some of the following claims have not been thoroughly verified.)

Using the Maclaurin series for $\tan$, for $t\in(0,1)$ we can write \begin{equation} f(t)=\sum_{k=1}^\infty c_k f_k(t), \end{equation} where \begin{equation} f_k(t):=\left(t-\frac{1}{2}\right)^{2 k+1}-2^{-2 k} (2 k+1) \left(t-\frac{1}{2}\right)+2^{-2 k}k, \end{equation} \begin{equation} c_k:=(-1)^{k+1} (2 \pi )^{2 k} \left(\frac{\left(2^{2 k}-1\right) B_{2 k}}{(2 k)!}+\frac{\pi ^2 \left(2^{2 k+2}-1\right) B_{2 k+2}}{(2 k+2)!}\right)>0, \end{equation} and the $B_j$ is the $j$th Bernoulli number.

The pictures for the functions $f_k$ look similar to the corresponding pictures for $f$ shown in the problem statement above (in particular, $f_k>0$ on $(0,1)$ for all $k$, so that $|f_k|=f_k$). So, the conjecture seems to hold with $f_k$ in place of $f$. Since $c_k>0$ for all $k$, it remains to prove the conjecture with $f_k$ in place of $f$.

The value of this reduction from $f$ to $f_k$ seems to be that, in distinction from $f$, the $f_k$'s are polynomials. However, with this approach, we have to deal with the additional parameter $k$, which can take arbitrarily large natural values, resulting in arbitrarily large degrees of the polynomials $f_k$.