0
$\begingroup$

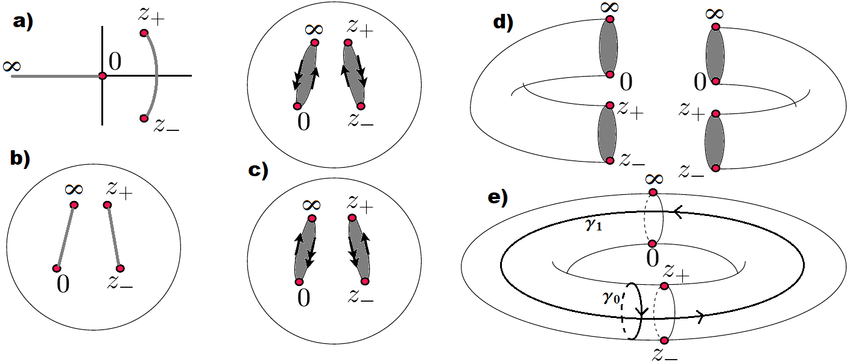
To deal with elliptic integral, we often cut riemann surface and glue them together, and gain a torus. We do this in order to avoid indeterminacy of integral, in other word, to avoid the condition that integral is not path independent.

However, on the torus, integral is not stil path independent. They differ up to two circles which generated first homology group of torus (in the picture,$r0$ and $r1$).

My question: What is the merit (necessity) of gluing to make torus and integrate on it? Indeterminancy cannot be solved by glueing process.

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ In some cases the inverse function is doubly periodic, it is a function on the torus, so the elliptic integral is a function to the torus. In many cases the inverse function is only doubly periodic up to an explicit multiplier; this leaves some indeterminacy, but a much better controllable one than without going to the torus. The projection of a torus to the Riemann sphere has four branching points so if you do not reglue you have to deal with them. With regluing, even if indeterminacy remains, the branch points are avoided. $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Jun 28, 2021 at 4:48

1 Answer 1

Reset to default
2
$\begingroup$

It is unclear what you are really asking. What does it mean to "integrate an elliptic integral"? Elliptic integrals cannot be expressed in terms of elementary functions. So we have to study them as they are. To understand their properties lifting them to a torus is very helpful, the main reason of this is that the torus has Abelian fundamental group. But the main point is that the inverse functions of some basic elliptic integrals is single valued.

Improve this answer
$\endgroup$
8
  • $\begingroup$ I'm asking the necessity of making torus. Why can't we deal with the integral on RIeman sphere? Maybe, The function we integrate is multi valued, so we avoid the multi valueness by making torus. But indeterminacy by paths is still unsolved. But the main point to make torus is to avoid multi values by making torus, right ? $\endgroup$
    – Duality
    Commented May 29, 2021 at 3:35
  • 1
    $\begingroup$ We do this because an elliptic integral is a holo- (or mero-) morphic form on the torus, not on the sphere. What you call"indeterminacy by paths" is actually a great asset, giving rise to the theorems of Abel and Jacobi. $\endgroup$
    – abx
    Commented May 29, 2021 at 7:53
  • 1
    $\begingroup$ @Nekorju: The torus is necessary to make the integrand single valued. The integral is still multi-valued, but its multi-valuedness of the simple kind: it is additive. $\endgroup$
    – Alexandre Eremenko
    Commented May 29, 2021 at 14:09
  • $\begingroup$ So basic reason is that the curve defined by the equation $X=\{y^2=(1-t^2)(1-k^2t^2)\}\subset {\mathbb CP}^2$ is a torus topologically. We can understand it in another way: the rieman surface of the function $y=\sqrt{(1-t^2)(1-k^2t^2)}$ is a torus. So although the integral of our differential form along some loop may be non-zero, function itself does not change when we follow round this loop. $\endgroup$
    – user21167
    Commented May 29, 2021 at 14:27
  • $\begingroup$ @Alexandre Eremenko Integrand is not single valued on torus? The integral depends on the paths, but the integrand itself maybe single valued ( we glued two Riemann surface to make it single valued). What is wrong ? $\endgroup$
    – Duality
    Commented May 31, 2021 at 6:46

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .