Euclidean and Minkowski Majorana spinors - inconsistency with Wikipedia Table

Question

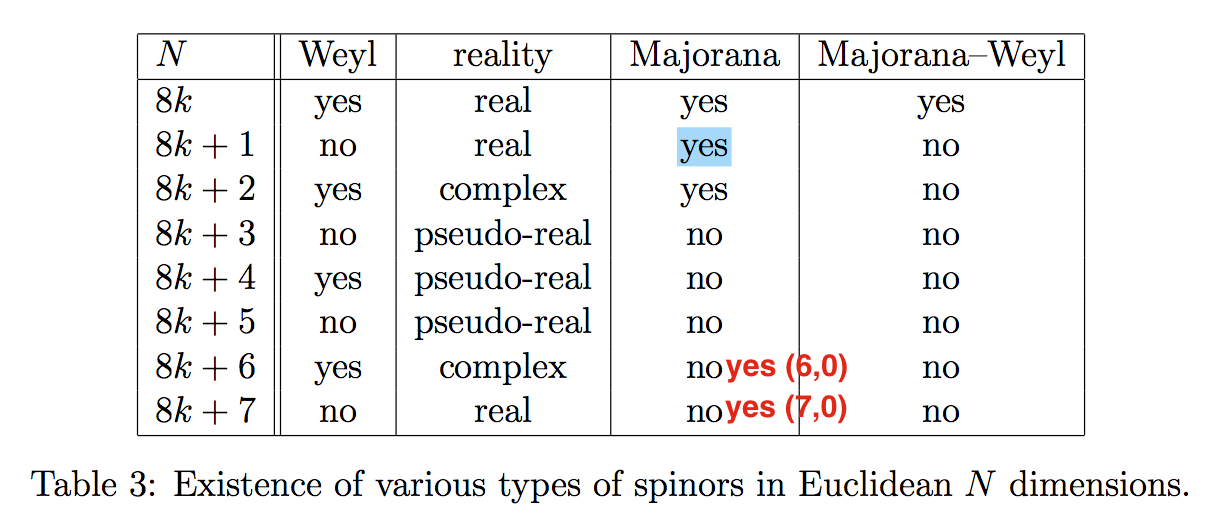
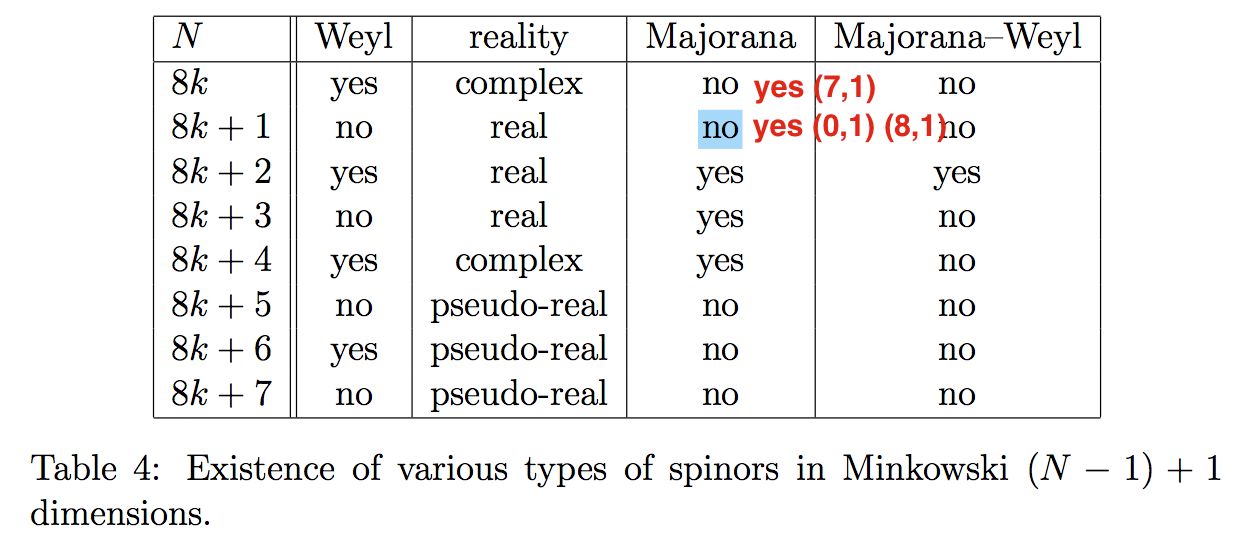
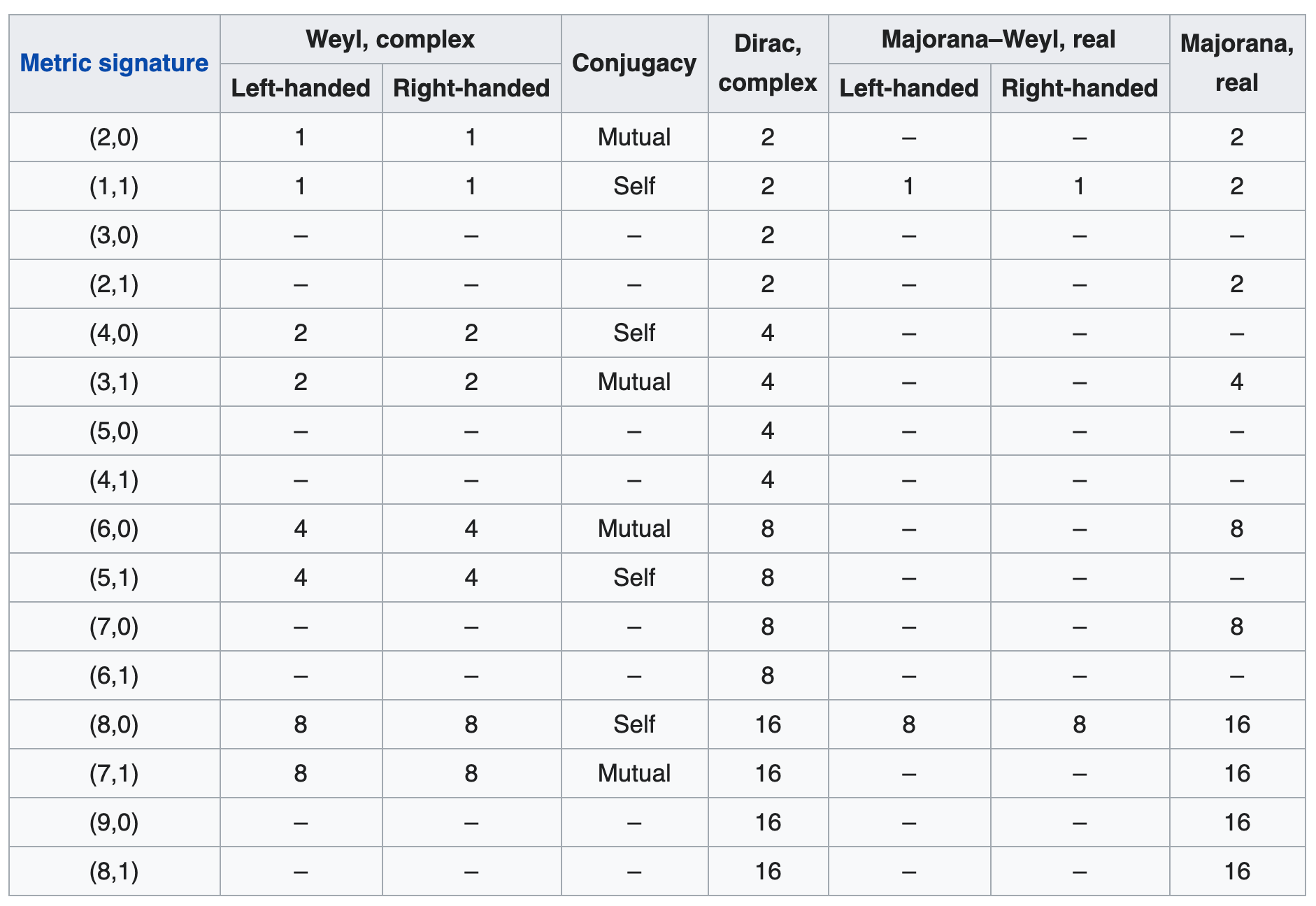
In this wonderful lecture note on Clifford Algebra and Spin(N) Representations, http://hitoshi.berkeley.edu/230A/clifford.pdf

Somehow I find some inconsistency with his Tables of Euclidean and Minkowski fermions, compared with the Table of Wikipedia. (see the bottom https://en.wikipedia.org/wiki/Spinor#Summary_in_low_dimensions)

Wikipedia page shows that for Euclidean fermions, even 6d (6,0) and 7d (7,0) have Majorana fermions. But his Table says NO Majorana in both 6d (6,0) and 7d (7,0).

Wikipedia page shows that for Minkowski fermions, even 8d (7,1) and 1d (0,1) or 9d (8,1) have Majorana fermions. But his Table says NO Majorana in both 8k and 8k+1 d.

Could any expert clarify why there is a discrepancy above? In summary, in Wikipedia, out of mod 8 dimensions, Wikipedia shows that 5 out of 8 cases have Majorana fermions, in both Euclidean and Minkowski signatures. This lecture note on Clifford Algebra and Spin(N) Representations only said that 3 out of 8 cases have Majorana fermions, in both Euclidean and Minkowski signatures.

Here I marked the red colors for the inconsistency data of this lecture note compared with Wikipedia Table.

See Wikipedia page :

Answer 1

It could be that the first reference uses a different terminology. There clearly are Majorana spinors for Spin(6)=SU(4). There are two ways of seeing this. One way is to note that there exists an anti-linear map (commuting with all transformations from Spin(6)), sending the two types of semi-spinors $S_+, S_-$ into each other and squaring to the identity. So, it is meaningful to impose the condition that a Dirac spinor $S=S_+ \oplus S_-$ is an eigenvector of this map with eigenvalue one. Or, in other words, there exists a reality condition that can be imposed on the Dirac spinors of Spin(6). Such real Dirac spinors are called Majorana. This is precisely analogous to what happens for the Lorentz group Spin(1,3).

Another way to see the Majorana spinors is to use Spin(6)=SU(4). A semi-spinor of Spin(6) is then the fundamental representation ${\mathbb C}^4$ of SU(4). One can take the complex conjugate representation of SU(4), which will transform as the other semi-spinor of Spin(6). So, there is a real Dirac spinor which is made up from a spinor of SU(4) and its complex conjugate spinor. Such a real Dirac spinor is parametrised by a single vector in ${\mathbb C}^4$ or by 8 real numbers.