1
$\begingroup$

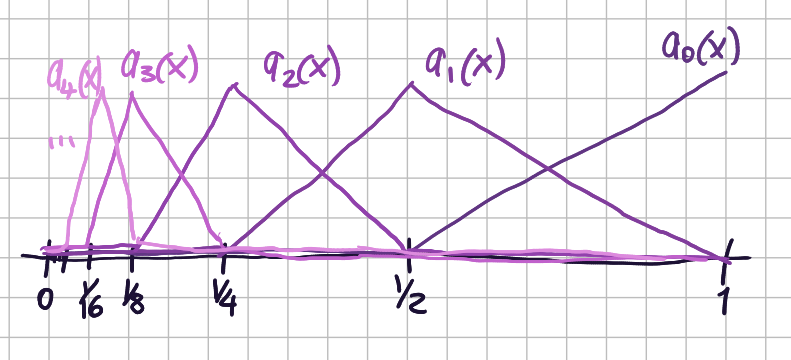
Let $a_1, a_2, \ldots : D\rightarrow\mathbb{R}$ be a sequence of continuous functions, with $D$ a compact metric space.

Suppose that the function $f : D\times[0,\infty)\rightarrow\mathbb{R}$ given by

$$\tag{1}f(x,y) := \sum_{i=1}^\infty a_i(x)\cdot y^i$$

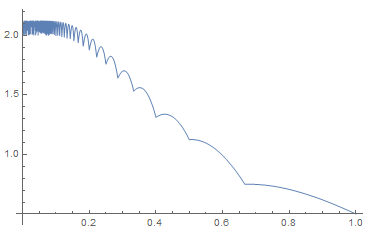
exists and is such that its $y$-section $f_{y_0}:=f(\,\cdot,y_0)$ is bounded on $D$ for some $y_0>0$.

Question: Can we conclude that the sections $f_{y}$ are bounded on $D$ for any $y\geq y_0$?

If necessary, it may be assumed that the $a_i$ are all non-negative.

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ If you look at the one-dimensional case (i.e., where $D$ is a point), then you have a power series which converges on the positive real axis and so on the whole complex plane (to an entire function). You wouldn't expect to extend any boundedness conditions at a given point to ones with larger absolute value. However, you can use the Cauchy integral formula when you reduce it. This applies in modified form to your case--you now have an entire function with values in $C(D)$ and Banach space valued function theory has been well established since the middle of the last century. $\endgroup$
    – bathalf15320
    Commented May 16, 2021 at 14:46

2 Answers 2

Reset to default
2
$\begingroup$

The answer is no. Indeed, let $D:=[0,1]$ and \begin{equation} a_n(x):=nx\,1(x\le1/n)+(2-nx)1(1/n<x<2/n) \end{equation} for natural $n$ and $x\in D$.

Then $a_n$ is continuous for each $n$, the sum \begin{equation} f(x,y)=\sum_{n=1}^\infty a_n(x)y^n \end{equation} has only finitely many nonzero summands for each $(x,y)\in D\times[0,\infty)$ and hence takes real values.
Also, $0\le a_n\le1$ and hence \begin{equation} 0\le f(x,y)=\sum_{n=1}^\infty a_n(x)y^i\le1 \end{equation} for $y=1/2$. So, $f(\cdot,1/2)$ is bounded.

However, for any natural $k$ and $x=1/k$, \begin{equation} f(x,2)\ge\sum_{n=1}^\infty nx\,1(x\le1/n)2^n\ge k(1/k)2^k\to\infty \end{equation} as $k\to\infty$. So, $f(\cdot,2)$ is unbounded.

Here is the graph $\Big\{\Big(x,\dfrac{f(x,2)}{2^{2/x}x}\Big)\colon10^{-3}\le x\le1\Big\}$:

enter image description here

Improve this answer
$\endgroup$
0
1
$\begingroup$

Take $D=[0,2]$, $a_i(x)=x^i$, then $f(x,1/3)$ converges for all $x\in D$, but $f(x,1)$ is unbounded as $x\to1$. So, unless I am misreading something in your question, the answer is negative.

EDIT: If we require that the series converges for all $x$ and $y$, the answer is still negative: let $x_i=1/2^i$ and $a_i(x)$ be the regular "hat" functions "centered" at $x_i$-s (see the illustration). Then (1) is $0$ for $x=0$ and a piecewise linear approximation to $x^{\log_{1/2}y}$ for $x>0$. The latter is bounded on $D=[0,1]$ for $y\leq1$ and unbounded for $y>1$.

enter image description here

Improve this answer
$\endgroup$
8
  • $\begingroup$ Thanks for your answer Iiro. Just from your example, it seems that the function $f$ you gave is not defined (divergent) on $[1,2]\times\{1\}\subset D\times\{1\}$, opposing the above requirement of existence? $\endgroup$
    – fsp-b
    Commented May 15, 2021 at 23:11
  • 1
    $\begingroup$ What do you mean by defined then? If you wish to say that "defined" = "the series converges for all $(x,y)\in D\times [0,\infty)$" then your definition of "defined" guarantees your claim for any fixed $y$. Or are you asking if $f(x,y)$ is bounded for all $x$ and $y$, i.e., is globally bounded? $\endgroup$
    – Iiro Ullin
    Commented May 15, 2021 at 23:18
  • $\begingroup$ I think our definitions of "defined" are in line. What I would like to exclude are sections $f_y : D\rightarrow \mathbb{R}$ which, although defined on all of $D$, are unbounded as functions on $D$ (say, exhibit a blow up towards the boundary of $D$). $\endgroup$
    – fsp-b
    Commented May 15, 2021 at 23:26
  • 1
    $\begingroup$ I was thinking along the lines that if you want your series (1) to be convergent for all $x$ and $y$, you must have $a_i(x)$ to be decaying super exponentially for any given $x$ and, as $D$ is compact, uniformly in $x$. But this would imply that the series would always be bounded. $\endgroup$
    – Iiro Ullin
    Commented May 16, 2021 at 0:16
  • 1
    $\begingroup$ Nevermind: I added an example which shows that the answer is still negative even if the series converges for all $(x,y)$. It also shows that my "uniform decay" claim is incorrect :) however... $\endgroup$
    – Iiro Ullin
    Commented May 16, 2021 at 11:39

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .