Does there exist an upper bound on the Fourier coefficients of the reciprocal theta function $\frac {1}{\theta}$?

Question

Define the theta function as $$ \theta(x) = \sum_{n=-\infty}^\infty e^{-\gamma(x+n)^2} $$ where $\gamma>0$. Clearly, $\theta$ is 1-periodic, non-zero and smooth. Therefore, the reciprocal map $x \mapsto \frac{1}{\theta(x)}$ is 1-periodic and smooth as well and can be expanded as a Fourier series: $$ \frac{1}{\theta(x)} = \sum_{n=-\infty}^\infty a_ne^{2\pi i nx}. $$ I'm interested in upper bounding the Fourier coefficients, i.e. I want to find a non-trivial map $f$ (with a fast decay) such that $$ |a_n| \leq f(n). $$ Are there any results in this direction? Thank you!

Answer 1

As @TerryTao stated correctly in the comments, a bound on the Fourier coefficients can be obtained by the saddle point method: The extension of $\frac 1 \theta$ to a meromorphic function on $\mathbb C$ has poles which are bounded away from the real axis. This implies that the Fourier coefficients of $\frac 1 \theta$ decay at least exponentially. In fact, the Fourier coefficients can be derived explicitly from which we can show that the decay is exactly exponentially:

First note, that your map $\theta$ is essentially the Jacobi theta function of third kind. Using Poisson's summation formula we have $$ \theta(t) = \sqrt \frac \pi \gamma \sum_n \left ( e^{-\frac{\pi^2}{\gamma}} \right )^{n^2} e^{2\pi i n t} = \sqrt \frac \pi \gamma \vartheta_3(it, e^{-\frac{\pi^2}{\gamma}}). $$ Hence, for $q = e^{-\frac{\pi^2}{\gamma}}$ and $z=it$ we have $$ \frac 1 {\theta(t)} = \sqrt \frac \gamma \pi \frac 1 {\vartheta_3(z,q)}. $$ In the paper A.J.E.M. Janssen, Some Weyl-Heisenberg frame bound calculations, Indagationes Mathematicae, Volume 7, Issue 2, 1996, Pages 165-183, Janssen obtains on page 178 the Fourier expansion of $\frac 1 {\vartheta_3}$:

$$ \frac 1 {\vartheta_3(z,q)} = \frac 1 C \sum_k (-1)^k a_k e^{2ikz} $$ $$ C = \sum_{n \in \mathbb Z} (-1)^n (2n+1)q^{(n+\frac 1 2)^2} $$ $$ a_k = 2 \sum_{m=0}^\infty (-1)^m q^{(m+\frac 1 2)(2|k|+m+\frac 1 2)} $$ From the value $|k|$ in the exponent in the definition of $a_k$ it follows directly that the $a_k$'s decay exponentially assuming that $|q|<1$. Applying some trivial bounds on $a_k$ gives you the desired map $f$ so that $|a_k| \leq f(k)$.

To derive the above formula, Jenssen refers to an exercise in the book Whittaker, E., & Watson, G. (1927). A Course of Modern Analysis (4th ed., Cambridge Mathematical Library). He essentially solves this exercise which leads to the above expression.

The above formula should agree with the one derived in the answer of @მამუკა ჯიბლაძე.

Answer 2

By no means anything like a complete answer, but what I have is an empirical explicit formula for the coefficients $a_n$ which might be useful.

For $\theta$ itself, $$ \theta(x)=\sqrt{\,\frac\pi\gamma\,}\ \left(1+2q\cos(2\pi x)+2q^4\cos(4\pi x)+2q^9\cos(6\pi x)+...+2q^{n^2}\cos(2\pi nx)+...\right), $$ where $q=e^{-\frac{\pi^2}\gamma}$.

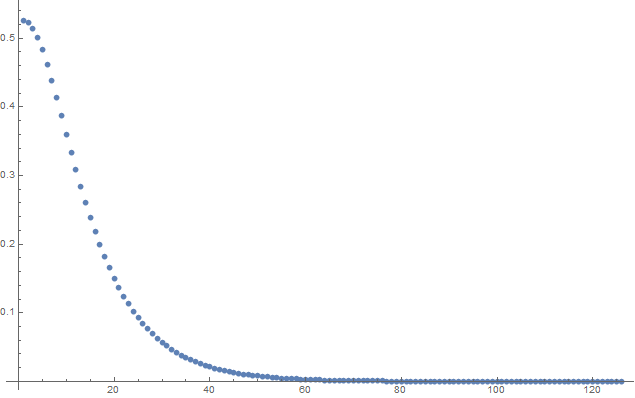
It seems that the following holds (checked in Mathematica to about 100th power of $q$): $$ \frac1{\theta(x)}=\sqrt{\,\frac\gamma\pi\,}\ \frac1{\left((1-q^2)(1-q^4)(1-q^6)\cdots(1-q^{2n})\cdots\right)^3}\ \sum_{n=0}^\infty c_n\cos(2\pi n x), $$ where \begin{align*} c_0=&\ \,1-q^2+q^6-q^{12}+...+(-1)^kq^{k(k+1)}+...,\\[.5ex] c_1=-2&\left(q-q^5+q^{11}-q^{19}+...+(-1)^kq^{k(k+3)+1}+...\right),\\ c_2=2&\left(q^2-q^8+q^{16}-q^{26}+...+(-1)^kq^{k(k+5)+2}+...\right),\\ c_3=-2&\left(q^3-q^{11}+q^{21}-q^{33}+...+(-1)^kq^{k(k+7)+3}+...\right),\\ \cdots\\ c_n=(-1)^n\times2&\sum_{k=0}^\infty(-1)^kq^{k(k+2n+1)+n} \end{align*} Again empirically, these $c_n$ are of order $q^n$, but I do not know how to prove this. Here is the plot of their absolute values for $\gamma=100$: