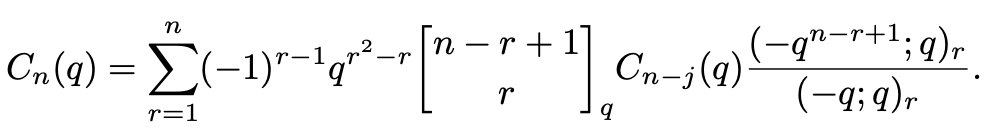I would like to know if the following recurrence relation for Catalan numbers (see mathoverflow.net/questions/191524 and also math.stackexchange.com/questions/2113830) has appeared in a paper or a book, so that I can cite it.
$$C_n = 1 + \sum_{k=1}^{\left\lceil\frac{n}{2} \right\rceil } (-1)^{k+1} \binom{n-k}{k} C_{n-k}$$ where $C_n$ is the $n$-th Catalan number.
Fixed error: added + 1 to the right hand side
T. Koshy, Catalan Numbers with Applications (Oxford, 2009), page 322, proves a very similar identity: $$C_n=\sum_{k=1}^{\left\lfloor\frac{n+1}{2}\right\rfloor}(-1)^{k-1} \binom{n-k+1}{k} C_{n-k}$$ $$\Leftrightarrow \sum_{k=1}^{\left\lfloor\frac{n+1}{2}\right\rfloor} (-1)^{k-1} \binom{n-k+1}{k} C_{n-k}=0.$$ (I did not check the book, the identity is quoted in this paper, equation 4.)
I also note that Mathematica knows the identity in the OP, in the form
$$\sum_{k=0}^{\left\lceil\frac{n}{2} \right\rceil } (-1)^{k+1} \binom{n-k}{k} C_{n-k}=-1,$$ (Floor or ceiling in the summation limits does not matter, the sum may also be extended to infinity, since the binomial coefficients vanish.)
In response to a question in the comment, I record the q-analog of Koshy's identity, derived by George Andrews.
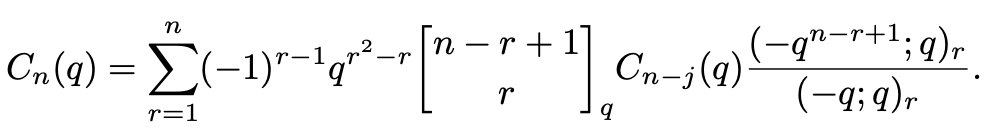
(The sum over $r$ may be terminated at $\left\lfloor\frac{n+1}{2}\right\rfloor$.)
