Prove $\int_{\mathbb R^N \setminus \Omega} |x - z|^{-N-s} dz \approx dist(x,\partial \Omega)^{-s}$, $s \in (0,2)$

Question

Let $\Omega \subset \mathbb R^N$ and $s \in (0,2)$. Under what assumptions on $\partial \Omega$ do we have $$\int_{\mathbb R^N \setminus \Omega} |x - z|^{-N-s} dz \approx \mathrm{dist}(x,\partial \Omega)^{-s} \ ?$$

Answer 1

$\newcommand{\Om}{\Omega}\newcommand{\de}{\delta}\newcommand{\ep}{\varepsilon}\newcommand{\R}{\mathbb R}\newcommand{\pOm}{\partial\Om}$Let $n:=N$. Consider the following "cone" condition:

the boundary $\pOm$ is nonempty and there are $\ep\in(0,1)$ and $\de\in(0,1)$ such that for each $y\in\pOm$ there is a unit vector $v_y$ such that $y+C_{\ep,\de,v_y}\subseteq\Om^c:=\R^n\setminus\Om$, where \begin{equation*} C_{\ep,\de,v_y}:=\{r\in\R^n\colon0<|r|<\ep,|u_r-v_y|<\de\}, \end{equation*} $$u_r:=r/|r|,$$ and $|\cdot|$ is the Euclidean norm on $\R^n$. Informally, this means that a small enough neighborhood of every boundary point of $\Om$ has some substantial enough intersection with the complement of $\Om$.

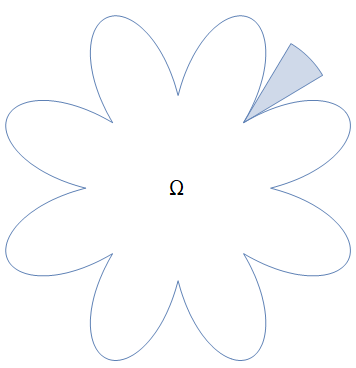
The "cone" condition is illustrated by the following picture, showing a domain $\Om$ and a "cone" (that is, a sector of a disk) $y+C_{\ep,\de,v_y}$ for a point $y\in\pOm$:

Let us show that this "cone" condition is enough for \begin{equation*} I_x:= \int_{\Om^c} dz\,|x-z|^{-n-s}\asymp d_x^{-s} \tag{1} \end{equation*} as $d_x:=\mathrm{dist}(x,\pOm)\to0$, where $x\in\Om$, $\Om^c:=\R^n\setminus\Om$, and $a\asymp b$ means $a=O(b)$ and $b=O(a)$.

Indeed, simple geometric considerations yield the following lemmas.

Lemma 1: For any $x,y,z$ in $\R^n$ and any unit vector $v\in\R^n$ we have the following continuity-type implication: \begin{equation*} |y-x|<\frac\de{2\sqrt2}\,|z-y|\ \&\ |u_{z-x}-v|<\de/2\implies|u_{z-y}-v|<\de. \end{equation*}

Lemma 2: For any $x,y,z$ in $\R^n$ and any real $k>2$ we have the following implication: \begin{equation*} \ep/2>|z-x|>k|y-x|\implies\ep>|z-y|>k|y-x|/2. \end{equation*}

The proofs of these lemmas will be given at the end of this answer.

Let now \begin{equation*} k:=\frac{4\sqrt2}\de, \end{equation*} so that $k>2$. Take any $x\in\R^n$. Then there is a point $y=y_x\in\pOm$ such that $d_x=|y-x|$. By Lemmas 1 and 2 and the "cone" condition, \begin{equation*} Z:=Z_x:=\{z\in\R^n\colon |u_{z-x}-v|<\de/2,\ep/2>|z-x|>kd_x\}\subseteq y+C_{\ep,\de,v_y}\subseteq\Om^c. \end{equation*} So, letting $p$ denote the (nonzero) probability that a random vector $U$ uniformly distributed on the unit sphere $S^{n-1}$ will be at distance $<\de/2$ from a given unit vector, we get \begin{equation*} I_x\ge\int_Z dz\,|x-z|^{-n-s}\asymp p\int_{kd_x}^{\ep/2}d\rho\,\rho^{n-1}\rho^{-n-s} \asymp d_x^{-s} \end{equation*} On the other hand, the condition $x\in\Om$ implies that $d_x=\mathrm{dist}(x,\Om^c)$. So, \begin{equation*} I_x\le\int\limits_{z\in\R^n\colon|x-z|\ge d_x} dz\,|x-z|^{-n-s}\asymp \int_{d_x}^\infty d\rho\,\rho^{n-1}\rho^{-n-s} \asymp d_x^{-s}. \end{equation*} Thus, (1) follows.

---

It remains to prove Lemmas 1 and 2.

Proof of Lemma 1: Replacing $z-x$ and $z-y$ by $x$ and $y$, respectively, we see that, to prove Lemma 1, it suffices to verify the implication \begin{equation*} |y-x|<\frac\de{2\sqrt2}\,|y|\implies|u_y-u_x|<\de/2. \end{equation*} Assuming that indeed $|y-x|<\dfrac\de{2\sqrt2}\,|y|$ and letting $a\in[0,\pi]$ denote the angle between $x$ and $y$, we see that $\sin a\le|y-x|/|y|<\dfrac\de{2\sqrt2}<\dfrac1{\sqrt2}$, so that $a<\pi/4$, and hence $a/2<\pi/4$ and $\cos a/2>1/\sqrt2$. So, $\sin a/2=\dfrac{\sin a}{2\cos a/2}\le\dfrac{\sin a}{\sqrt2}<\de/4$. Thus, $|u_y-u_x|=2\sin a/2<\de/2$, as desired. $\Box$

Proof of Lemma 2: Assume that indeed $\ep/2>|z-x|>k|y-x|$ and $k>2$. Then $|z-y|\ge|z-x|-|y-x|>(k-1)|y-x|\ge k|y-x|/2$ and $|z-y|\le|z-x|+|y-x|<\ep/2+\ep/(2k)<\ep$. $\Box$