12
$\begingroup$

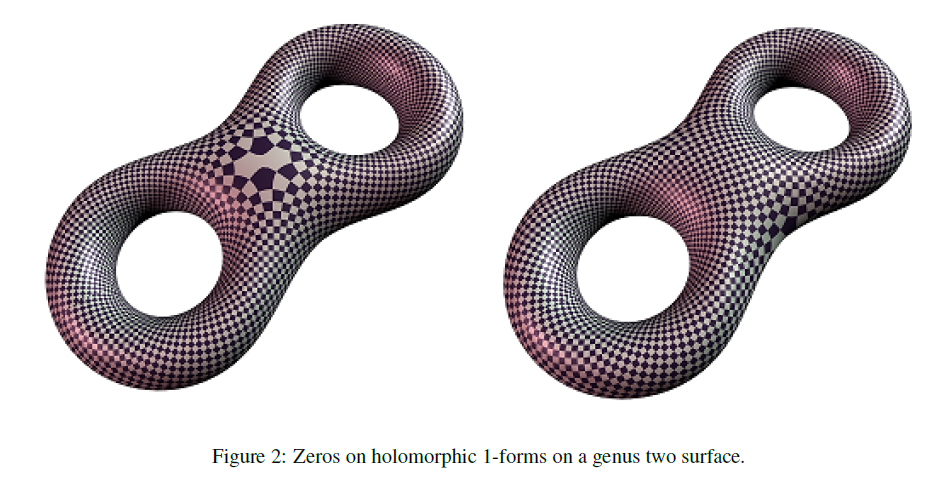
It is a classical result that the vector space of holomorphic differentials on a compact Riemann surface of genus $g$ has dimension $g$. I am wondering if there is a way of visualizing this wonderful result.

I should perhaps say a bit more about what I mean by "visualizing." I found another MO question on visualizing the Riemann–Roch theorem, and what I am asking for here could be viewed as the simplest case of Riemann–Roch. There are some interesting answers to that question, but I am hoping for something more literally visual, along the lines of Dan Piponi's note On the visualisation of differential forms and/or the paper On the geometry, flows and visualization of singular complex analytic vector fields on Riemann surfaces by Alvarez-Parrilla et al. The ultimate dream would be some kind of animation that allows one to get some intuitive feeling for what holomorphic (or harmonic, if that is easier) differentials are constrained to be like, and why adding an extra hole adds an extra degree of freedom.

Improve this question
$\endgroup$
1

2 Answers 2

Reset to default
9
$\begingroup$

This is not really an answer, it is a comment with images. The paper "Quadrilateral Mesh Generation II : Meromorphic Quartic Differentials and Abel-Jacobi Condition" (arXiv 2019) contains (imo) nice exposition of theoretical background and several illuminating illustrations.

enter image description here

enter image description here

enter image description here

Improve this answer
$\endgroup$
6
$\begingroup$

There is a very nice hydrodynamic interpretation, going back to Riemann and Klein, and popularized by Courant (Hurwitz and Courant, Function theory). For those who do not read German, there is a modern exposition in French: E. Ghys and D. Smai, Six lecons autour des surfaces de Riemann

Edit. Klein's book is "On Riemann's theory of algebraic functions", the only one of the three which has been translated into English.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Fluid and air streams, heat distribution, electric potential were investigated via complex analysis by Riemann, Klein, and others--Klein, in his book I note in the other MO-Q on RR. Do you know of additional books that present Riemann sufaces in these ways? Does Springer in Intro to Riemann Surfaces do so? I've seen other older books that have done so (and once had such a book that I left in Japan), but I can't recall the authors. $\endgroup$
    – Tom Copeland
    Commented Apr 21, 2021 at 23:08
  • $\begingroup$ In my answer, I mention the two books known to me that do this. (In addition to the classical book by Klein). Of these, only Klein has been translated to English, I believe. $\endgroup$
    – Alexandre Eremenko
    Commented Apr 22, 2021 at 0:06
  • $\begingroup$ See also mathoverflow.net/questions/19649/… and Conformal Mapping on Riemann Surfaces by Harvey Cohn. $\endgroup$
    – Tom Copeland
    Commented Apr 22, 2021 at 4:04
  • $\begingroup$ Also, Visual Complex Analysis by Needham. $\endgroup$
    – Tom Copeland
    Commented Apr 22, 2021 at 20:07
  • $\begingroup$ @Tom Copeland: Needham book does not cover Riemann surfaces. And in general I do not recommend Needham's books to mathematicians to study mathematics, though they can be somewhat entertaining. $\endgroup$
    – Alexandre Eremenko
    Commented Apr 22, 2021 at 21:00

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .