Objects in bijection with integer partitions (and lattices)

Question

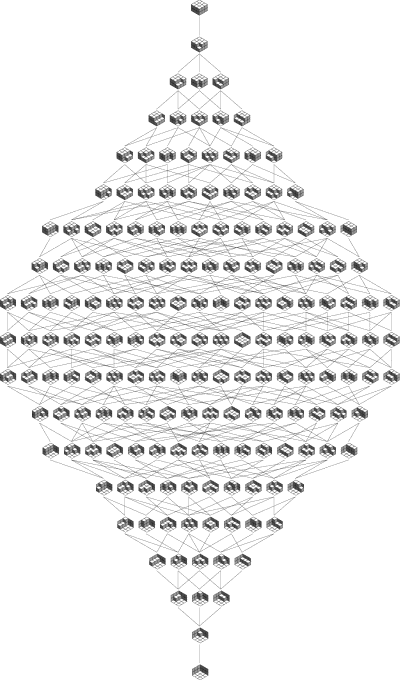
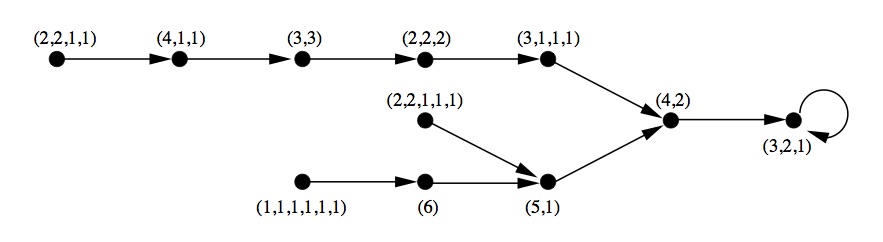
A partition of $n$ is a non-increasing sequence of positive integers of sum $n$. Several lattices are defined over integer partitions, in particular the dominance order and the Young lattice.

Several combinatorial objects are in bijection with integer partitions and their lattice structures. For instance, Dyck words have the Young lattice structure, and the linear Sand Pile Model and its Brylawski extension have the dominance order lattice structure.

Going further, one may define plane integer partitions, and generalized integer partitions. A generalized partition of $n$ on a given directed acyclic graph is a positive weight for each vertex such that the weight sum is $n$ and weights are non-increasing when we follow edges. Such partitions also have a Young lattice structure.

Plane partitions and Young lattice are in bijection with rhombus tilings of hexagons with flips (figure below, better resolution here), and generalized partitions are in bijection with rhombus tilings of 2D-gons.

                 

I am interested in finding more combinatorial objects in bijection with (meaningful subsets of) (various kinds of) integer partitions, in particular (but not necessarily) if they preserve a lattice structure.

Answer 1

The states of the recreational mathematics topic Bulgarian Solitaire are integer partitions. Once you get past the story about shifting piles of cards or books or coins, the operation takes the partition $(\lambda_1,\ldots,\lambda_t)$ to $(t, \lambda_1-1,\ldots,\lambda_t-1)$ where any zeros are removed and the parts are put in nonincreasing order. Here is the state diagram for the partitions of 6 (with a typo: the 'middle' partition should be (2,1,1,1,1) not (2,2,1,1,1)).

One of the first results on this topic was that there is a fixed point/1-cycle like (3,2,1) exactly when $n$ is a triangular number. For other values of $n$, there are longer cycles and usually multiple components, which complicates lattice analysis. Characterizations of the cycle partitions and "Garden of Eden" states (partitions with no predecessors under the operation; (2,2,1,1), (2,1,1,1,1), and (1,1,1,1,1,1) in the example) have been worked out.

There are many variations of Bulgarian Solitaire, varying both the operation and even the underlying object (compositions, plane partitions, and restricted pairs of partitions in "Austrian Solitaire"). To get into the literature (and understand of the odd name), see a survey article I wrote for one of the Martin Gardner tributes in The College Mathematics Journal called 30 Years of Bulgarian Solitaire (JSTOR link).