Zhu's algebra for the Virasoro VOA

Question

I am trying to understand the proof in the appendix of the following paper: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.110.8757&rep=rep1&type=pdf

The paper discusses Zhu's algebra for the Virasoro VOA $M_c$ and showed that $A(M_c)\simeq \mathbb{C}[x]$. The isomorphism is proved using the claim that $O(M_c)$ is spanned by elements of the form

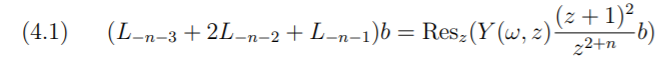
A proof is provided in the appendix.

What I am confused on is the last step:

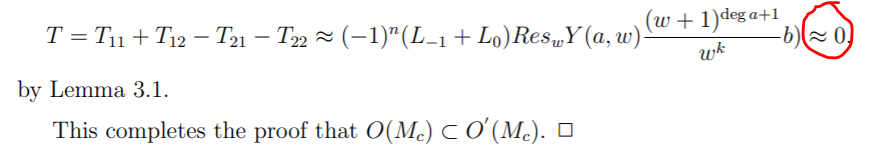
It seems that we have to use $(L_{-1}+L_0)b$ is a linear combination of elements of the form (4.1) for every $b\in M_c$. I am having some troubles to see this. Any help will be appreicated.

Update: It says by Lemma 3.1. But Lemma 3.1 is proved by $$Res_z Y(a,z)\textbf{1} \frac{(1+z)^{\deg a}}{z^2} \in O(M_c).$$ I am not sure how it fits in the induction process.

Answer 1

Since the question got at least one upvote, perhaps I should post my own answer instead of deleting the question.

It can be proved by induction that $(L_0 + L_{-1})b \in O'(M_c)$, i.e., $(L_0+L_{-1})b$ is a linear combination of elements of the form (4.1). Here is the sketch:

1. Show that $(L_{-2}+L_{-1}) O'(M_c)\subset O'(M_c)$.

2. Show that for every $n\geq 2$ and every $b\in M_c$ $$L_{-n} b \equiv (-1)^n((n-1)L_{-2} + (n-2)L_{-1}) b \mod O'(M_c). $$

3. Assume the conclusion holds for every $b=L_{-n_1}\cdots L_{-n_k}\textbf{1}$ with $k<s$.

   3.1. Show that $(L_0+L_{-1})L_{-1}L_{-n_2}\cdots L_{-n_s}\textbf{1}\in O'(M_c)$, using $$L_{-1} L_{-n_2}\cdots L_{-n_s} \textbf{1} = \sum_{i=2}^s (n_i-1) L_{-n_2} \cdots L_{-n_i-1} \cdots L_{-n_s}.$$

   3.2. Compute as follows \begin{align*} & (L_0+L_{-1})L_{-n_1} L_{-n_2}\cdots L_{-n_s}\textbf{1} \\ =& L_{-n_1} (L_0+L_{-1}) L_{-n_2}\cdots L_{-n_s}\textbf{1} \\ & + ((n_1-1)L_{-n_1-1}+ n_1 L_{-n_1}) L_{-n_2}\cdots L_{-n_s}\textbf{1} \end{align*} By 1 and 2 and the induction hypothesis, the first term is equivalent to $$(-1)^{n_1+1}L_{-1} (L_0+L_{-1})L_{-n_2}\cdots L_{-n_s}\textbf{1}$$ By 1 (or by a straghtforward induction), the second term is equivalent to $$(-1)^{n_1+1}L_{-1} L_{-n_2}\cdots L_{-n_s}\textbf{1}$$ The sum of these guys are precisely $$(-1)^{n_1+1}(L_0+L_{-1}) L_{-1} L_{-n_2}\cdots L_{-n_s} \textbf{1}$$ which is in $O'(M_c)$ by 3.1.