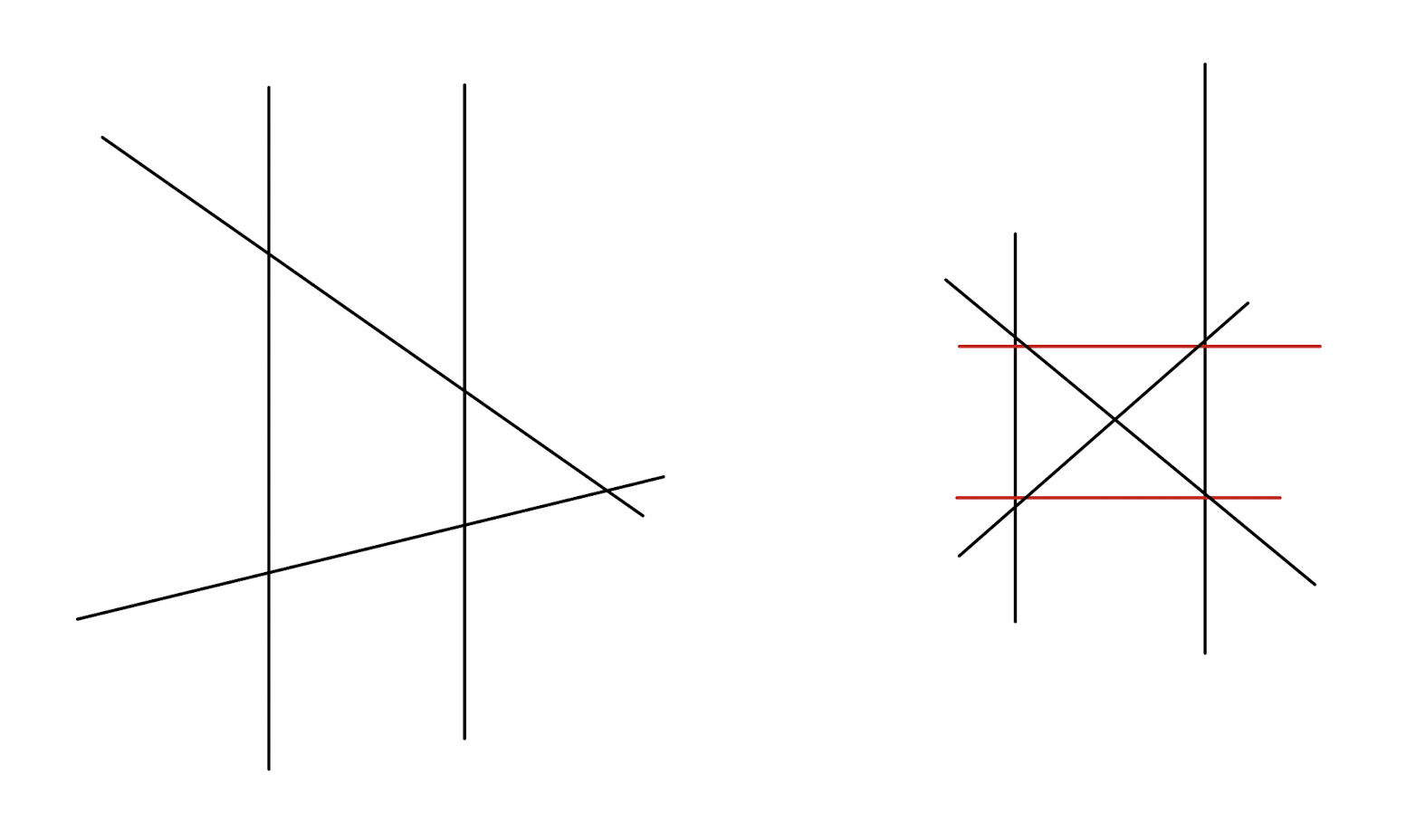1
$\begingroup$

Let $A_1,A_2\subset \mathbb{C}^n$ be hyperplane arrangements with equivalent intersection lattices $L(A_1)\cong L(A_2)$. If $A_1\subset B_1$, where $B_1$ is third hyperplane arrangement, does there always a hyperplane arrangement $B_2$ with $A_2\subset B_2$ such that $L(B_1)\cong L(B_2)$?

I guess the answer is no, but I have not been able to come up with a counter-example.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

I'm pretty sure you can do this with line arrangements: enter image description here The two black line arrangements are equivalent, but in the picture on the right if we add two parallel lines that intersect only at triple intersections, there's not going to be a way to do similarly on the left.

These are real pictures but you can complexify.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ I'm not well-versed in hyperplane arrangements, so forgive me if I'm being silly, but as affine arrangements these don't have intersection lattices at all, right? Since the answer was accepted I assume it's just an abuse of terminology and this is what was meant, but one can certainly get a projectively equivalent arrangement by having the red lines cross in the middle. $\endgroup$
    – lambda
    Commented Mar 19, 2021 at 15:28
  • 1
    $\begingroup$ @lambda: ah, apologies, you are correct. It is very common to consider affine arrangements and their meet semilattices which is what I was implicitly doing. But there's no big difference between central and affine arrangements because you can always obtain central arrangement in one dimension higher by taking the cone over the hyperplanes in an affine arrangement. So I believe this should still answer the question. $\endgroup$
    – Sam Hopkins
    Commented Mar 19, 2021 at 15:55
  • $\begingroup$ As far as the intersection lattice is concerned, I think taking that cone is the same as considering it projectively. (Either way it's the lattice of flats of the corresponding matroid, right?) So this arrangement would become equivalent to the one on the right. $\endgroup$
    – lambda
    Commented Mar 19, 2021 at 16:30
  • 1
    $\begingroup$ @lambda: no, having two pairs of parallel lines will be very different from having one. For an explanation of coning, see e.g. Stanley's notes: cis.upenn.edu/~cis610/sp06stanley.pdf. $\endgroup$
    – Sam Hopkins
    Commented Mar 19, 2021 at 16:35
  • $\begingroup$ Ah, I see, I had forgotten that the cone construction also involves adding an additional hyperplane, so you get (different) single-element extensions of the matroid I was thinking of. $\endgroup$
    – lambda
    Commented Mar 19, 2021 at 18:53

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .