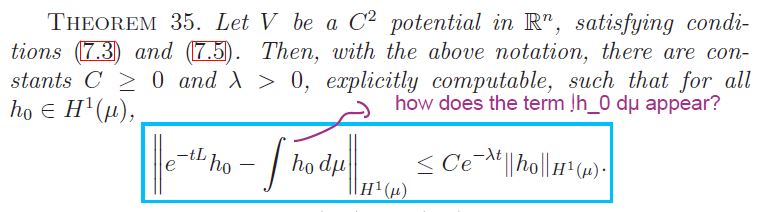1
$\begingroup$

enter image description here

enter image description here

I can not figure out the appearance of the term $\int h_0\,d\mu$ in the statement of Theorem 35 above. Here are some background information: $L$ is an unbunded operator on a Hilbert space $\mathcal{H}^1$, whose kernel is $\mathcal{K}$, the notation $\mathcal{H}^1/\mathcal{K}$ represents $\mathcal{K}^\perp$, $\mu$ is some reference measure of the form $\mu(dx\,dv) = f_\infty(x,v)dx\,dv$, where $f_\infty(x,v)$ is a probability density in both $x$ and $v$ variables. My question is: since Theorem 35 is a direct application (ignoring technical details), shouldn't the conclusion (from Theorem 18) $\|\mathrm{e}^{-tL}\|_{H^1(\mu)/\mathcal{K} \to H^1(\mu)/\mathcal{K} } \leq C\,\mathrm{e}^{-\lambda\,t}$ translates to $$\|\mathrm{e}^{-tL}\,h_0\|_{H^1(\mu)} \leq C\,\mathrm{e}^{-\lambda\,t}\|h_0\|_{H^1(\mu)}?$$ Why the term $\int h_0\,d\mu$ suddenly appears out of the blue? Thank you very much!

Improve this question
$\endgroup$
8
  • $\begingroup$ You need to handle the kernel though. I don't know his notation, but is it the case that (a) constant functions are in $H^1(\mu)$ and (b) the constant functions comprise the entirety of the kernel of $L$? $\endgroup$
    – Willie Wong
    Commented Mar 18, 2021 at 1:33
  • $\begingroup$ @WillieWong Yes, in the particular case under Theorem 35's consideration, $\ker(L)$ contains only constant functions. Also, constant functions are in $H^1(\mu)$ (as $\mu$ is a probability measure in the space $x$ and velocity $v$ variables) $\endgroup$
    – Fei Cao
    Commented Mar 18, 2021 at 1:41
  • $\begingroup$ Then in this case the projection of $h_0$ to $H^1/K$ is exactly $h_0 - \int h_0 ~d\mu$, and $e^{-tL}$ acts trivially on the latter factor. $\endgroup$
    – Willie Wong
    Commented Mar 18, 2021 at 13:28
  • $\begingroup$ @WillieWong I understand that $e^{-tL}\left(h_0 - \int h_0 \,d\mu\right) = e^{-tL}\,h_0 - \int h_0 \,d\mu$, but why do you say that "the projection of $h_0$ to $\mathcal{K}^\perp$ is exactly $h_0 - \int h_0 \,d\mu$"? $\endgroup$
    – Fei Cao
    Commented Mar 18, 2021 at 17:27
  • $\begingroup$ @WillieWong Continuing on my previous comment, why shouldn't the conclusion be $\|\mathrm{e}^{-tL}\,h_0 - \int h_0\, d\mu\|_{H^1(\mu)} \leq C\,\mathrm{e}^{-\lambda\,t}\|h_0 - \int h_0 \, d\mu\|_{H^1(\mu)}$ $\endgroup$
    – Fei Cao
    Commented Mar 18, 2021 at 17:30

0

Reset to default

You must log in to answer this question.