$\ell_\infty$-norm covering number of RKHS ball $\{f\in\mathcal{H}: \|f\|_\mathcal{H} \leq R\}$

Question

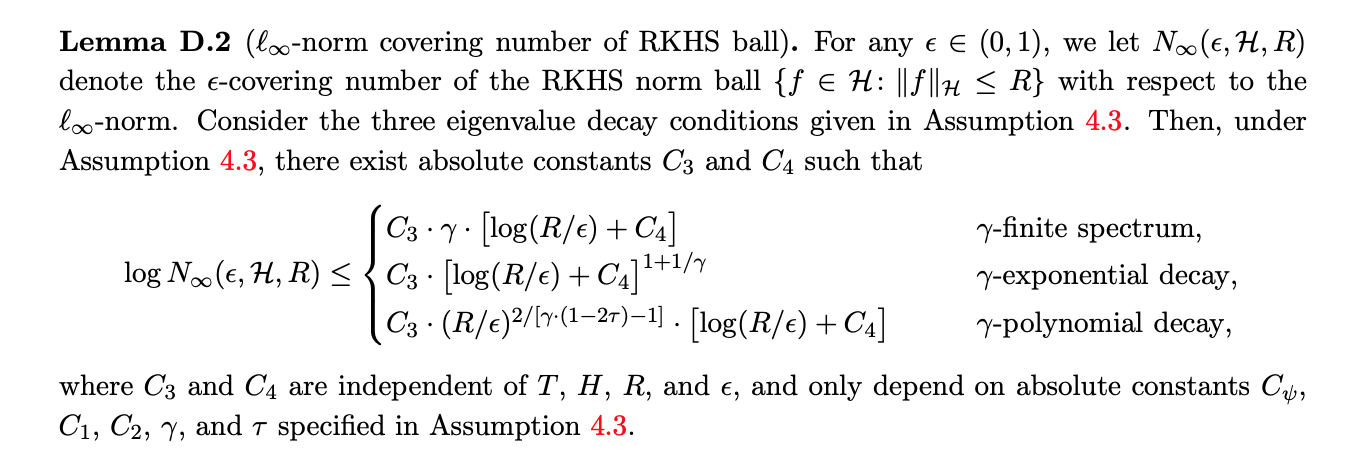
For any $\epsilon \in (0,1)$, let $N_\infty(\epsilon, \mathcal{H}, R)$ denote the $\epsilon$-covering number of the RKHS norm ball $\{f\in\mathcal{H}: \|f\|_\mathcal{H} \leq R\}$ with respect to the $\ell_\infty$-norm. What are some reference where I can find upperbound for $\log {N_\infty(\epsilon, \mathcal{H}, R)}$?

I am currently reading this paper (link) and in Lemma D.2 in page 59, they give bounds under three different conditions for eigenvalue decay of $\mathcal{H}$. But the assumption seems quite restrictive and I would like the avoid this assumption.

Here is Assumption 4.3

Here is Equation (2.3) from the paper.