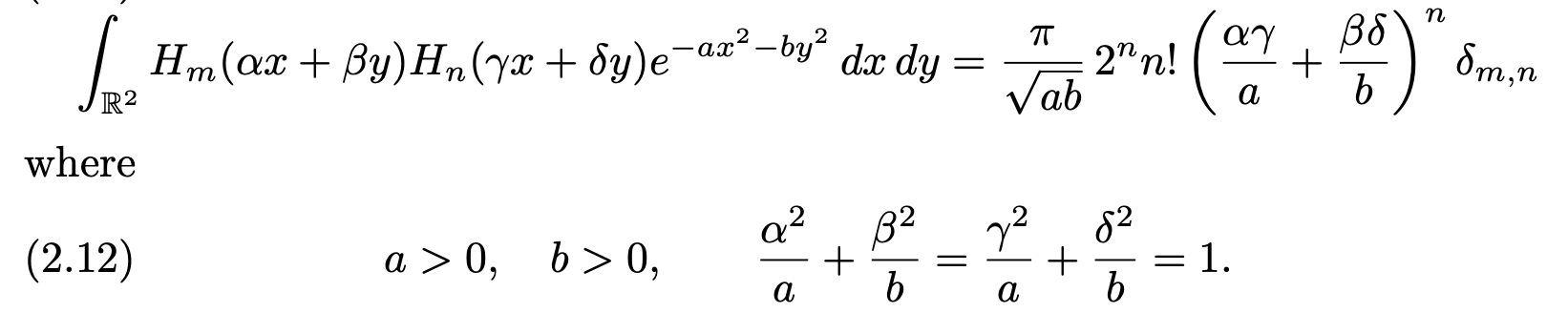3
$\begingroup$

Consider the "probabilist's" Hermite polynomials given by $$H_n(x)=(-1)^ne^{\frac{x^2}{2}}\partial_x^ne^{-\frac{x^2}{2}}.$$

These polynomials trivially extend to functions of $w\in\mathbb{C}$. While working with them, I noticed the following orthogonality identity:

$$\iint_{\mathbb{C}}H_k(w)H_n(i\overline{w})e^{-\frac{1}{2}w^2-\mathrm{Im}(w)^2}\,dx\,d\xi=0$$ where $w=x+i\xi$ and $k\neq n$.

I have a proof but it is very roundabout (using the FBI transform). Is there a simple direct proof of the identity somewhere, or else a transformation to a more well-known orthogonality identity (with reference)?

Improve this question
$\endgroup$
2
  • $\begingroup$ just to be sure, in the exponent it says ${\rm Im}(w)^2$, is that the imaginary part of $w^2$ or the square of the imaginary part of $w$? $\endgroup$
    – Carlo Beenakker
    Commented Feb 15, 2021 at 21:25
  • $\begingroup$ The square of the imaginary part. $\endgroup$
    – Yonah Borns-Weil
    Commented Feb 15, 2021 at 21:44

2 Answers 2

Reset to default
5
+50
$\begingroup$

Fix $\xi$ and assume $k>n$ (the other case is similar). Integrate over $x$. You'll get $\int_{\mathbb R+i\xi}H_k(z)P_n(z-i\xi,\xi)e^{-z^2/2-\xi^2}\,dz$ where $P_n$ is some polynomial of 2 variables of degree $n$ with complex coefficients, so for fixed $\xi$, it can be written as a polynomial of degree $\le n$ in $z$. Now just shift the contour back to the real line and use the fact that $H_k$ is orthogonal to every polynomial of degree $\le n<k$ with the weight $e^{-x^2/2}$. That's it.

Improve this answer
$\endgroup$
0
$\begingroup$

In Complex Hermite polynomials: their combinatorics and integral operators the following general orthogonality relation is proven:

Here the Hermite polynomials are defined with factors $e^{\pm x^2}$ instead of $e^{\pm x^2/2}$.

Not quite the relation in the OP, yet....

Improve this answer
$\endgroup$

You must log in to answer this question.